Display Title
Definition--Quadratics Concepts--The Discriminant
Display Title
The Discriminant
Topic
Quadratics Concepts
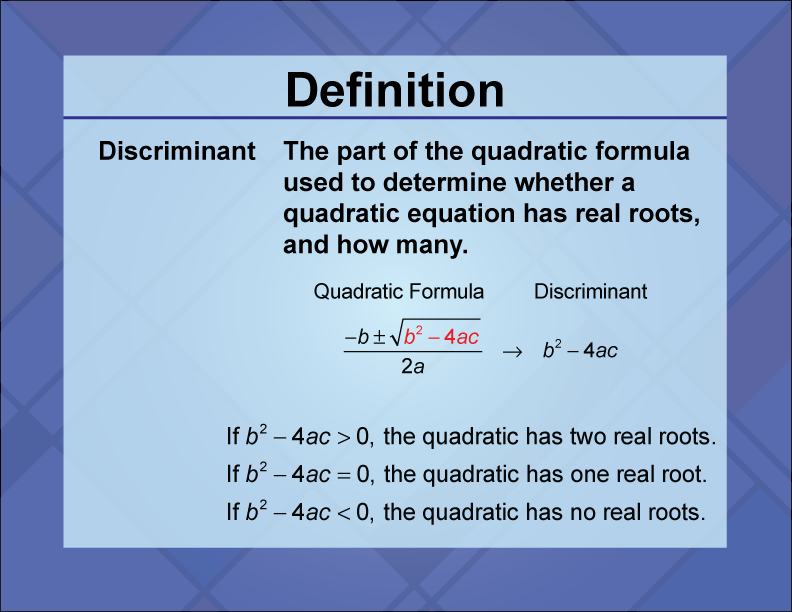
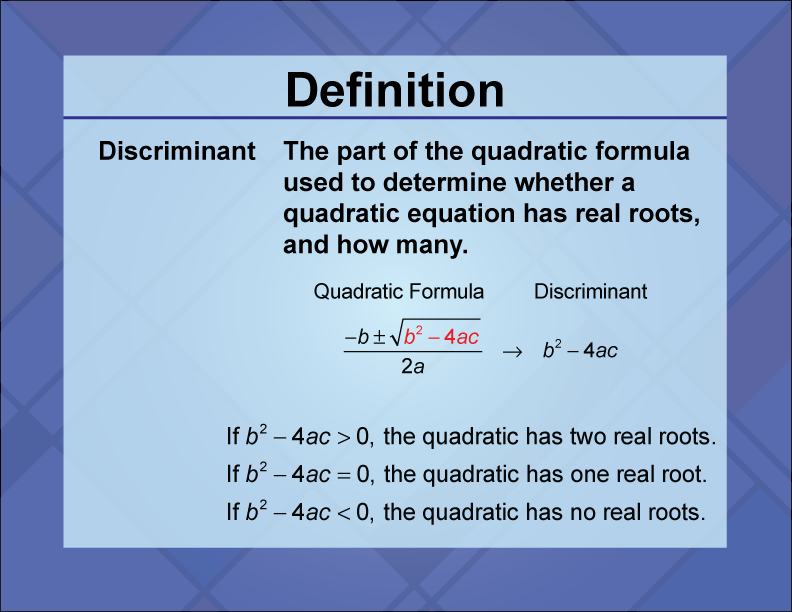
Definition
The discriminant is a component of the quadratic formula that determines the nature of the roots of a quadratic equation.
Description
The discriminant, given by
b2 − 4ac
is a key element in the quadratic formula that indicates whether a quadratic equation has two real roots, one real root, or two complex roots. This value provides insight into the nature of the solutions without solving the equation completely. In real-world applications, the discriminant is used to assess the feasibility of solutions in engineering and physics. In math education, understanding the discriminant helps students predict the number and type of solutions of quadratic equations, enhancing their problem-solving skills and comprehension of algebraic concepts. By analyzing the discriminant, students learn to determine the nature of the roots and relate them to the graph of the quadratic function.
An example of using the discriminant is in the equation
x2 + 4x + 4 = 0
where the discriminant is zero, indicating a repeated real root.
For a complete collection of terms related to Quadratic Expressions, Functions, and Equations click on this link: Quadratics Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSN.CN.C.7, CCSS.MATH.CONTENT.HSA.SSE.B.3.B, CCSS.MATH.CONTENT.HSA.REI.B.4.A, CCSS.MATH.CONTENT.HSF.IF.C.8.A |
|---|---|
| Grade Range | 6 - 10 |
| Curriculum Nodes |
Algebra • Quadratic Functions and Equations • Quadratic Equations and Functions |
| Copyright Year | 2013 |
| Keywords | quadratic functions, quadratic equations, quadratic formula, definitions, glossary terms |