Display Title
Definition--Quadratics Concepts--Quadratic Function in Factored Form
Display Title
Quadratic Function in Factored Form

Topic
Quadratics Concepts
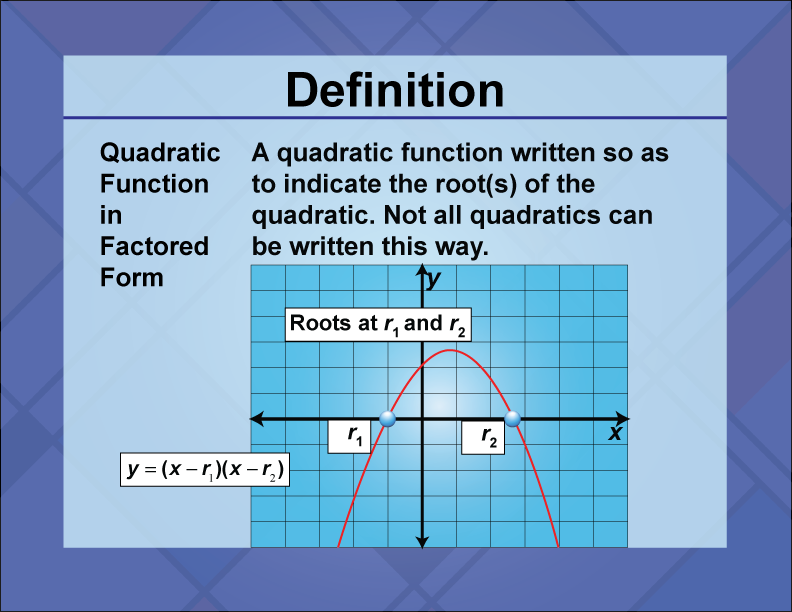
Definition
A quadratic function in factored form is expressed as
f(x) = a(x − r1)(x − r2)
where r1 and r2 are the roots.
Description
The factored form of a quadratic function is particularly useful for identifying the roots or x-intercepts of the function directly. This form is advantageous in solving quadratic equations and graphing parabolas, as it clearly shows where the graph intersects the x-axis. In real-world applications, the factored form is used in scenarios where determining the points of zero output is essential, such as in physics for finding equilibrium points or in business for break-even analysis. In math education, understanding the factored form helps students grasp the concept of roots and how they relate to the graph of a quadratic function. It also aids in teaching factoring techniques and the relationship between different forms of quadratic equations.
An example of a quadratic function in factored form is
f(x) = (x − 1)(x − 3)
which has roots at x = 1 and x = 3.
For a complete collection of terms related to Quadratic Expressions, Functions, and Equations click on this link: Quadratics Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSN.CN.C.7, CCSS.MATH.CONTENT.HSA.SSE.B.3.B, CCSS.MATH.CONTENT.HSA.REI.B.4.A, CCSS.MATH.CONTENT.HSF.IF.C.8.A |
|---|---|
| Grade Range | 6 - 10 |
| Curriculum Nodes |
Algebra • Quadratic Functions and Equations • Quadratic Equations and Functions |
| Copyright Year | 2021 |
| Keywords | quadratic functions, quadratic equations, quadratic formula, definitions, glossary terms |