Display Title
Definition--Quadratics Concepts--Quadratic Function in Vertex Form
Display Title
Quadratic Function in Vertex Form
Topic
Quadratics Concepts
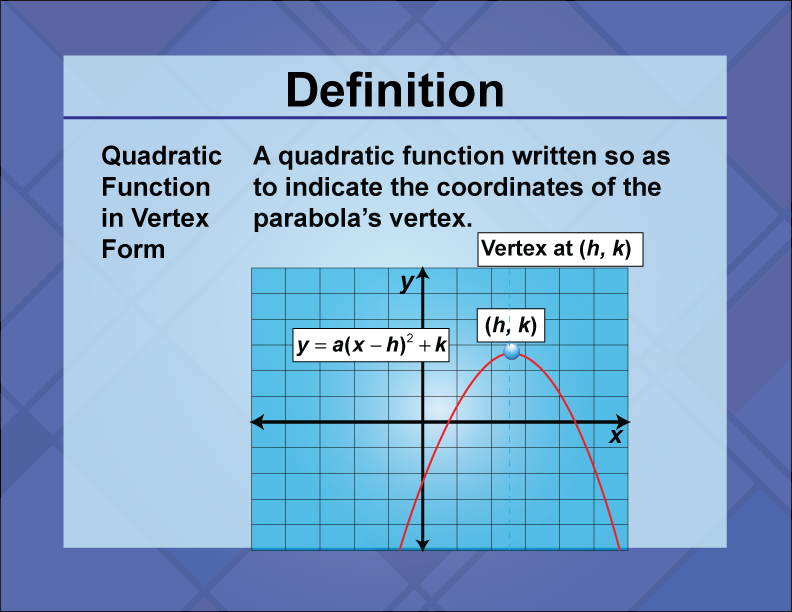
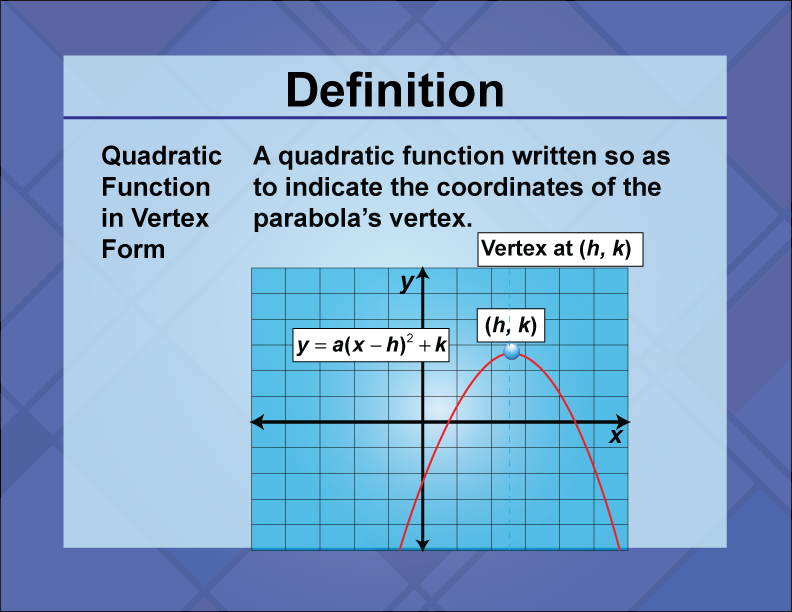
Definition
A quadratic function in vertex form is expressed as
f(x) = a(x − h)2 + k
where (h, k) is the vertex of the parabola.
Description
The vertex form of a quadratic function highlights the vertex, making it easy to identify the maximum or minimum point of the parabola. This form is particularly useful for graphing and transforming quadratic functions, as it directly provides the vertex coordinates. In real-world applications, vertex form is used in optimization problems where finding the highest or lowest point is crucial, such as in maximizing profit or minimizing cost. In math education, understanding the vertex form helps students learn how to complete the square, convert between different forms of quadratic functions, and analyze the impact of transformations on the graph.
An example of a quadratic function in vertex form is
f(x) = 3(x − 2)2 + 5
which has a vertex at (2, 5).
For a complete collection of terms related to Quadratic Expressions, Functions, and Equations click on this link: Quadratics Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSN.CN.C.7, CCSS.MATH.CONTENT.HSA.SSE.B.3.B, CCSS.MATH.CONTENT.HSA.REI.B.4.A, CCSS.MATH.CONTENT.HSF.IF.C.8.A |
|---|---|
| Grade Range | 6 - 10 |
| Curriculum Nodes |
Algebra • Quadratic Functions and Equations • Quadratic Equations and Functions |
| Copyright Year | 2013 |
| Keywords | quadratic functions, quadratic equations, quadratic formula, definitions, glossary terms |