Display Title
Definition--Rationals and Radicals--Laws of Rational Exponents
Display Title
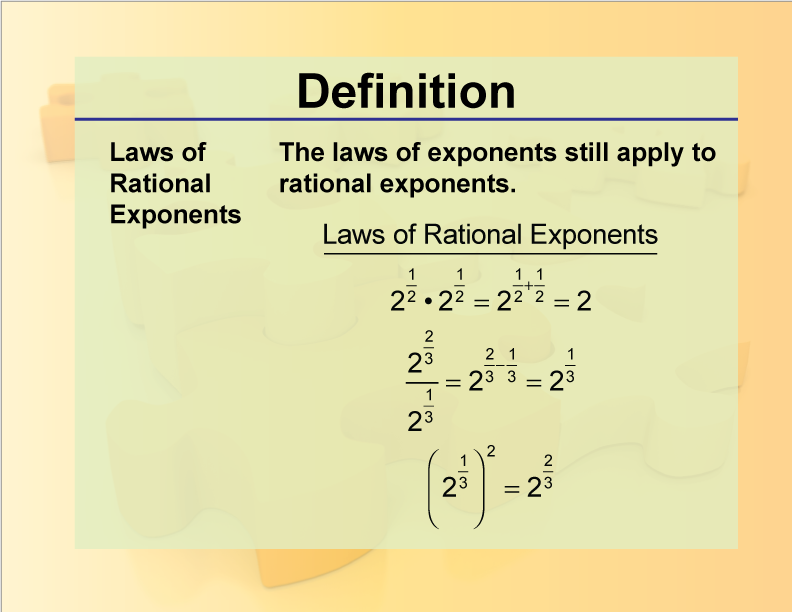
Laws of Rational Exponents

Topic
Rationals and Radicals
Definition
The laws of rational exponents describe how to handle exponents that are fractions, including rules for multiplication, division, and raising a power to a power.
Description
The Laws of Rational Exponents are vital in the study of Rational Numbers, Expressions, Equations, and Functions. These laws provide a framework for simplifying expressions involving exponents that are fractions. For example, the law
$$a^{m/n} = \sqrt[n]{a^m}$$
allows us to rewrite expressions in a more manageable form. Understanding these laws is crucial for solving equations involving rational exponents and for manipulating algebraic expressions. They are widely used in various fields, such as engineering, physics, and computer science, where exponential relationships are common. Mastery of these laws enables the simplification of complex expressions and the solving of equations that arise in real-world applications.
For a complete collection of terms related to polynomials click on this link: Rationals and Radicals Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSA.REI.A.2, CCSS.MATH.CONTENT.HSN.RN.A.1, CCSS.MATH.CONTENT.HSF.IF.C.7 |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Rational Expressions and Functions • Rational Expressions |
| Copyright Year | 2022 |
| Keywords | radicals, radical expressions, rational numbers, rational expressions, definitions, glossary term, rational functions |