Display Title
Definition--Rationals and Radicals--Partial Fraction Decomposition of a Rational Expression
Display Title
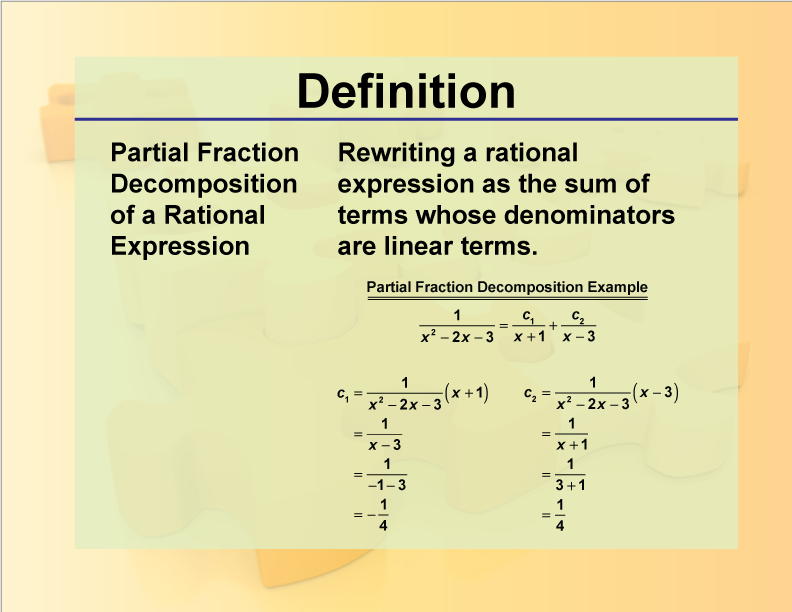
Partial Fraction Decomposition of a Rational Expression

Topic
Rationals and Radicals
Definition
Partial fraction decomposition is a method used to express a rational expression as a sum of simpler fractions.
Description
Partial Fraction Decomposition is a powerful tool in the study of Rational Numbers, Expressions, Equations, and Functions. It involves breaking down a complex rational expression into a sum of simpler fractions, which are easier to integrate or differentiate. For example, the rational function
2x+3(x+1)(x−2)
can be decomposed into
Ax+1+Bx−2
where A and B are constants determined by solving a system of equations. This technique is essential in calculus, particularly in the integration of rational functions. It simplifies the process of finding antiderivatives and helps in solving differential equations. Partial fraction decomposition is also used in control theory and signal processing, where it aids in analyzing and designing systems. By breaking down complex expressions, this method provides a clearer understanding of the underlying mathematical structure and facilitates the solution of problems in various fields.
For a complete collection of terms related to polynomials click on this link: Rationals and Radicals Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSA.REI.A.2, CCSS.MATH.CONTENT.HSN.RN.A.1, CCSS.MATH.CONTENT.HSF.IF.C.7 |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Rational Expressions and Functions • Rational Expressions |
| Copyright Year | 2022 |
| Keywords | radicals, radical expressions, rational numbers, rational expressions, definitions, glossary term, rational functions |