Display Title
Definition--Rationals and Radicals--Rational Functions
Display Title
Rational Functions

Topic
Rationals and Radicals
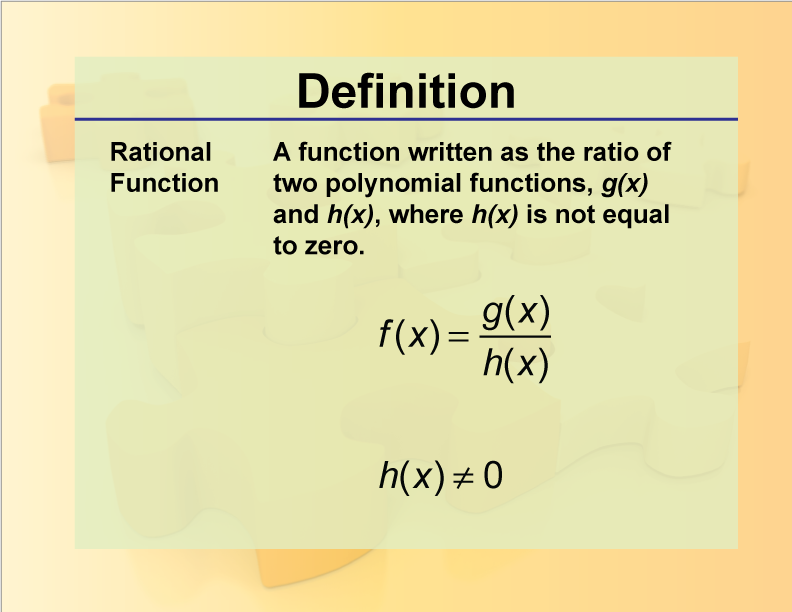
Definition
Rational functions are functions that are the ratio of two polynomials.
Description
Rational Functions are a key concept in the study of Rational Numbers, Expressions, Equations, and Functions. These functions are the ratio of two polynomials, such as
f(x)=P(x)Q(x)
where P(x) and Q(x) are polynomials. Understanding rational functions involves analyzing their behavior, including identifying asymptotes, intercepts, and discontinuities. For example, the function
f(x)=1x
has a vertical asymptote at x = 0 and a horizontal asymptote at y = 0. Rational functions are used to model various real-world phenomena, such as the relationship between supply and demand in economics. They also play a significant role in calculus, where they are used in limits, derivatives, and integrals. Mastery of rational functions enables students to graph these functions, solve equations, and apply these concepts to practical situations. Rational functions provide a deeper understanding of the properties of rational expressions and their behavior, leading to a more comprehensive grasp of mathematical concepts.
For a complete collection of terms related to polynomials click on this link: Rationals and Radicals Collection
| Common Core Standards | CCSS.MATH.CONTENT.HSA.REI.A.2, CCSS.MATH.CONTENT.HSN.RN.A.1, CCSS.MATH.CONTENT.HSF.IF.C.7 |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Rational Expressions and Functions • Rational Functions and Equations |
| Copyright Year | 2013 |
| Keywords | radicals, radical expressions, rational numbers, rational expressions, definitions, glossary term, rational functions |