Display Title
Definition--Ratios, Proportions, and Percents Concepts--The Constant of Proportionality
Display Title
The Constant of Proportionality
Topic
Ratios, Proportions, and Percents
Definition
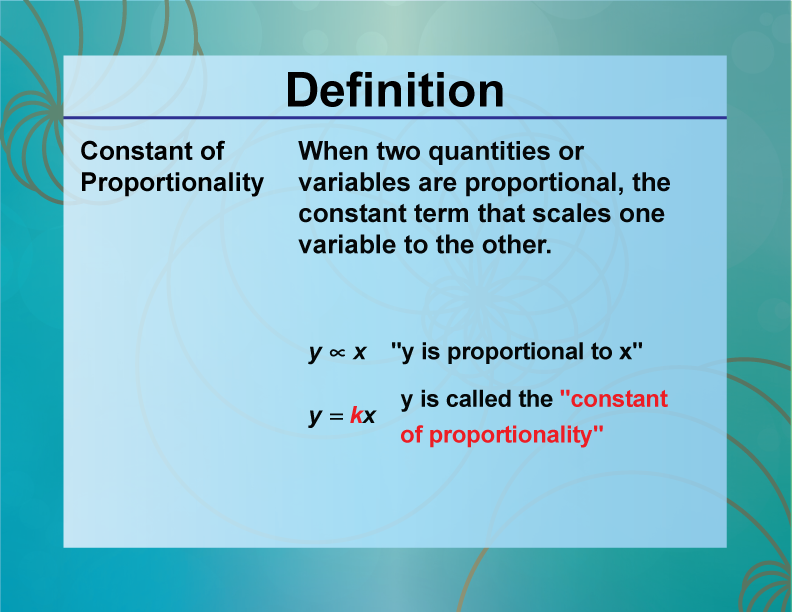
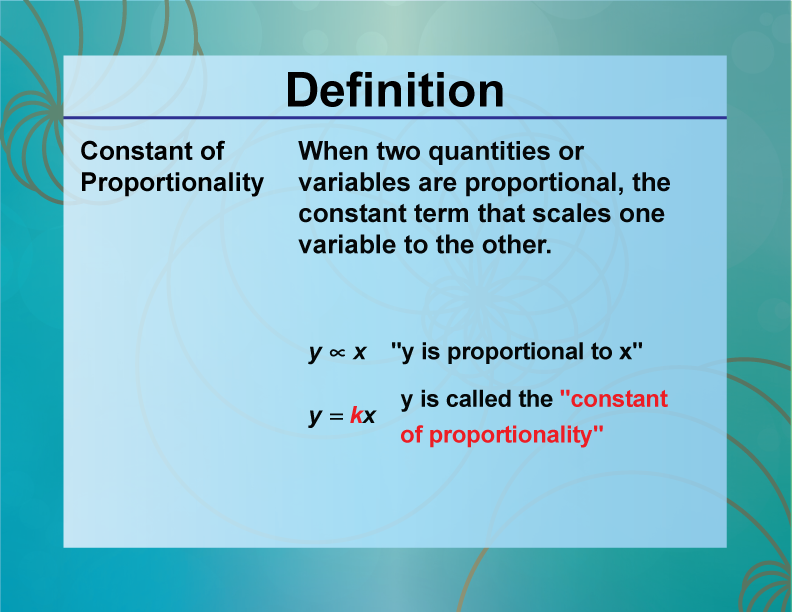
The constant of proportionality is the constant value that relates two proportional quantities.
Description
The constant of proportionality is a fundamental concept in mathematics, particularly in linear relationships and direct variation. For example, in the equation y = kx, k is the constant of proportionality that relates y and x. This concept is crucial in fields like physics, where it is used to describe relationships such as speed (distance/time).
In math education, understanding the constant of proportionality helps students grasp the concept of direct variation and linear relationships. For instance, if a car travels at a constant speed of 60 miles per hour, the distance traveled (d) is directly proportional to the time (t) with the constant of proportionality being 60. This knowledge is essential for solving problems involving proportional relationships and linear equations.
Teacher’s Script: "If a car travels at a constant speed of 60 miles per hour, the distance traveled is directly proportional to the time. To find the distance traveled use the equation: d = 60t, where t is the number of hours. The constant of proportionality is 60, meaning for every hour, the car travels 60 miles."

For a complete collection of terms related to Ratios, Proportions, and Percents click on this link: Ratios, Proportions, and Percents Collection
Watch the following video to learn about the constant of proportionality. (The transcript is also included.)
Constant of Proportionality

The video was uploaded on 10/15/2022.
You can view the video here.
The video lasts for 1 minute and 49 seconds.
Video Transcript
When two variables, x and y, are proportional, they are related by way of a constant of proportionality.
There are two ways a constant of proportionality works.
In the first case, as the value of x changes, the value of y changes in a corresponding manner, as you can see in this illustration.
The result is what looks like a straight line of points.
In function notation, the equation y = k*x captures the proportional relationship between x and y.
This type of function is called a direct variation.
The graph of y = kx is a straight line.
The coefficient k is the constant of proportionality.
The constant of proportionality can be positive or negative.
Here's an example of a negative constant of proportionality.
As x increases in value, y decreases in value in a consistent amount.
The graph is still a straight line, but a negative constant of proportionality results in a graph that slants downward, as shown here.
The constant of proportionality is the slope of the line.
The second type of constant of proportionality is called an inverse variation.
In these cases, as x increases in value, y decreases in value.
The equation for an inverse variation looks like this.
Notice the constant of proportionality, k, is the coefficient of the x-term.
The graphs of inverse variations are curves.
Both direct and inverse variations are used to model many real-world phenomena.
Often the constant of proportionality is found through measurement.
To learn more about the constant of proportionality, read the following background material.
Proportions
When two ratios are equal to each other, they can form an equation called a proportion. Look at this definition.
To learn more about proportions, click on this link. It is a presentation that goes over the proportions in more detail.
When proportions include a variable, this results in an equation that can be solved.
To learn more about solving proportions, click on this link. To see worked-out examples of solving proportions, click on this link.
Constant of Proportionality
When two variables x and y are proportional, they are related by way of a constant of proportionality. Look at this definition.
| Common Core Standards | CCSS.MATH.CONTENT.6.RP.A.1, CCSS.MATH.CONTENT.6.RP.A.2, CCSS.MATH.CONTENT.6.RP.A.3, CCSS.MATH.CONTENT.7.RP.A.3, CCSS.MATH.CONTENT.HSG.SRT.A.1.B, CCSS.MATH.CONTENT.7.RP.A.2.B |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Algebra • Ratios, Proportions, and Percents • Proportions |
| Copyright Year | 2021 |
| Keywords | ratios, Percent, definitions, glossary terms, rates, proportions, constant of proportionality |