Display Title
Definition--Sequences and Series Concepts--Arithmetic Sequence
Display Title
Arithmetic Sequence
Topic
Sequences and Series
Definition
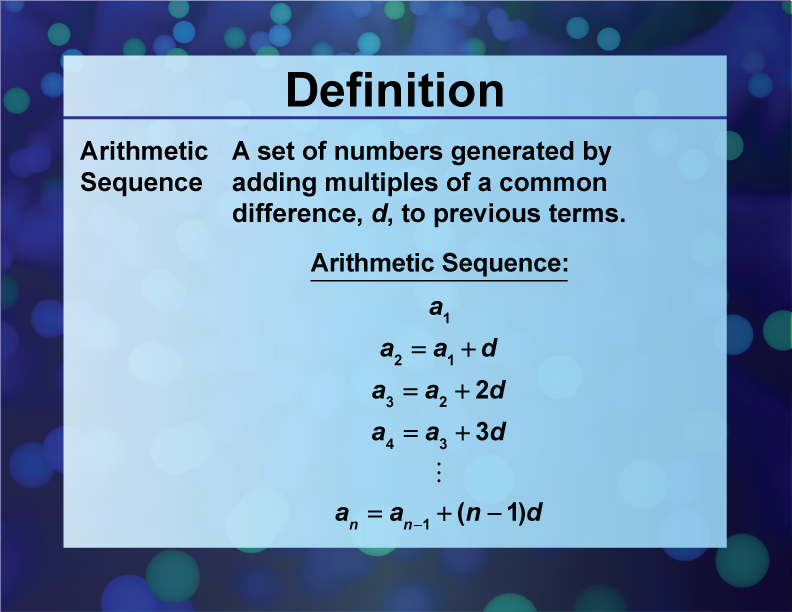
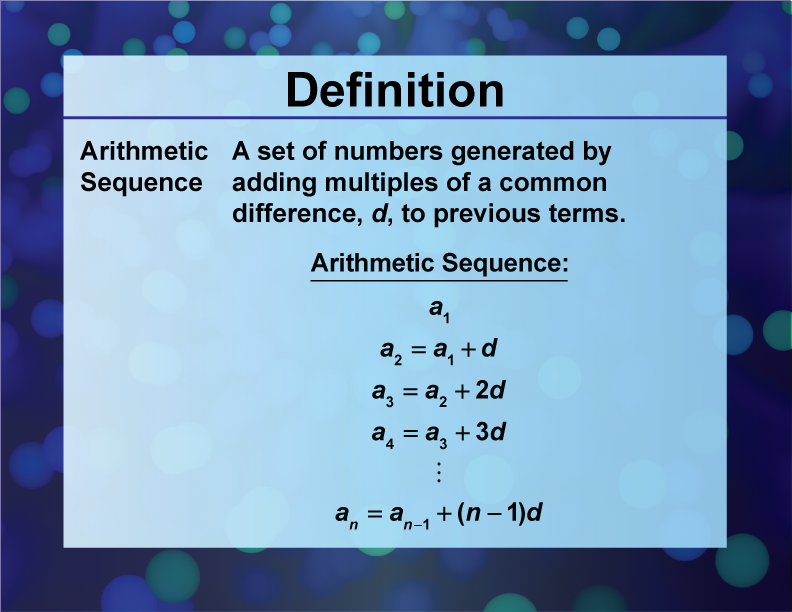
An arithmetic sequence is a sequence of numbers in which the difference between consecutive terms is constant.
Description
An arithmetic sequence is a fundamental concept in mathematics, particularly in the study of sequences and series. It is defined by the property that each term after the first is the sum of the previous term and a constant, known as the common difference. This concept is crucial in various mathematical applications, including solving problems related to linear growth and predicting future events based on past data.
In real-world applications, arithmetic sequences can be seen in situations where there is a consistent addition or subtraction of a fixed amount, such as in financial planning, where regular deposits or withdrawals are made. Algebraically, an arithmetic sequence can be expressed as
an =a1 + (n − 1)d
where an is the nth term, a1 is the first term, and d is the common difference.
Understanding arithmetic sequences is essential for math education as it lays the groundwork for more complex topics in algebra and calculus. It helps students develop skills in recognizing patterns and solving linear equations, which are foundational for advanced mathematical studies.
For a complete collection of terms related to sequences and series click on this link: Sequences and Series Collection
Sequences
Some number patterns are examples of sequences. A sequence is a set of numbers generated by applying the same rule to each term in the sequence.
Here is an example of a sequence.
In the example above, the rule “add 2” is applied to each term to generate the next term.
Arithmetic Sequences
An arithmetic sequence involves adding or subtracting the same amount to each subsequent term.
Here’s an example of an arithmetic sequence that involves subtraction.
With an arithmetic sequence, the term that keeps being added or subtracted is called the common difference. Here is an example of an arithmetic sequence with the common difference indicated.
The terms of an in a sequence can be listed symbolically, as shown below.
The first term is a1, the second term is a2, and so on to an. Any arithmetic sequence can be written this way.
Why would we write sequences this way? It makes it easier to see how each subsequent term is built from the previous term, as shown below.
The general form of an arithmetic sequence is known as a recursive formula. Furthermore, you can see how each term in the sequence is really based on two values, a1 and the common difference, c. This results in a different formula, shown below.
This form of the sequence equation is known as an explicit formula, as shown below.
Explicit formulas are extremely useful for finding any term in the sequence, as shown below.
Geometric Sequences
Another type of sequence is called a geometric sequence. Instead of adding or subtracting a number to generate terms, use multiplication. Here is an example.
This is the recursive formula for finding the nth term of a geometric sequence.
This is the explicit formula for a geometric sequence.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.HSF.IF.A.3, CCSS.MATH.CONTENT.HSF.BF.A.2, CCSS.Math.CONTENT.HSF.LE.A.2 |
|---|---|
| Grade Range | 6 - 9 |
| Curriculum Nodes |
Algebra • Sequences and Series • Sequences |
| Copyright Year | 2013 |
| Keywords | data analysis, arithmetic sequence, common difference, definitions, glossary terms, geometric sequence, common ratio |