Display Title
Definition--Sequences and Series Concepts--Fibonacci Sequence
Display Title
Fibonacci Sequence
Topic
Sequences and Series
Definition
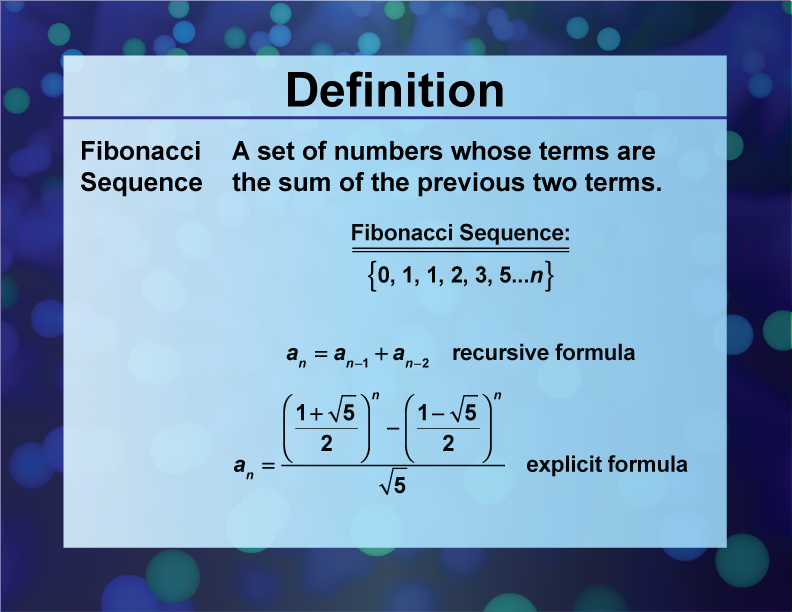
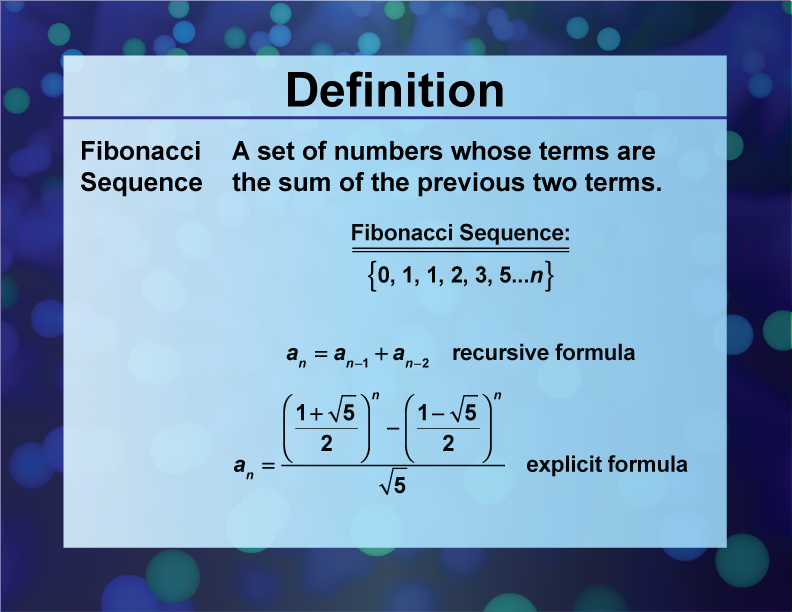
The Fibonacci sequence is a sequence where each term is the sum of the two preceding ones, starting from 0 and 1.
Description
The Fibonacci sequence is a famous sequence in mathematics, where each term is the sum of the two preceding ones, starting from 0 and 1. This sequence is significant in various mathematical and scientific applications, including computer science, biology, and art.
In real-world applications, the Fibonacci sequence is used in algorithm design, particularly in recursive algorithms. It is also observed in natural phenomena, such as the arrangement of leaves on a stem or the branching of trees. Algebraically, the Fibonacci sequence can be expressed as
Fn = Fn − 1 + Fn − 2
with initial conditions F0 = 0 and F1 = 1.
Understanding the Fibonacci sequence is crucial for math education as it provides a foundation for more advanced topics in number theory and combinatorics. It helps students develop skills in recognizing and analyzing patterns, which are essential for higher-level mathematics and various scientific fields.
For a complete collection of terms related to sequences and series click on this link: Sequences and Series Collection
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.HSF.IF.A.3, CCSS.MATH.CONTENT.HSF.BF.A.2, CCSS.Math.CONTENT.HSF.LE.A.2 |
|---|---|
| Grade Range | 6 - 9 |
| Curriculum Nodes |
Algebra • Sequences and Series • Sequences |
| Copyright Year | 2021 |
| Keywords | data analysis, arithmetic sequence, common difference, definitions, glossary terms, geometric sequence, common ratio |