Display Title
Definition--Sequences and Series Concepts--Geometric Sequence
Display Title
Geometric Sequence
Topic
Sequences and Series
Definition
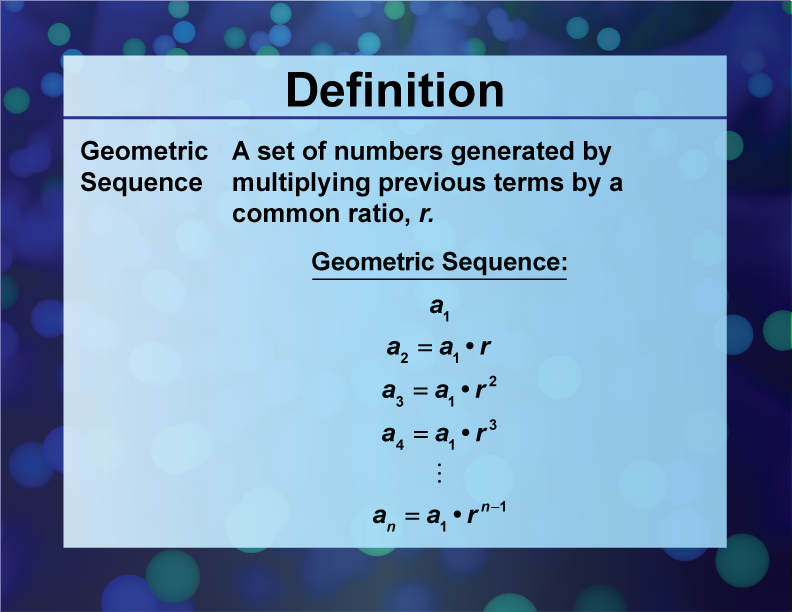
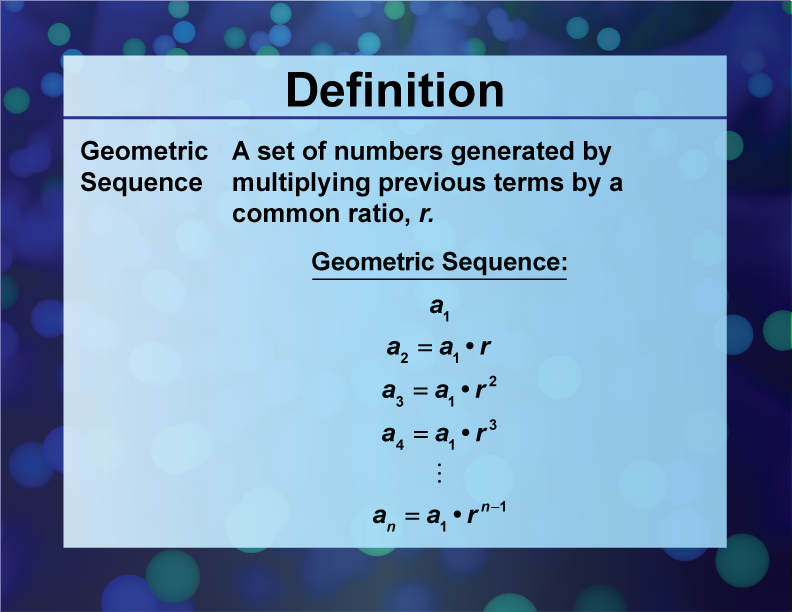
A geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous term by a fixed, non-zero number called the common ratio.
Description
A geometric sequence is a fundamental concept in mathematics, particularly in the study of sequences and series. It is defined by the property that each term after the first is the product of the previous term and a constant, known as the common ratio. This concept is crucial in various mathematical applications, including exponential growth and decay.
In real-world applications, geometric sequences can be seen in situations where there is consistent multiplication or division, such as in population growth, radioactive decay, and financial investments. Algebraically, a geometric sequence can be expressed as
an = a1 ⋅ r(n − 1)
where an is the nth term, a1 is the first term, and r is the common ratio.
Understanding geometric sequences is essential for math education as it lays the groundwork for more complex topics in algebra and calculus. It helps students develop skills in recognizing patterns and solving exponential equations, which are foundational for advanced mathematical studies.
For a complete collection of terms related to sequences and series click on this link: Sequences and Series Collection
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.HSF.IF.A.3, CCSS.MATH.CONTENT.HSF.BF.A.2, CCSS.Math.CONTENT.HSF.LE.A.2 |
|---|---|
| Grade Range | 6 - 9 |
| Curriculum Nodes |
Algebra • Sequences and Series • Sequences |
| Copyright Year | 2013 |
| Keywords | data analysis, arithmetic sequence, common difference, definitions, glossary terms, geometric sequence, common ratio |