Display Title
Definition--Slope Concepts--Zero Slope
Display Title
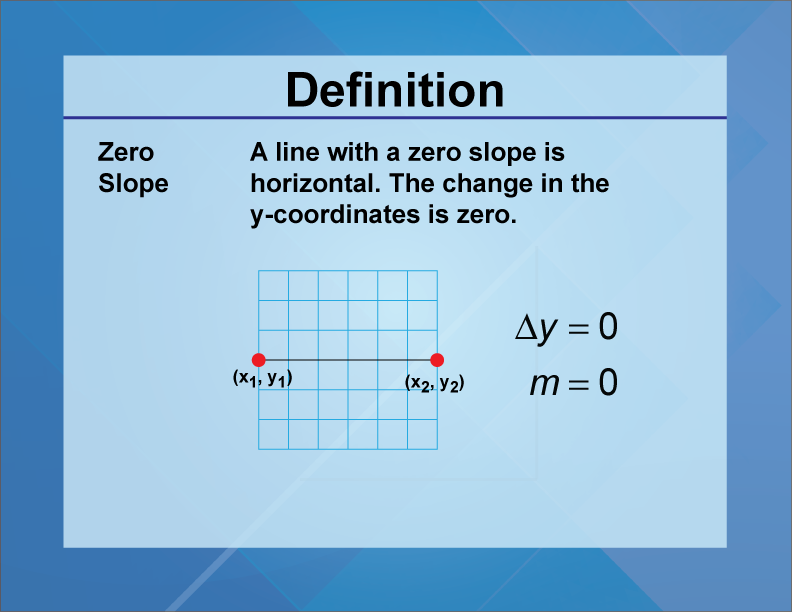
Zero Slope

Topic
Slope
Definition
Zero slope describes a horizontal line where there is no change in y as x changes.
Description
A zero slope is a key concept in algebra and geometry, representing a horizontal line. It is used in various applications, such as designing flat surfaces and analyzing constant relationships in data. In education, understanding zero slopes helps students in graphing and interpreting horizontal lines, enhancing their ability to analyze linear relationships.
For a complete collection of terms related to Slope click on this link: Slope Collection.
Slope Formula, Video 1

The video was uploaded on 10/15/2022.
You can view the video here.
The video lasts for 2 minutes and 24 seconds.
Video Transcript
This is the slope formula. Use it when you want to find the slope of the line that connects two points. It is the ratio of the Rise over the Run, or the ratio of this vertical distance over this horizontal distance.
In terms of the coordinates, it is the ratio in the difference in the y coordinates over the difference in the x coordinates. When you know the coordinates of two points, use this version of the slope formula.
Here are two points. This one has coordinates x1 and y1 and this one has coordinates x2 and y2. Use these coordinates in the slope formula to calculate the slope. Here are two points in Quadrant I.
Here are two points in Quadrant I. This point has coordinates 2, 3 and this point has coordinates 6,7. To find the slope of the line that crosses these two points, use the slope formula. For the purposes of the slope formula, let's call these coordinates x1 and y1, and let's call these coordinates x2 and y 2.
Take those coordinates and plug them into the slope formula. y2 goes here and y1 goes here. x2 goes here and x1 goes here. That means that 7 minus 3 in the numerator and 6 - 2 in the denominator. Simplify the numerator and denominator.
Simplify the numerator and denominator. Then simplify the expression. The slope of the line that crosses these two points has a slope of 1.
Watch this video to learn about zero slope. (The transcript is also included.)
Video Transcript
Slope is defined as the ratio of the change in the y-coordinates over the change in the x-coordinates.
When calculating slopes, the ratio is one number divided by another number.
A slope of zero means that the change in y-coordinates is zero.
What does "delta-y equals zero" correspond to?
Let's place two points on a coordinate grid, like this.
Delta-y is the vertical separation between the two points.
If delta-y equals zero, that means there is no vertical separation between the two points.
That means the two points are in this relative position.
Do you see what this means?
If you connect the points with a line, you'll see that this line is parallel with the x-axis.
Since the x-axis is a horizontal axis, that means a line with a slope of zero is a horizontal line.
It also means that each point has the same y-coordinate.
Let's look at an example.
Here are two points, A and B.
Point A has coordinates (1, 5).
Point B has coordinates (8, 5).
Notice that both coordinates have the same y-coordinate, 5.
To find the slope of the line connecting these two points, use the slope formula, as shown here.
Plug in the values of the coordinates into the formula.
Because the y-coordinates are identical, the value for delta-y is zero.
In fact, it doesn't matter what delta-x is.
The fact that delta-y is zero means that the slope of the line is zero.
So, what we've seen is that if two points have the same y-coordinate then the line connecting them has a slope of zero.
Since the equation for any line can be written in slope-intercept form, as shown below, what happens when the slope is zero?
A zero slope means that the x-term goes away.
What's left is the equation y = b, which is the equation of the horizontal line that crosses the y-intercept.
This is known as a constant function.
A line with zero slope is horizontal, which means that it doesn't slant one way or the other.
Slope is a ratio. Let’s first review what a ratio is.
Brief Review of Ratio
What is a ratio? It is a relationship between two quantities. Take a look at the definition.
Click on this link to learn more about ratios. This slide show includes a video and examples of different ratios. Here are some additional ratios topics you should review. Each of the following includes a link to a slide show with additional content:
- Ratios with Three Items: Explore ratios of the form a:b:c.
- Ratios with Fractions: Explore ratios where the the terms are made up of fractions.
- Equivalent Ratios: Explore ratios that represent the same amount.
Brief Review of Coordinates
Slope is the ratio of the difference in coordinates. Let’s briefly review the Cartesian coordinate system.
The Cartesian coordinate system consists of two axes that are perpendicular axes. The horizontal axis is labeled x and the vertical axis is labeled y.
Each axis is a number line that represents all positive and negative real numbers, as well as zero. There are four quadrants, which are labeled as shown.
A point on this coordinate grid has coordinates x and y, as shown below.
Slope
Slope is a ratio. See the definition below.
Recall that a ratio can look like a fraction and can be thought of as one number divided by another. What two numbers make up this ratio? As you can see from the definition, it’s the ratio of the change in y-coordinates over the change in x-coordinates.
Where do these changes in coordinates come from? Take a look at this example. There are two points, each with its own coordinates.
The difference in the y-coordinates is this:
The difference in the x-coordinates is this:
The ratio of these differences is shown below:
This ratio is also known as the slope formula.
| Common Core Standards | CCSS.MATH.CONTENT.8.EE.B.6 |
|---|---|
| Grade Range | 6 - 10 |
| Curriculum Nodes |
Algebra • Linear Functions and Equations • Slope |
| Copyright Year | 2021 |
| Keywords | slope, definitions, glossary term |