Display Title
Definition--Triangle Concepts--5-12-13 Right Triangle
Display Title
5-12-13 Right Triangle
Topic
Triangles
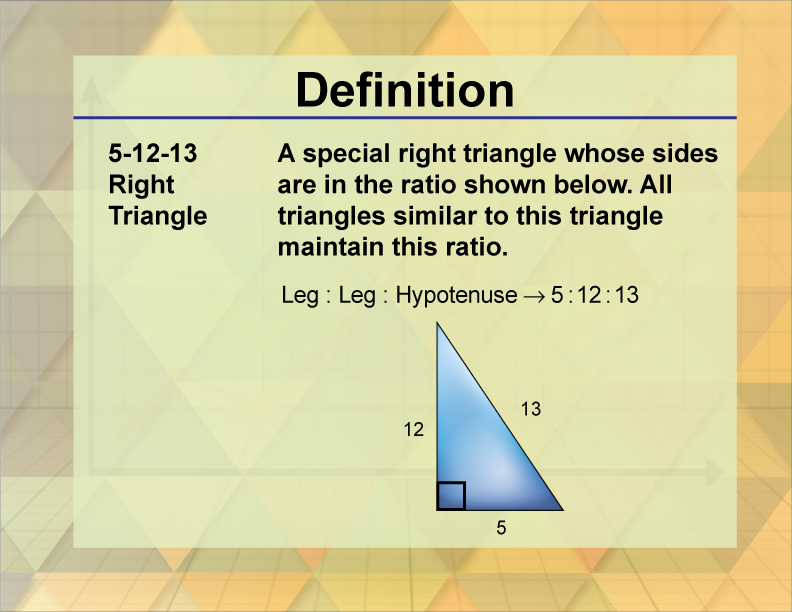
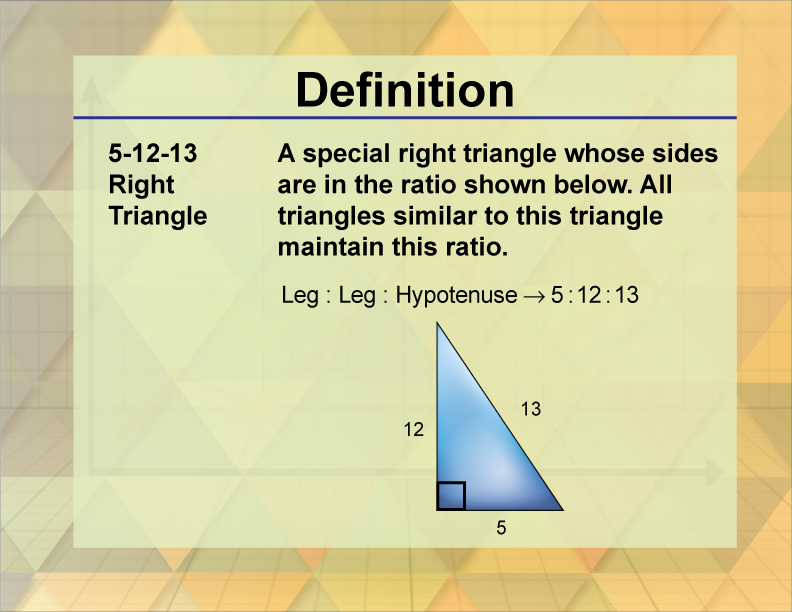
Definition
A 5-12-13 right triangle is a right triangle with side lengths in the ratio 5:12:13.
Description
The 5-12-13 right triangle is another example of a Pythagorean triple, where the sides satisfy the Pythagorean theorem:
52 + 122 = 132
This triangle is useful in various mathematical problems and real-world applications due to its simple integer side lengths.
In construction and design, the 5-12-13 triangle can be used to create right angles and ensure structural integrity. For example, if a builder needs to confirm a right angle, they can measure 5 units along one side, 12 units along the adjacent side, and if the diagonal measures 13 units, they have a right angle.
Understanding the 5-12-13 triangle is important in math education as it provides a clear example of the Pythagorean theorem and helps students understand the properties of right triangles. By working with this triangle, students can develop a deeper understanding of geometric principles and their practical applications.
For a complete collection of terms related to functions and relations click on this link: Triangles Collection
| Common Core Standards | CCSS.MATH.CONTENT.4.G.A.1, CCSS.MATH.CONTENT.7.G.A.2, CCSS.MATH.CONTENT.4.G.A.2, CCSS.MATH.CONTENT.6.G.A.1 |
|---|---|
| Grade Range | 4 - 8 |
| Curriculum Nodes |
Geometry • Triangles • Definition of a Triangle |
| Copyright Year | 2013 |
| Keywords | triangle, defnitions, glossary |