
Display Title
Definition--Triangle Concepts--Incenter of a Triangle
Display Title
Incenter of a Triangle
Topic
Triangles
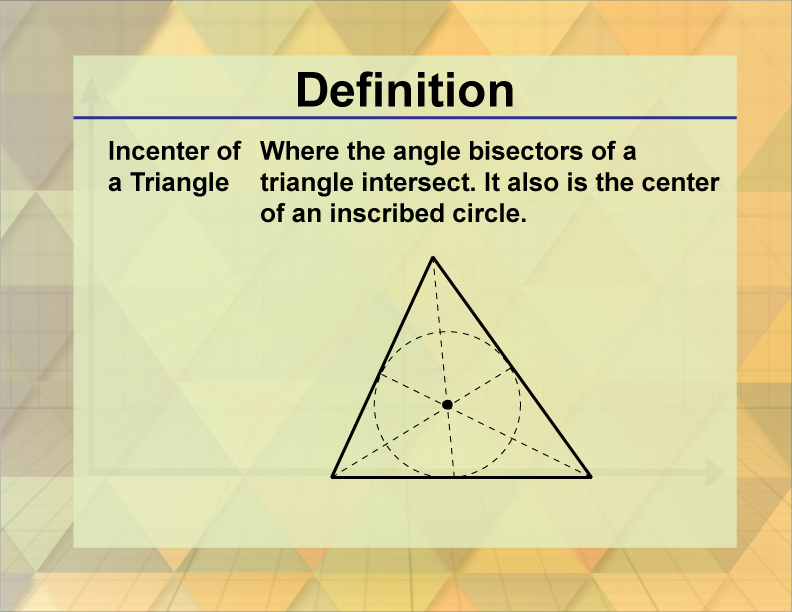
Definition
The incenter of a triangle is the point where the angle bisectors of the triangle intersect, and it is the center of the triangle's incircle.
Description
The incenter of a triangle is a significant point in geometry, representing the intersection of the triangle's angle bisectors. This point is equidistant from all three sides of the triangle, making it the center of the incircle, which is the circle that is tangent to all three sides.
This concept is essential in various real-world applications, such as in design and architecture, where precise measurements and symmetry are crucial. For example, understanding the incenter helps in creating balanced and aesthetically pleasing designs.
In math education, learning about the incenter helps students understand the broader concepts of geometry and the properties of triangles. It also provides a foundation for more advanced topics, such as coordinate geometry and calculus. By mastering this concept, students can apply their knowledge to solve practical problems and develop a deeper understanding of geometric principles.
For a complete collection of terms related to functions and relations click on this link: Triangles Collection
| Common Core Standards | CCSS.MATH.CONTENT.4.G.A.1, CCSS.MATH.CONTENT.7.G.A.2, CCSS.MATH.CONTENT.4.G.A.2 |
|---|---|
| Grade Range | 4 - 8 |
| Curriculum Nodes |
Geometry • Triangles • Definition of a Triangle |
| Copyright Year | 2020 |
| Keywords | triangle, defnitions, glossary |