Display Title
Elementary Math Definitions--Addition Subtraction Concepts--Associative Property
Display Title
Associative Property
Topic
Addition and Subtraction
Definition
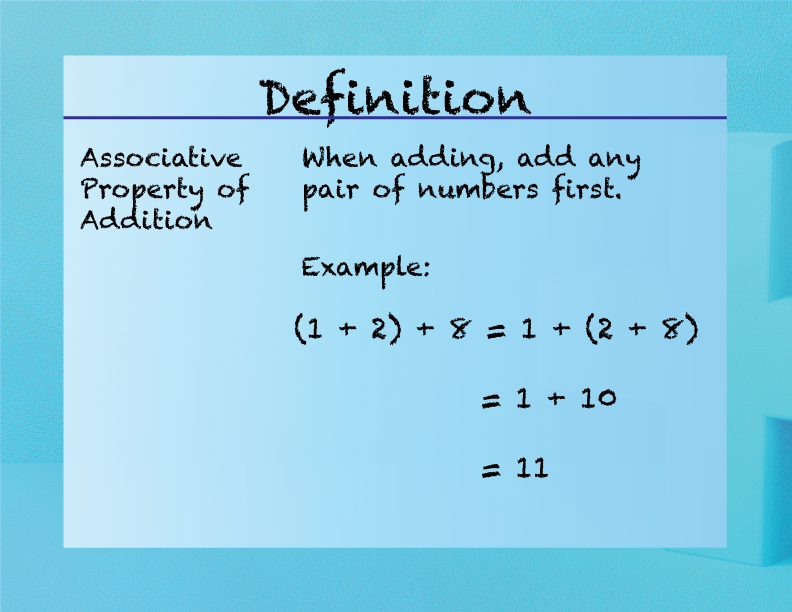
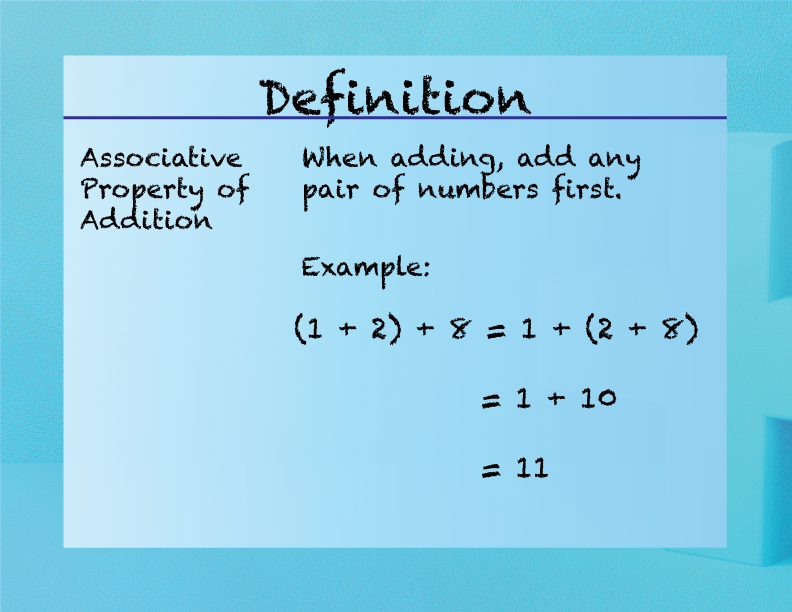
The associative property states that the way in which numbers are grouped in addition does not change their sum.
Description
The associative property is a fundamental concept in mathematics that applies to addition and multiplication. It states that the way numbers are grouped when being added does not affect the sum. For example, in the expression
(2 + 3) + 4 = 2 + (3 + 4)
both groupings result in the same sum of 9.
This property is essential for simplifying complex calculations and solving algebraic equations. It allows for flexibility in grouping numbers, making it easier to perform mental math and understand mathematical relationships. The associative property is also a building block for more advanced mathematical concepts, such as algebra and calculus.
In real-world applications, the associative property can be seen in various scenarios, such as calculating the total cost of items, determining the total distance traveled, and more. For example, if you buy 2 apples, 3 oranges, and 4 bananas, the associative property allows you to group the fruits in any order to find the total number.
Teacher's Script: "Let's learn about the associative property. This property tells us that it doesn't matter how we group numbers when we add them; the total will be the same. For example, if we add 2 and 3 first, then add 4, we get the same total as if we add 3 and 4 first, then add 2. Can you try grouping numbers in different ways and see if the total stays the same?"
For a complete collection of terms related to Addition and Subtraction click on this link: Addition and Subtraction Collection
| Common Core Standards | CCSS.MATH.CONTENT.1.OA.B.3, CCSS.MATH.CONTENT.2.NBT.B.9 |
|---|---|
| Grade Range | 1 - 3 |
| Curriculum Nodes |
Arithmetic • Addition • Addition Expressions and Equations |
| Copyright Year | 2021 |
| Keywords | addition, subtraction, equation, place value |