
Display Title
Math Clip Art--Applications of Linear Functions: Cost vs. Time 07
Display Title
Math Clip Art--Applications of Linear Functions: Cost vs. Time 07
Topic
Linear Functions
Description
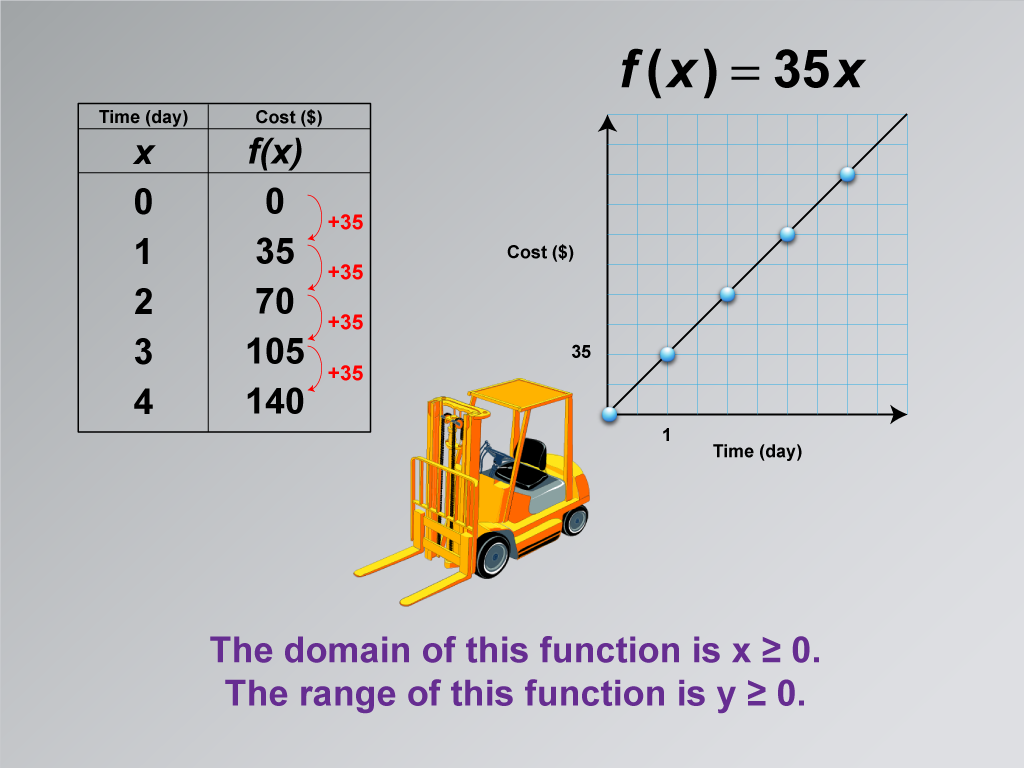
This math clip art image is the seventh in a series dedicated to illustrating applications of linear functions, specifically focusing on building a linear function model for equipment rental costs. The image builds upon the previous one by introducing the concepts of domain and range in the context of the rental cost function. It continues to feature the construction equipment scenario, emphasizing the practical constraints on the function's input and output values.
The caption explicitly states that the domain of the function is x ≥ 0, and the range is y ≥ 0. This information is crucial for understanding the real-world limitations of the mathematical model. It reinforces the idea that in this practical scenario, negative time or negative costs are not meaningful. The domain restriction (x ≥ 0) indicates that rental time cannot be negative, while the range restriction (y ≥ 0) shows that the rental cost will always be non-negative.
Using such math clip art in teaching linear functions is invaluable for helping students connect abstract mathematical concepts to real-world constraints. This image bridges the gap between pure mathematics and practical applications, allowing students to see how theoretical concepts like domain and range have tangible meanings in real situations. Incorporating these visuals into lessons can significantly enhance student understanding of the limitations and interpretations of mathematical models in real-world contexts, fostering a deeper appreciation for the practical applications of mathematics.
Teacher's Script
Let's consider the domain and range of our rental cost function. The domain, x ≥ 0, tells us that we can't have negative time. Why do you think this makes sense in our rental scenario? Similarly, the range, y ≥ 0, indicates that our cost will never be negative. How does this relate to our equation and graph? Can you think of other real-world situations where we might have similar restrictions on domain and range?
For a complete collection of math clip art related to Applications of Linear Functions click on this link: Applications of Linear Functions: Cost vs. Time Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.F.A.2, CCSS.MATH.CONTENT.8.F.A.3, CCSS.MATH.CONTENT.HSF.LE.A.1.A |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Linear Functions and Equations • Graphs of Linear Functions • Slope-Intercept Form |
| Copyright Year | 2021 |
| Keywords | linear functions |