
Display Title
Math Clip Art--Applications of Linear Functions: Hooke's Law 03
Display Title
Math Clip Art--Applications of Linear Functions: Hooke's Law 03
Topic
Linear Functions
Description
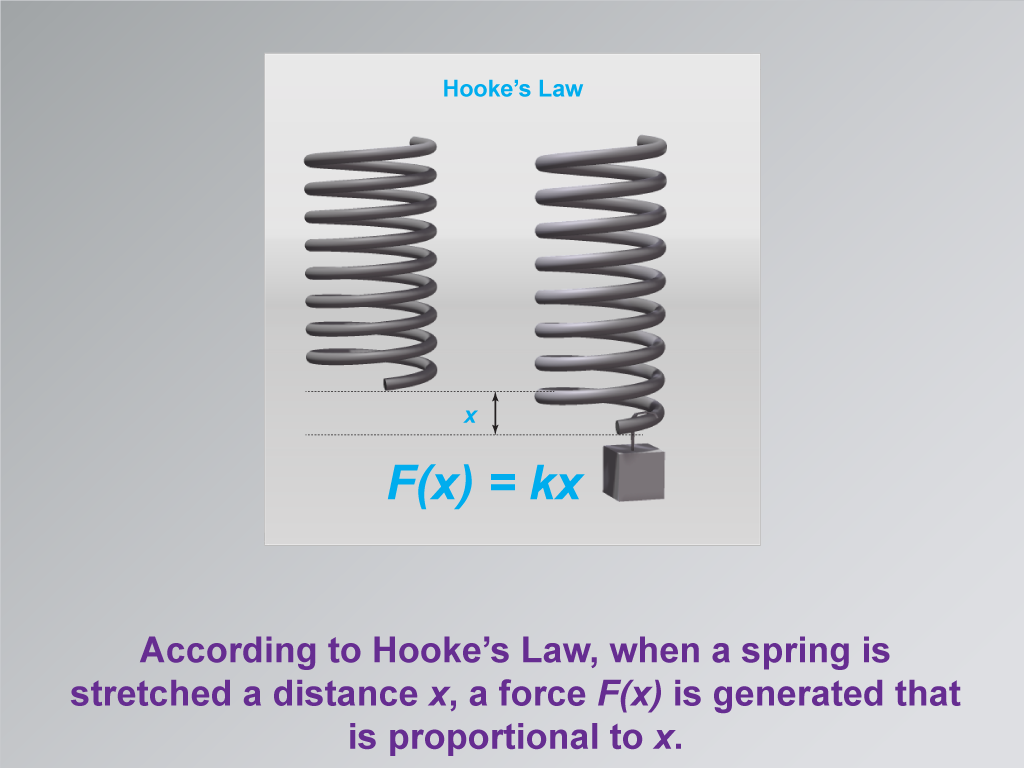
This image is a continuation of the series on applications of linear functions, specifically illustrating Hooke's Law. It builds upon the previous image by introducing the mathematical equation F(x) = kx, which is the core of Hooke's Law.
The illustration shows that when a spring is stretched a distance x, a force F(x) is generated that is proportional to x. This visual representation, combined with the equation, helps students understand the linear relationship between force and displacement in springs.
Incorporating such math clip art into lessons is crucial for effectively teaching complex mathematical concepts. It provides a visual anchor for the abstract equation, helping students connect the mathematical expression with its physical meaning. These images can be seamlessly integrated into lessons on linear functions, enhancing students' understanding of how mathematical models describe real-world phenomena.
A teacher might explain this image by saying: "Now that we've seen how a spring stretches when we apply a force, let's look at the math behind it. This equation, F(x) = kx, is Hooke's Law. It tells us that the force (F) needed to stretch or compress a spring is directly proportional to the distance (x) it's stretched or compressed. The 'k' is a constant that depends on how stiff the spring is. This is a perfect example of a linear function in action!"
For a complete collection of math clip art related to Applications of Linear Functions click on this link: Applications of Linear Functions: Hooke's Law Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.F.A.2, CCSS.MATH.CONTENT.8.F.A.3, CCSS.MATH.CONTENT.HSF.LE.A.1.A |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Linear Functions and Equations • Graphs of Linear Functions • Slope-Intercept Form • Ratios, Proportions, and Percents • Proportions |
| Copyright Year | 2021 |
| Keywords | linear functions, constant of proportionality |