Display Title
Math Clip Art--Function Concepts--Function Graphs 02
Display Title
Math Clip Art--Function Concepts--Function Graphs 02
Topic
Functions
Description
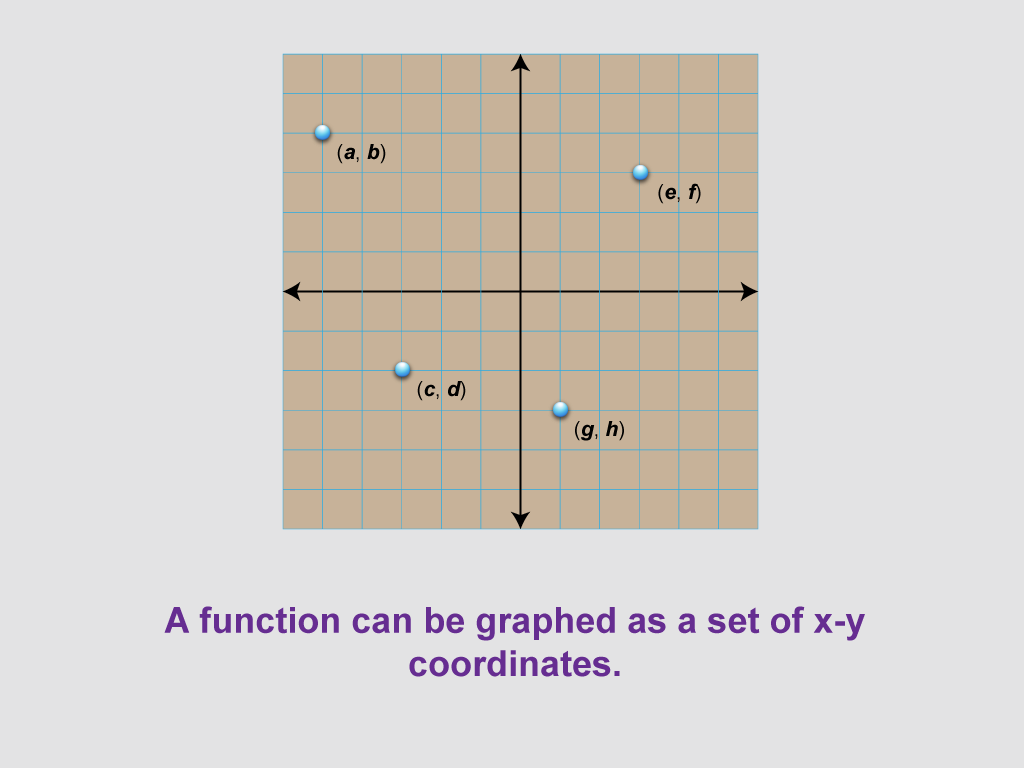
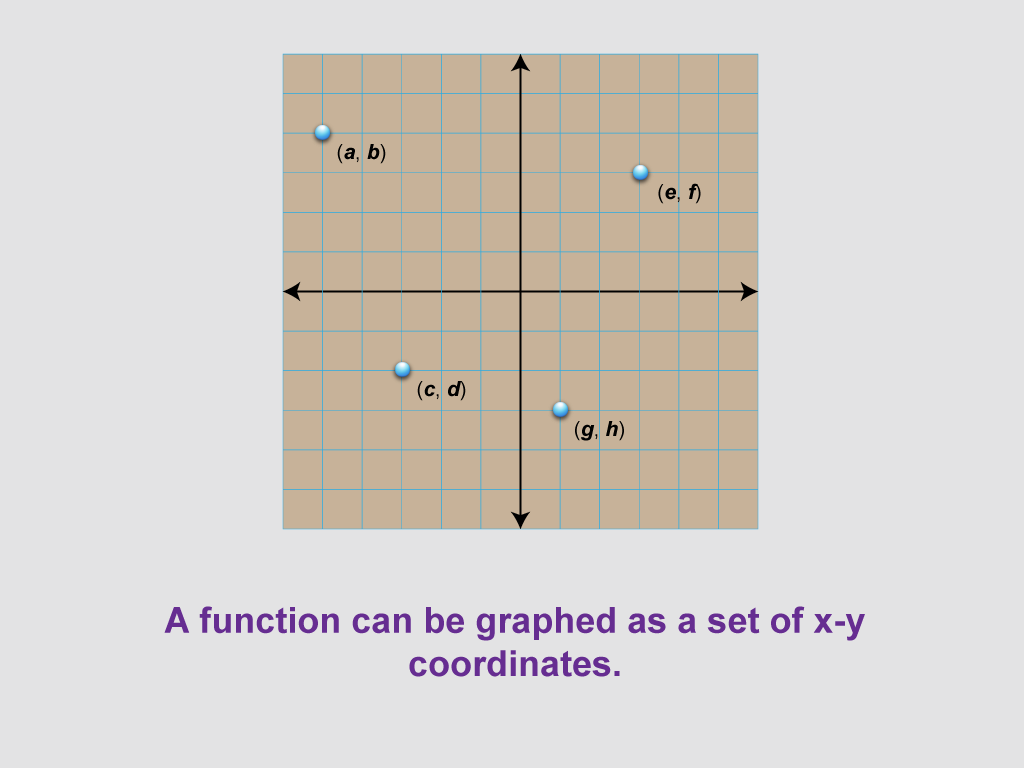
This image presents a graph with points labeled (a, b), (c, d), (e, f), and (g, h) without connecting lines. It illustrates that a function can be graphed as a set of x-y coordinates, emphasizing the discrete nature of data points in a function.
The unconnected points on the graph serve to introduce the concept of plotting individual coordinates to represent a function. This visualization helps students understand that functions can be defined by specific pairs of input and output values, without necessarily implying continuity between these points. It's an excellent starting point for discussing discrete functions and the importance of domain and range in function analysis.
Using this type of visual representation allows educators to emphasize the fundamental concept of ordered pairs in functions. Students can learn to identify the x and y components of each point, understanding how they relate to the input and output of a function. This image also provides an opportunity to discuss the difference between discrete and continuous functions, setting the stage for more complex function representations.
Teacher's Script: "Take a look at this graph with labeled points. What do you notice about how these points are arranged? Can you identify the x and y coordinates for each point? Let's discuss how these individual points can represent a function and what information we can gather from this type of representation."
For a complete collection of math clip art related to Functions click on this link: Function Representations Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.A.1, CCSS.MATH.CONTENT.HSF.IF.A.2, CCSS.MATH.CONTENT.HSF.IF.C.8, CCSS.MATH.CONTENT.HSF.BF.A.1, CCSS.MATH.CONTENT.HSF.BF.A.1.A |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Functions and Relations • Relations and Functions |
| Copyright Year | 2020 |
| Keywords | functions |