Display Title
Math Clip Art--Geometry Basics--Polygon Basics 09
Display Title
Math Clip Art--Geometry Basics--Polygon Basics 09
Topic
Geometry Basics
Description
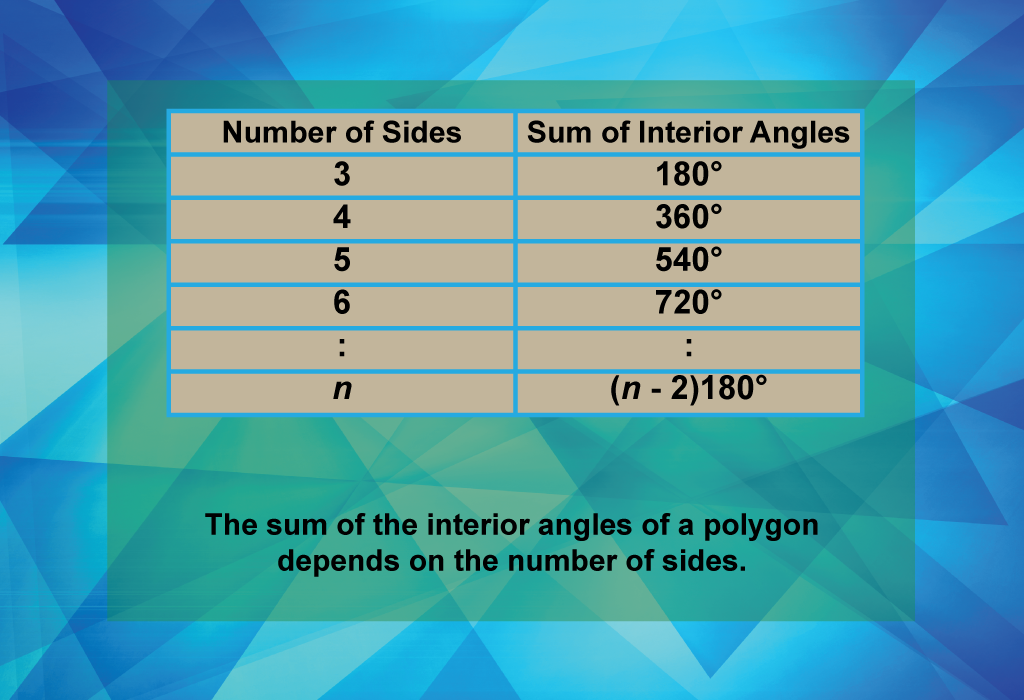
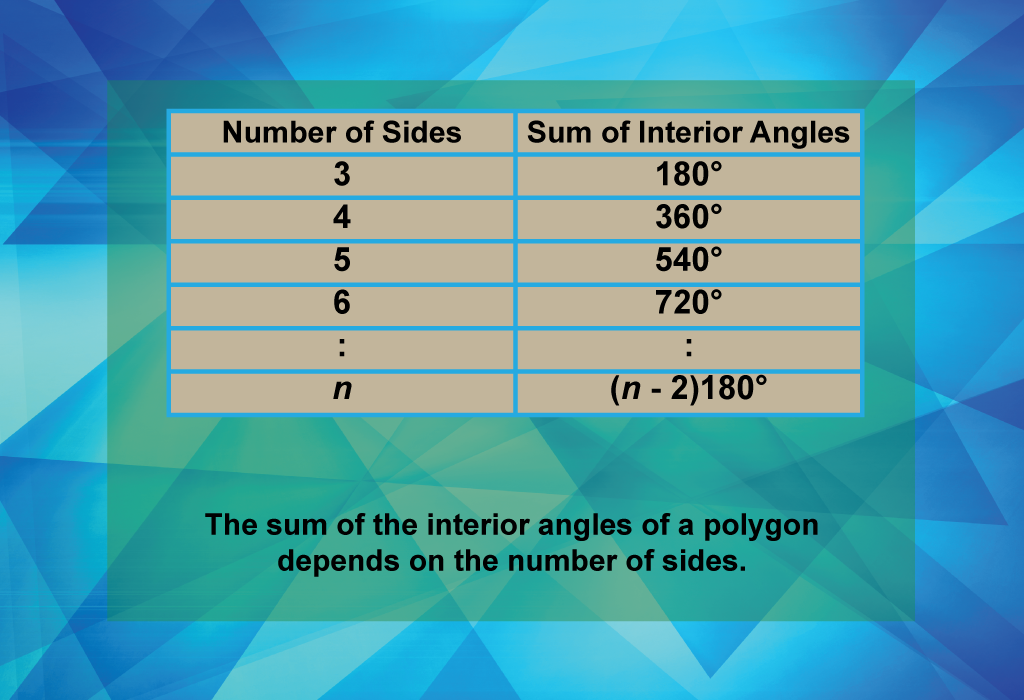
This image is part of a series designed to teach fundamental concepts about polygons. It presents a table that illustrates the relationship between the number of sides in a polygon, the number of triangles that can be formed within it, and the sum of its interior angles. The caption emphasizes that the sum of interior angles depends on the number of sides, reinforcing this crucial geometric principle.
Incorporating such math clip art into geometry lessons can significantly enhance students' understanding of polygon properties. The table format provides a clear, systematic way to visualize how these properties change as the number of sides increases. This visual aid serves as an excellent tool for helping students recognize patterns and deduce the formula for calculating the sum of interior angles in any polygon.
Teacher's Script: "Let's examine this table together. Notice how as the number of sides increases, both the number of triangles that can be formed within the polygon and the sum of its interior angles also increase. Can you spot any patterns? For instance, how does the number of triangles relate to the number of sides? And what about the sum of interior angles - can you figure out how it's calculated? Use this table to make predictions about polygons with even more sides. This relationship is key to understanding more complex geometric concepts we'll explore later."
For a complete collection of math clip art related to Polygons click on this link: Geometry Basics: Polygons Collection.
| Common Core Standards | CCSS.MATH.CONTENT.3.MD.D.8 |
|---|---|
| Grade Range | 3 - 5 |
| Curriculum Nodes |
Geometry • Polygons • Definition of a Polygon |
| Copyright Year | 2021 |
| Keywords | polygons |