Display Title
Math Clip Art: Parallel Lines Cut by a Transversal 9
Display Title
Math Clip Art: Parallel Lines Cut by a Transversal 9
Topic
Geometry
Description
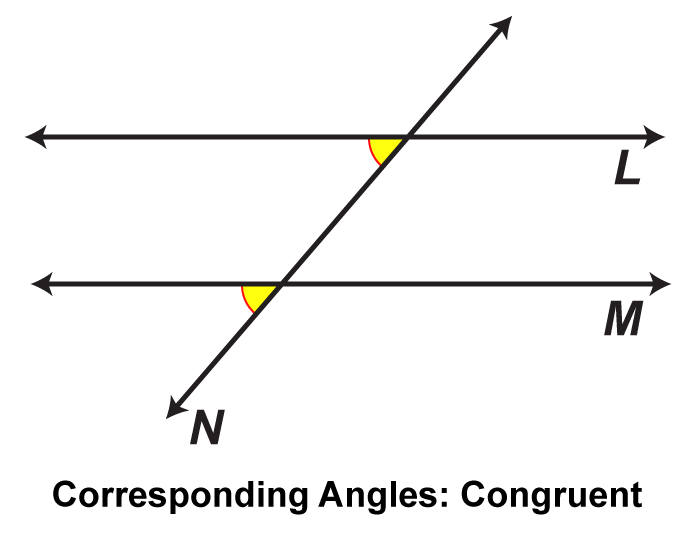
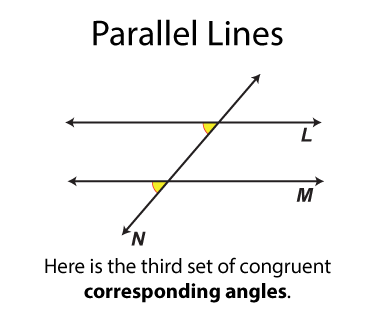
This math clip art image is part of a series illustrating the properties of parallel lines cut by a transversal. It highlights yet another pair of corresponding angles, further reinforcing the concept that these angles are congruent when parallel lines are cut by a transversal. The image uses a unique color coding to identify this third pair of corresponding angles, providing students with multiple examples of this geometric relationship.
Incorporating math clip art like this into geometry lessons can greatly enhance students' comprehension of abstract concepts. The repeated visual representation of corresponding angles helps students solidify their understanding of this concept more effectively than verbal explanations alone. This image can be seamlessly integrated into lessons on geometry, serving as a powerful tool for reinforcing the concept of corresponding angles and their congruence.
Teacher's Script: "Let's examine this third pair of corresponding angles, class. Notice how they're highlighted in a different color from the previous examples. Can you see the pattern forming? All pairs of corresponding angles are congruent when we have parallel lines cut by a transversal. This consistency is what makes these relationships so powerful in geometry. How might we use this knowledge to solve more complex geometric problems or prove other theorems?"
For a complete collection of math clip art related to Geometry click on this link: Parallel Lines Cut by a Transversal Collection.
Parallel Lines Cut by a Transversal
Parallel lines are on the same plane and do not intersect. Here are two lines, L and M, that are on plane P and parallel.
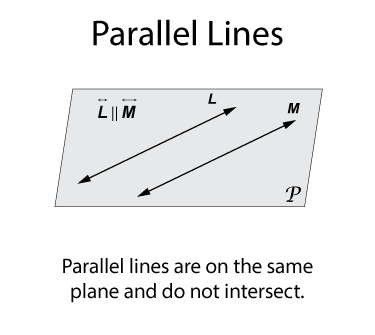
Parallel lines are are always the same distance from each other. In this illustration the dashed segment indicates the distance between the two lines. That distance doesn't change.
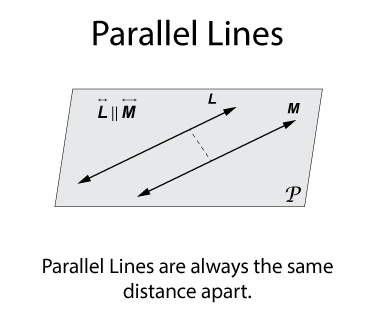
A line that intersects the parallel lines is called a transversal. In the illustration below you can see transveral N that insersects lines L and M.
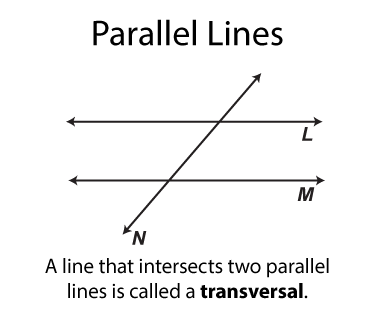
When parallel lines are cut by a transversal, there are number of set of angles whose properties are important to remember. The categories of angles include:
- Alternate interior angles
- Alternate exterior angles
- Same side interior angles
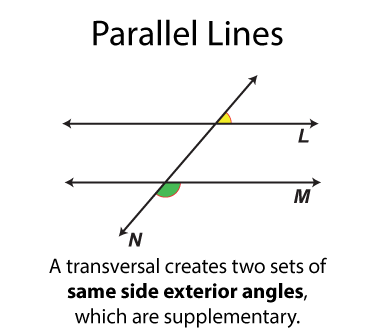
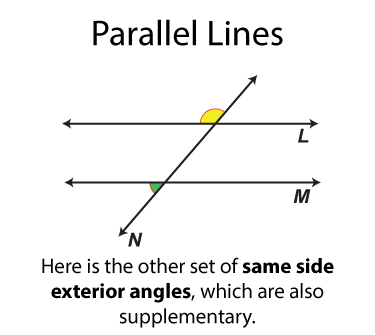
- Same side exterior angles
- Supplementary angles
- Vertical angles
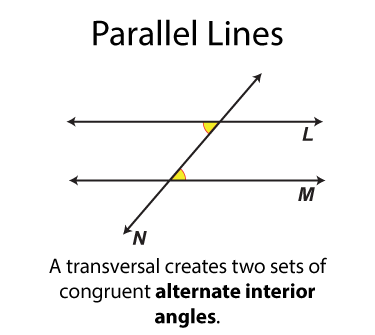
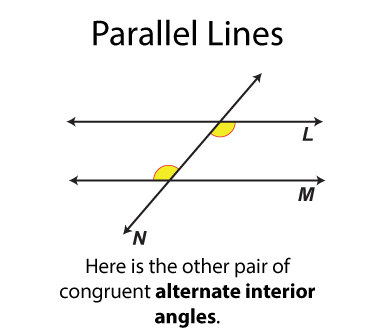
Let's start with the alternate interior angles, which are shown here. There are two sets of alternate interior angles. These pairs of angles are congruent. The word "alternate" means "opposite" In each case the one angle is on the opposite side of the transversal from the other angle.
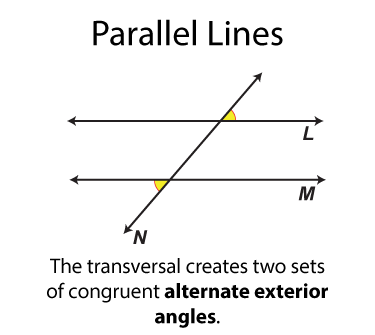
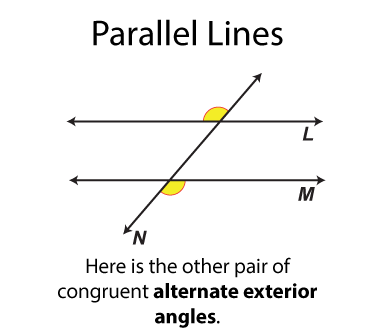
The next set of angles are called alternate exterior angles. There are two sets. Each set of angles is congruent. Each angle is on one side of the transversal from the other angle it is congruent to.
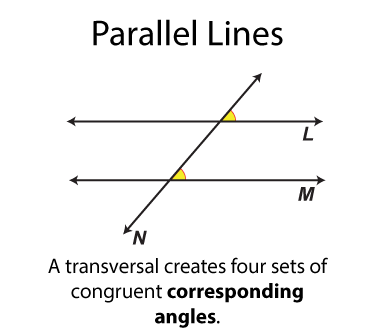
The next set of angles are called corresponding angles. There are four sets. Each set of angles is congruent. Each set of angles is on the same side of the transversal.

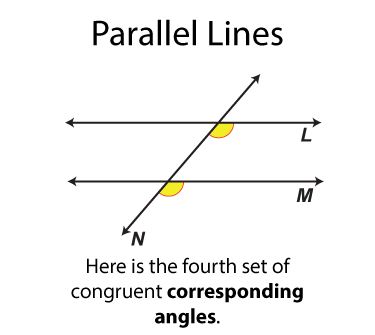
The next set of angles are called vertical angles. There are two sets. Each set of angles is congruent. (By definition all vertical angles are congruent.) Each angle is on on the opposite side of the transversal from the other angle it is congruent to.
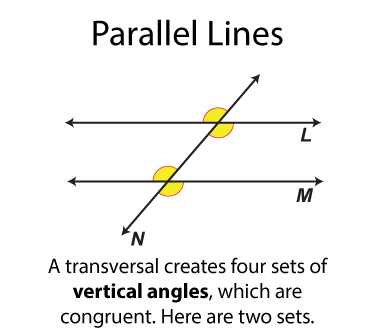
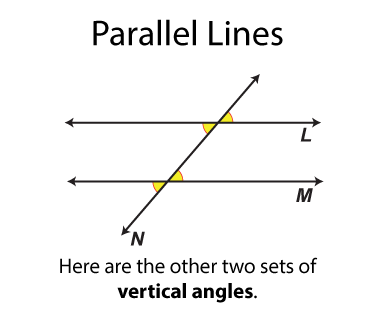
The next set of angles are called vertical angles. There are two sets. Each set of angles is congruent. (By definition all vertical angles are congruent.) Each angle is on on the opposite side of the transversal from the other angle it is congruent to.


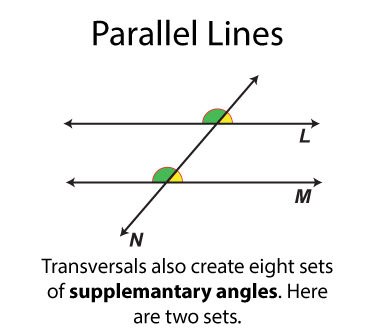
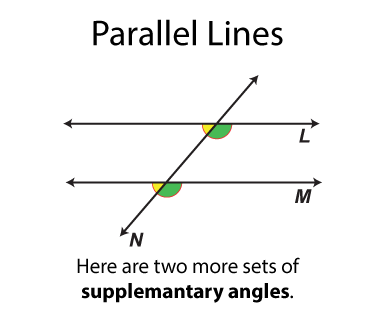
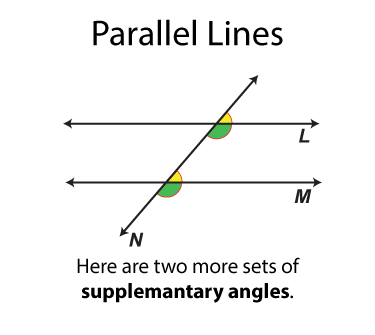
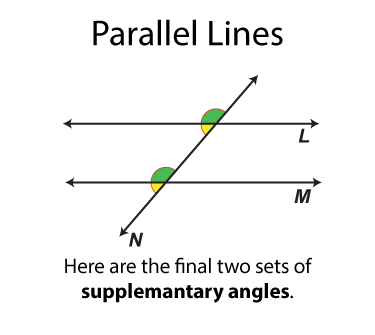
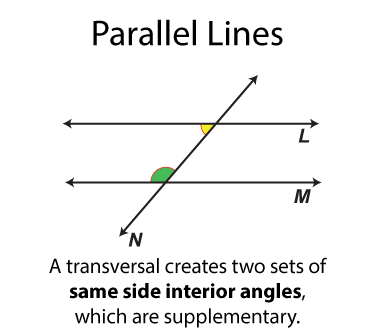
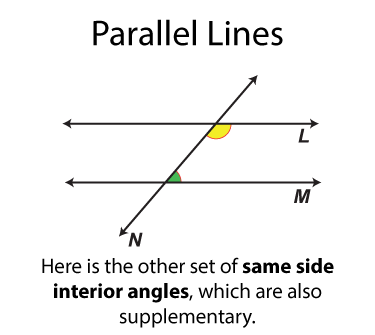
The next set of angles are supplementary angles. There are eight sets. By definition the supplementary angles add up to 180°. Some pairs of supplementary angles are on opposite sides of the transversal and some are on the same side.
| Common Core Standards | CCSS.MATH.CONTENT.4.G.A.2, CCSS.MATH.CONTENT.8.G.A.5 |
|---|---|
| Grade Range | 4 - 8 |
| Curriculum Nodes |
Geometry • Points and Lines • Parallel Lines |
| Copyright Year | 2020 |
| Keywords | Parallel Lines Cut by a Transversal, parallel lines, transversal, vertical angles, supplementary angles, alternate interior angles, alternate exterior angles |