Display Title
Math Example--Area and Perimeter--Surface Area: Example 23
Display Title
Math Example--Area and Perimeter--Surface Area: Example 23
Topic
Surface Area
Description
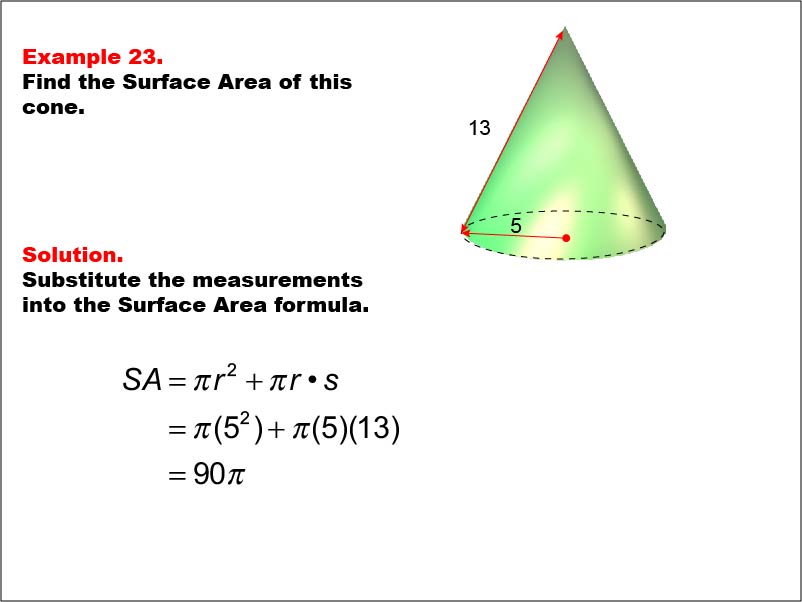
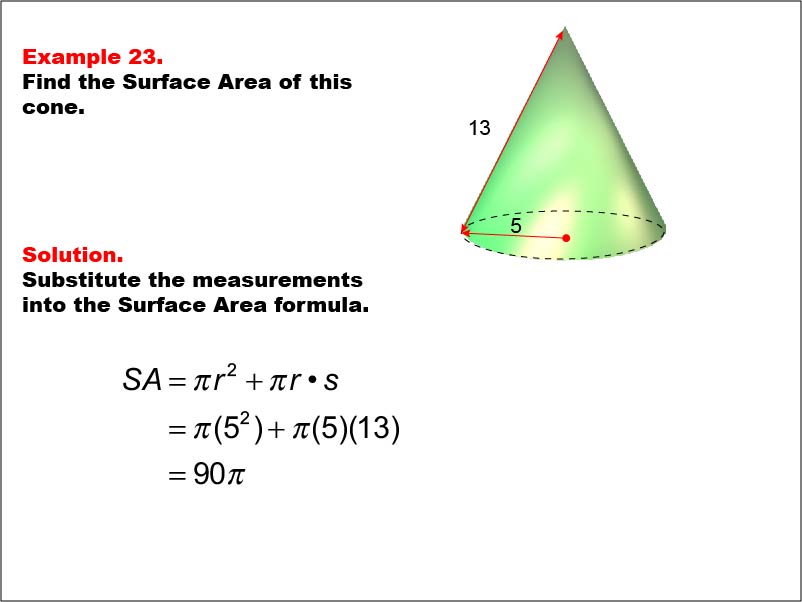
This example demonstrates the calculation of surface area for a cone with a radius of 5 units and a slant height of 13 units. The surface area is calculated using the formula SA = π * r2 + π * r * s, where r is the radius and s is the slant height. For this specific cone, the calculation yields a surface area of 90π square units.
Understanding surface area calculations for three-dimensional curved shapes like cones is essential in mathematics and its applications. This collection of examples helps teach the topic by introducing different types of solids, allowing students to apply surface area principles to more diverse shapes and reinforce their understanding of geometric relationships, especially those involving curved surfaces.
Exposure to multiple worked-out examples is crucial for students to fully grasp the concept of surface area across different shapes. Each example builds upon previous knowledge while introducing new geometric forms, helping students develop versatility in their problem-solving approach and a deeper understanding of spatial relationships, particularly when dealing with curved surfaces and the use of pi in calculations.
Teacher's Script: In this example, we're exploring the surface area of a cone. Notice how the formula for a cone's surface area includes both the circular base and the curved surface. As we work through this example, think about why we need both the radius and the slant height to calculate the surface area. Understanding these concepts will help you tackle surface area problems for various curved shapes in the future and appreciate the relationship between different parts of a three-dimensional object.
For a complete collection of math examples related to Surface Area click on this link: Math Examples: Surface Area Collection.
| Common Core Standards | CCSS.MATH.CONTENT.7.G.B.6 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Geometry • Surface Area and Volume • Surface Area |
| Copyright Year | 2013 |
| Keywords | surface area |