Display Title
Math Example: Comparing Area: Example 8
Display Title
Math Example: Comparing Area: Example 8

Topic
Geometry
Description
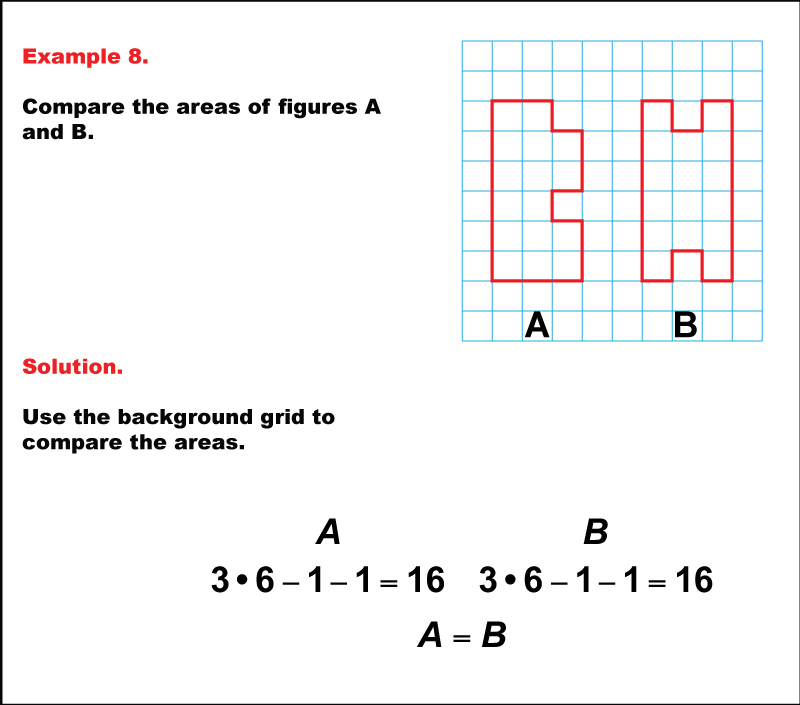
This example compares the areas of two complex shapes (Figures A and B) made up of rectangles, displayed on a grid. Both figures have identical areas calculated as 3 * 6 - 1 - 1 = 16 square units. By comparing these results, we can conclude that A = B, meaning both shapes have equal areas despite their different appearances.
This example is part of a series designed to teach students about comparing areas of different geometric shapes. By presenting complex shapes composed of rectangles, students learn to break down composite figures into simpler shapes and calculate their areas. This approach helps reinforce the concept that area is conserved even when a shape is rearranged, and that visually different shapes can have identical areas.
Exposing students to multiple worked-out examples is essential for developing a deep understanding of area comparison, especially for complex shapes. Each example provides a unique scenario, allowing students to apply their knowledge to new situations. This repetition helps solidify the concept and improves students' ability to tackle various area-related problems independently, particularly when dealing with composite figures.
Teacher Script: "Now we're looking at two shapes that appear quite different. How can we find their areas? Let's break them down into rectangles and subtract the missing parts. What do you notice about the results? Even though the shapes look different, they have the same area. This is an important concept in geometry -- shapes can be rearranged without changing their area. Can you think of any real-world examples where this might be useful, like in architecture or design?"
For a complete collection of math examples related to Geometry click on this link: Math Examples: Comparing Areas Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.G.A.1 |
|---|---|
| Grade Range | 6 - 7 |
| Curriculum Nodes |
Geometry • Quadrilaterals • Area and Perimeter of Quadrilaterals • Triangles • Area and Perimeter of Triangles • Circles • Area and Circumference |
| Copyright Year | 2024 |
| Keywords | comparing areas |