Display Title
Math Example--Complex Numbers--Complex Coordinates--Example 4
Display Title
Math Example--Complex Numbers--Complex Coordinates--Example 4
Topic
Complex Numbers
Description
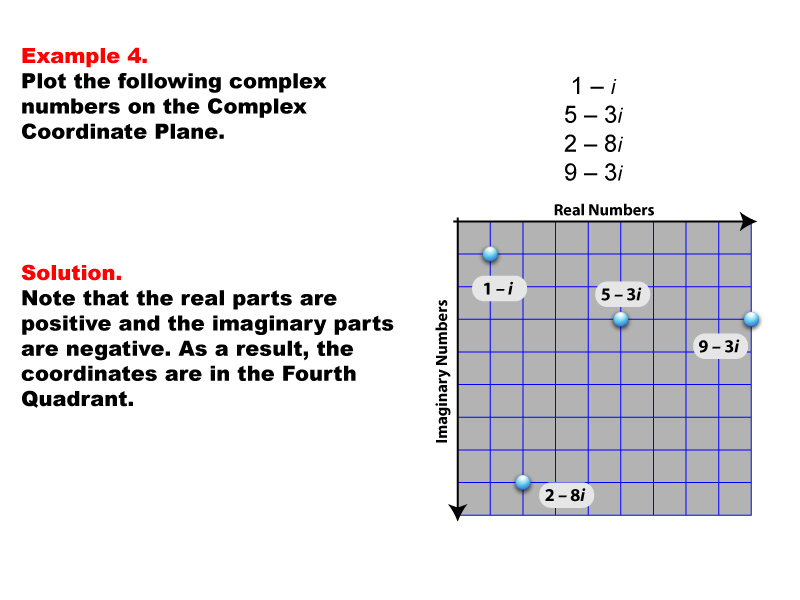
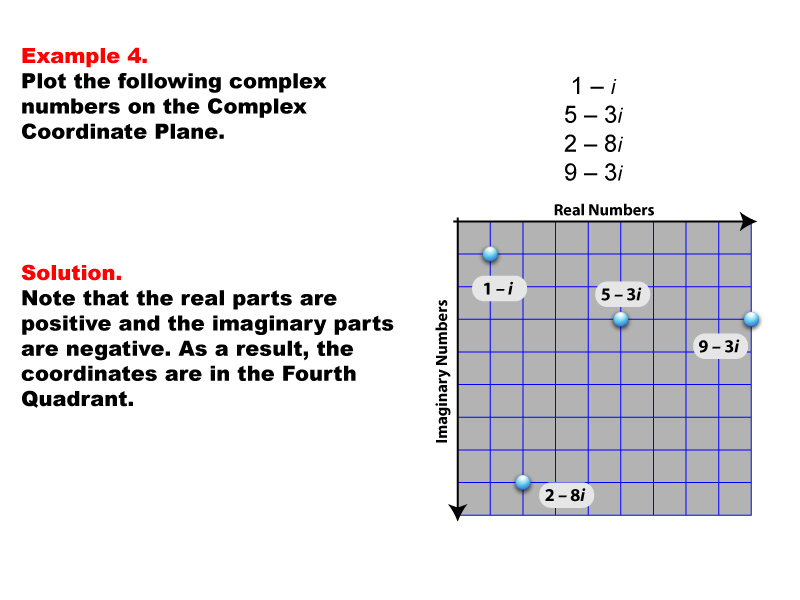
The image shows a complex plane with points plotted in the Fourth Quadrant. The complex numbers plotted are 1 - i, 5 - 3i, 2 - 8i, and 9 - 3i. The real parts are positive while the imaginary parts are negative. Example 4: The caption explains that because the real parts are positive and the imaginary parts are negative, all points lie in the Fourth Quadrant. The complex numbers plotted are: 1 - i, 5 - 3i, 2 - 8i, and 9 - 3i.
Complex numbers allow for a two-dimensional representation of numbers using real and imaginary components. Examples like these help students grasp how to plot complex coordinates, understanding both real and imaginary axes.
Seeing multiple worked-out examples is crucial for students to fully understand complex concepts like plotting complex numbers. Through varied examples, students can see consistent patterns and learn effective strategies.
Teacher’s Script: Imagine a number line that moves in two directions--right and up. Each complex number has a place on this plane, just like how we plot coordinates. Let's use this example to practice reading and plotting complex coordinates.
For a complete collection of math examples related to Complex Numbers click on this link: Math Examples: Complex Coordinates Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSN.CN.B.4, CCSS.MATH.CONTENT.HSN.CN.B.5, CCSS.MATH.CONTENT.HSN.CN.B.6 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Numerical and Algebraic Expressions |
| Copyright Year | 2020 |
| Keywords | complex numbers |