Display Title
Math Example--Complex Numbers--Complex Coordinates--Example 9
Display Title
Math Example--Complex Numbers--Complex Coordinates--Example 9
Topic
Complex Numbers
Description
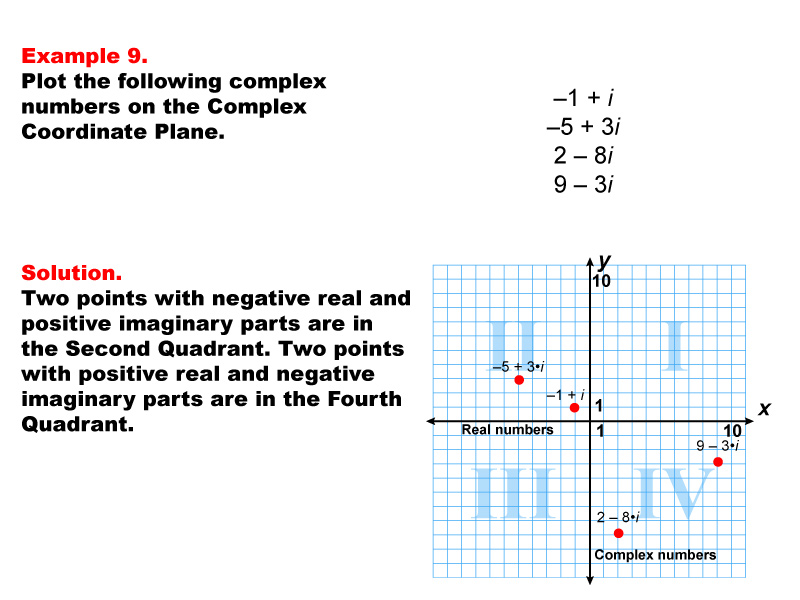
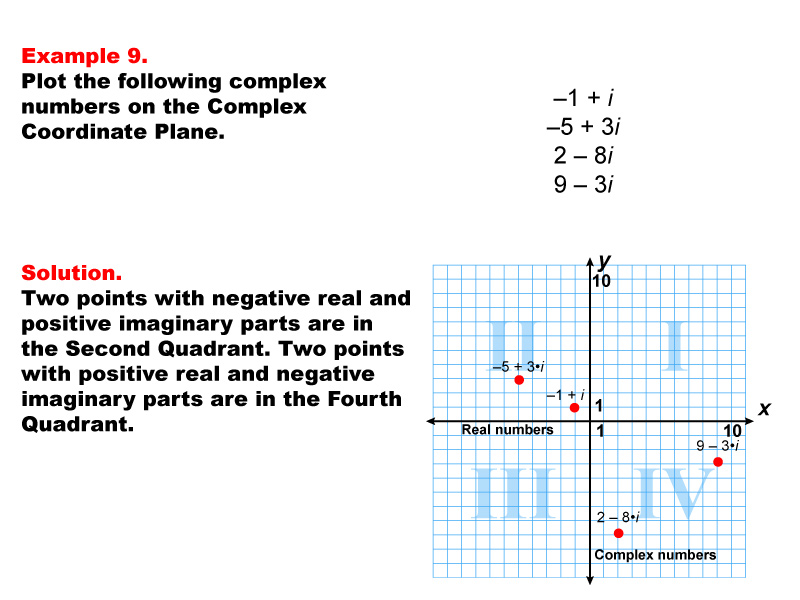
The image shows a complex coordinate plane with four plotted points: -1 + i, -5 + 3i, 2 - 8i, and 9 - 3i. The x-axis represents the real numbers, and the y-axis represents the imaginary numbers. Two points are in the second quadrant, and two points are in the fourth quadrant. Example 9: Plot the complex numbers -1 + i, -5 + 3i, 2 - 8i, and 9 - 3i on the complex coordinate plane. Two points with negative real and positive imaginary parts are in the second quadrant. Two points with positive real and negative imaginary parts are in the fourth quadrant.
Complex numbers allow for a two-dimensional representation of numbers using real and imaginary components. Examples like these help students grasp how to plot complex coordinates, understanding both real and imaginary axes.
Seeing multiple worked-out examples is crucial for students to fully understand complex concepts like plotting complex numbers. Through varied examples, students can see consistent patterns and learn effective strategies.
Teacher’s Script: The complex coordinate plane consists of two separate number lines.. Each complex number has a place on this plane, just like how we plot coordinates. Let's use this example to practice reading and plotting complex coordinates.
For a complete collection of math examples related to Complex Numbers click on this link: Math Examples: Complex Coordinates Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSN.CN.B.4, CCSS.MATH.CONTENT.HSN.CN.B.5, CCSS.MATH.CONTENT.HSN.CN.B.6 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Numerical and Algebraic Expressions |
| Copyright Year | 2020 |
| Keywords | complex numbers |