
Display Title
Math Example--Complex Numbers--Multiplying and Dividing Complex Numbers--Example 12
Display Title
Math Example--Complex Numbers--Multiplying and Dividing Complex Numbers--Example 12
Topic
Complex Numbers
Description
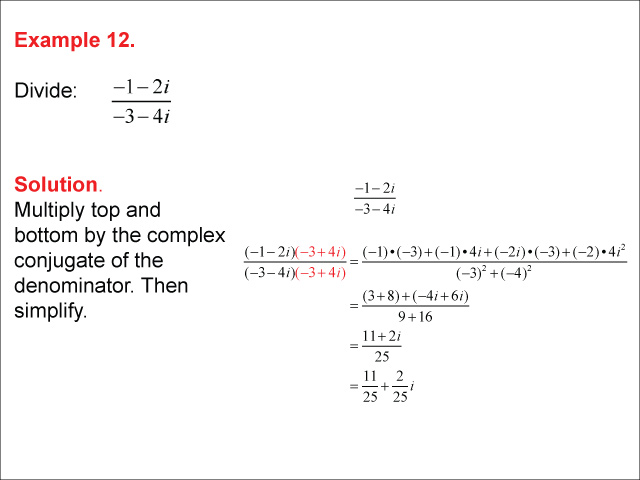
This image shows a worked example of dividing two complex numbers: (-1 - 2i) / (-3 - 4i). The solution involves multiplying both numerator and denominator by the complex conjugate of the denominator and simplifying.
Example 12: Dividing (-1 - 2i) by (-3 - 4i). The numerator and denominator are multiplied by (-3 + 4i), and the expression is simplified to (11/25) + (2/25)i.
This topic explores complex numbers, specifically multiplication and division involving imaginary components. The examples in this collection are designed to provide a step-by-step approach, showing how each part of the complex expressions is multiplied or divided to arrive at a simplified result. By breaking down the operations, these examples help demystify complex arithmetic and reinforce the rules of operations for complex numbers.
Seeing multiple worked-out examples allows students to understand the structure of complex arithmetic, enabling them to apply the same process to similar problems. This repetition builds confidence and ensures students recognize patterns and solutions that can be generalized across different types of complex number operations.
Teacher’s Script: Let's look at this example together. Notice how each step builds on the previous one. Take a close look at how we handle both the real and imaginary parts separately before combining them for the final answer. By understanding each part of the solution, you'll be ready to solve similar problems on your own.
For a complete collection of math examples related to Complex Numbers click on this link: Math Examples: Multiplying and Dividing Complex Numbers Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSN.CN.A.1, CCSS.MATH.CONTENT.HSN.CN.A.2, CCSS.MATH.CONTENT.HSN.CN.A.3 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Expressions, Equations, and Inequalities • Numerical and Algebraic Expressions |
| Copyright Year | 2020 |
| Keywords | complex numbers, multiplying and dividing complex numbers |