Display Title
Math Example--Coordinate Geometry--Coordinate Systems: Example 18
Display Title
Math Example--Coordinate Geometry--Coordinate Systems: Example 18
Topic
Geometry
Description
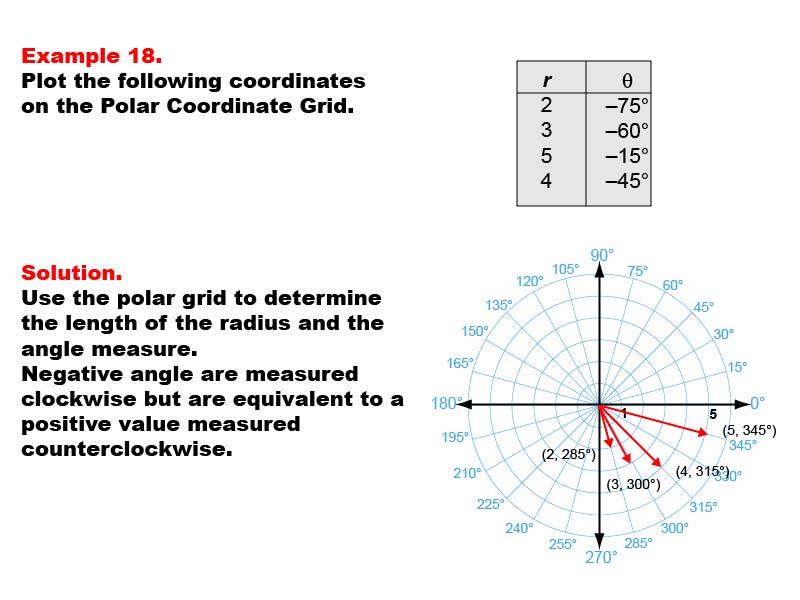
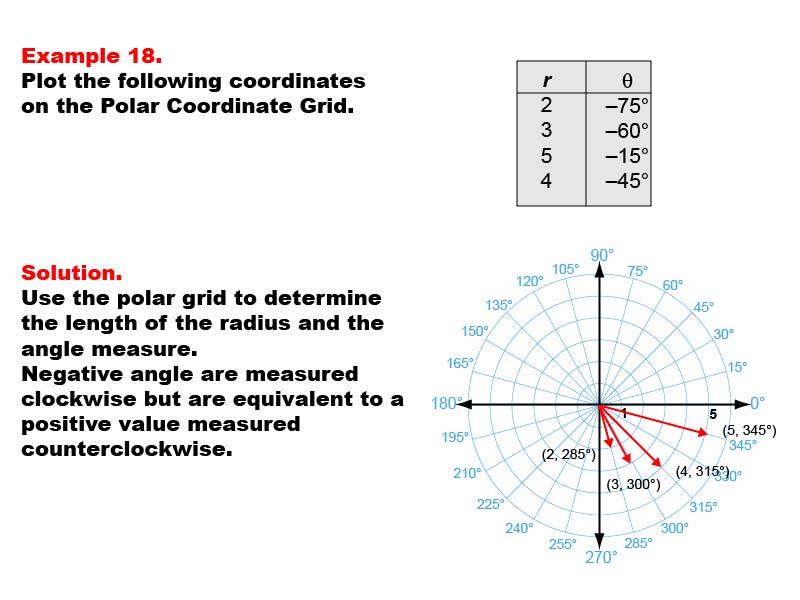
This example demonstrates plotting coordinates on a polar coordinate grid using negative angles. The image shows four points with their polar coordinates (r, θ): (2, -75°), (3, -60°), (5, -15°), and (4, -45°). The grid displays angles in both positive and negative directions, illustrating how negative angles are equivalent to positive ones.
Understanding negative angles in polar coordinates is crucial for a comprehensive grasp of this system. This example helps students visualize how negative angles are measured clockwise, contrary to the standard counterclockwise measurement of positive angles.
By providing multiple examples with negative angles, students can develop a clear understanding of the relationship between positive and negative angles in polar coordinates. This reinforces the concept of angle measure and its impact on point location.
Teacher's Script: Look closely at how these points are plotted using negative angles. Notice that measuring clockwise gives us the same point as measuring counterclockwise with a positive angle. Can you determine the positive angle equivalent for each of these points? Let's discuss why understanding negative angles is important in polar coordinates and how it relates to real-world applications.
For a complete collection of math examples related to Coordinate Systems click on this link: Math Examples: Coordinate Systems Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.NS.C.8 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Geometry • Coordinate Geometry • Coordinate Systems |
| Copyright Year | 2013 |
| Keywords | coordinate geometry, coordinate system, coordinate systems |