
Display Title
Math Example--Coordinate Geometry--Coordinate Systems: Example 30
Display Title
Math Example--Coordinate Geometry--Coordinate Systems: Example 30
Topic
Geometry
Description
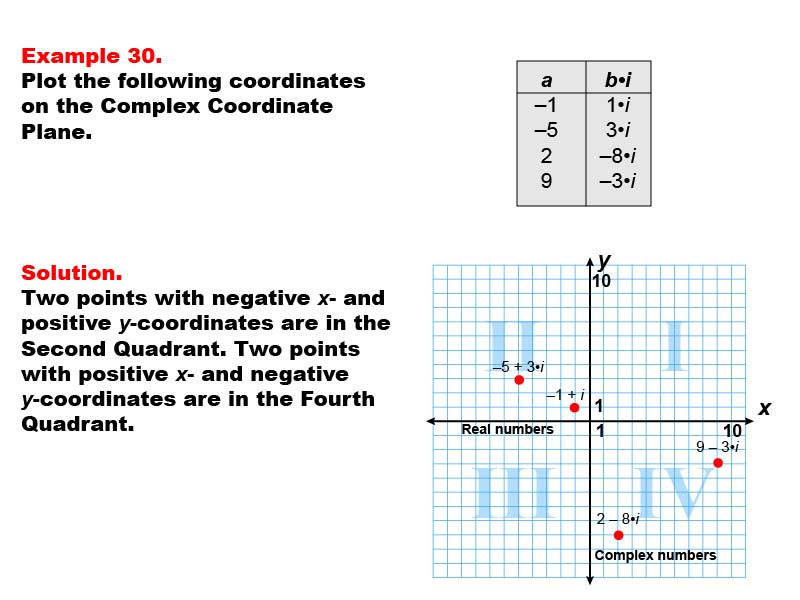
This example illustrates plotting complex numbers in the second and fourth quadrants of the complex plane. The image shows four points: (-1, i), (-5, 3i), (2, -8i), and (9, -3i). These points represent complex numbers with varying real and imaginary parts.
Understanding how to plot complex numbers across different quadrants is crucial for a comprehensive grasp of complex number theory. This example helps students visualize how complex numbers with different combinations of positive and negative real and imaginary parts are positioned on the complex plane.
By providing examples that span diagonally opposite quadrants, students can develop a deeper understanding of the relationship between complex numbers and their geometric representation. This approach reinforces the concept of how the signs of real and imaginary parts determine a point's quadrant.
Teacher's Script: Examine how these complex numbers are plotted in the second and fourth quadrants. Notice that the points in the second quadrant have negative real parts and positive imaginary parts, while those in the fourth quadrant have positive real parts and negative imaginary parts. Can you explain the relationship between these diagonally opposite quadrants? Let's discuss how this relates to our understanding of both complex numbers and coordinate geometry.
For a complete collection of math examples related to Coordinate Systems click on this link: Math Examples: Coordinate Systems Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.NS.C.8 |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Geometry • Coordinate Geometry • Coordinate Systems |
| Copyright Year | 2013 |
| Keywords | coordinate geometry, coordinate system, coordinate systems |