Display Title
Math Example--Coordinate Geometry--Distance Formula: Example 6
Display Title
Math Example--Coordinate Geometry--Distance Formula: Example 6
Topic
Geometry
Description
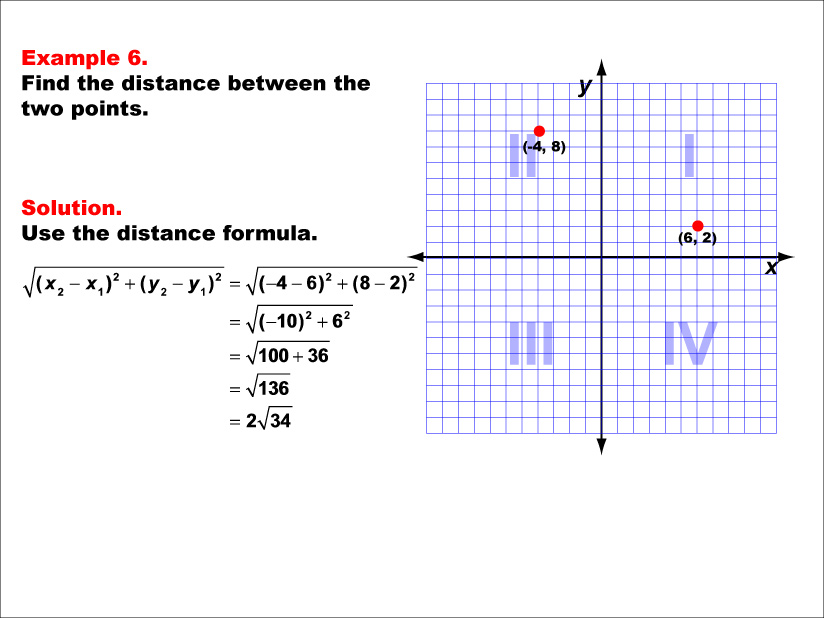
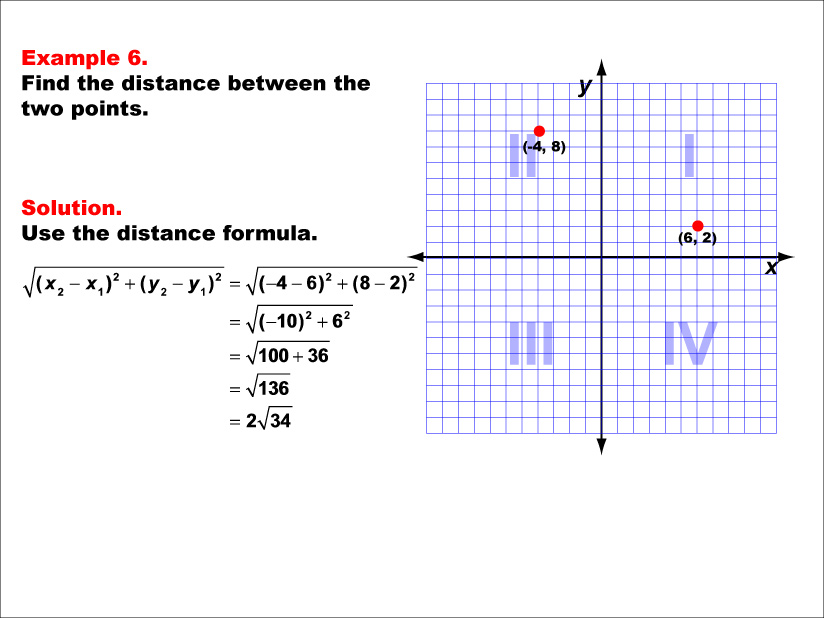
This example illustrates the use of the distance formula to calculate the distance between two points on a coordinate plane. The points (-4, 8) and (6, 2) are plotted on a graph, and the distance between them is determined using the formula: √((-4 - 6)2 + (8 - 2)2) = 2 √(34).
The distance formula is a key concept in coordinate geometry, allowing students to find the length of a line segment given the coordinates of its endpoints. This collection of examples showcases various applications of the formula, helping students understand its versatility in solving geometric problems across different scenarios, including points in different quadrants and with both positive and negative coordinates.
Providing multiple worked-out examples is essential for students to fully comprehend the distance formula and its applications. By observing the formula in action across different contexts, students can develop a more robust understanding of how to apply it effectively. This approach also reinforces the step-by-step process of using the formula, enabling students to tackle similar problems with confidence.
Teacher Script: "In this sixth example, we're working with the points (-4, 8) and (6, 2). Notice how these points are in different quadrants and have both positive and negative coordinates. As we apply the distance formula, pay attention to how we handle the negative numbers. Remember, when we square a negative number, it becomes positive. Can you explain why this is important in our distance calculations?"
For a complete collection of math examples related to Geometry click on this link: Math Examples: Distance Formula Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.G.B.8, CCSS.MATH.CONTENT.HSG.GPE.B.4, CCSS.MATH.CONTENT.HSG.GPE.B.7 |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Geometry • Coordinate Geometry • The Distance Formula |
| Copyright Year | 2013 |
| Keywords | pythagorean theorem, coordinate, square root, radical, distance formula |