Display Title
Math Example--Coordinate Geometry--Slope Formula: Example 1
Display Title
Math Example--Coordinate Geometry--Slope Formula: Example 1
Topic
Slope Formula
Description
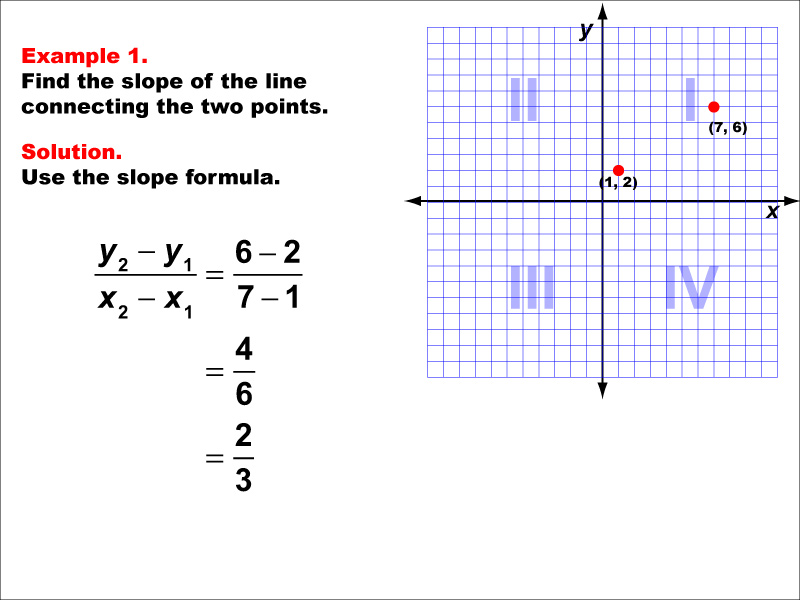
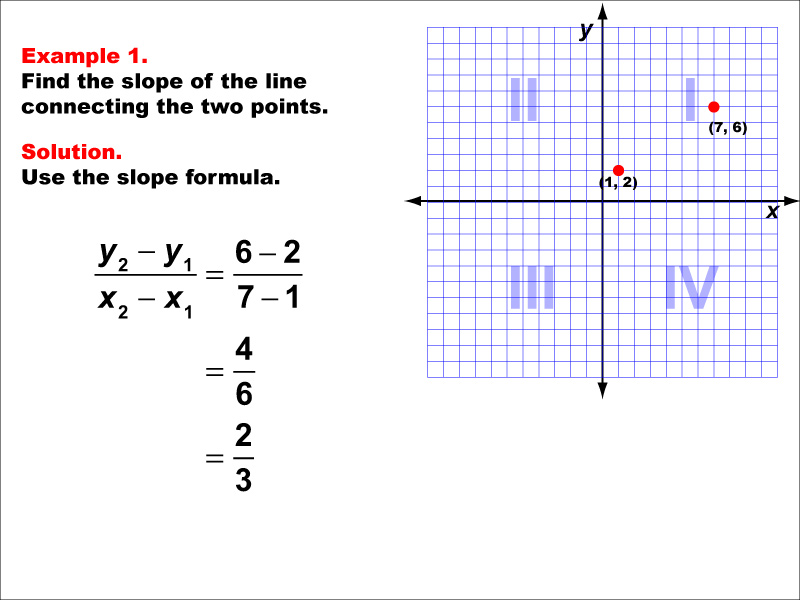
This example demonstrates how to calculate the slope of a line connecting two points on a coordinate grid. The points (1, 2) and (7, 6) are plotted, and the slope formula is applied to find the slope between them. The calculation shows that the slope is (6 - 2) / (7 - 1) = 4 / 6 = 2 / 3.
The slope formula is a fundamental concept in coordinate geometry, allowing us to determine the steepness and direction of a line. It's essential for understanding linear relationships and is widely used in various mathematical and real-world applications.
By providing multiple examples like this one, students can see how the slope formula is applied in different scenarios. This helps reinforce the concept and improves their ability to calculate slopes accurately in various situations.
Teacher's Script: Let's examine this example closely. Notice how we use the coordinates of the two points to calculate the slope. Remember, the slope tells us how much the line rises or falls for each unit it moves horizontally. In this case, for every 3 units we move to the right, the line rises by 2 units.
For a complete collection of math examples related to Slope Formula click on this link: Math Examples: Slope Formula Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.EE.B.5, CCSS.MATH.CONTENT.7.RP.A.2.D |
|---|---|
| Grade Range | 6 - 8 |
| Curriculum Nodes |
Algebra • Linear Functions and Equations • Slope |
| Copyright Year | 2013 |
| Keywords | slope, rise over run, slope formula |