Display Title
Math Example--Exponential Concepts--Exponential Functions in Tabular and Graph Form: Example 40
Display Title
Math Example--Exponential Concepts--Exponential Functions in Tabular and Graph Form: Example 40
Topic
Exponential Functions
Description
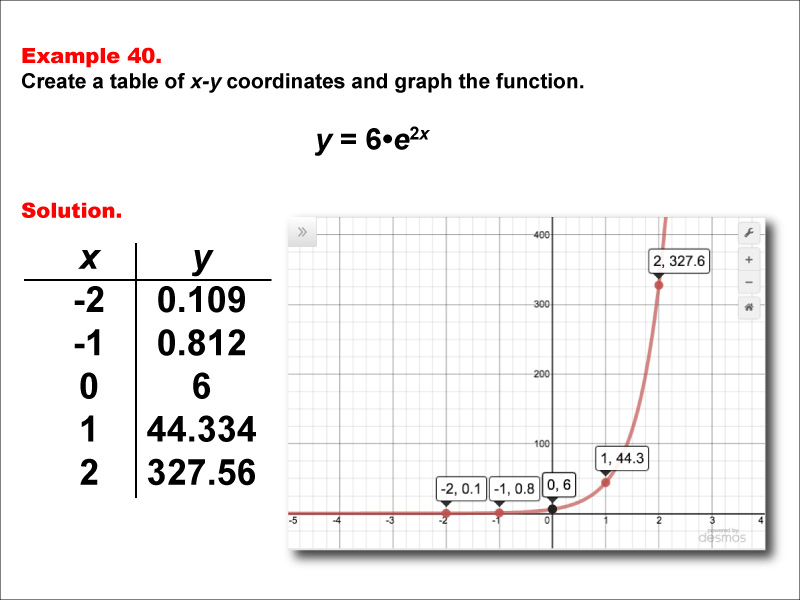
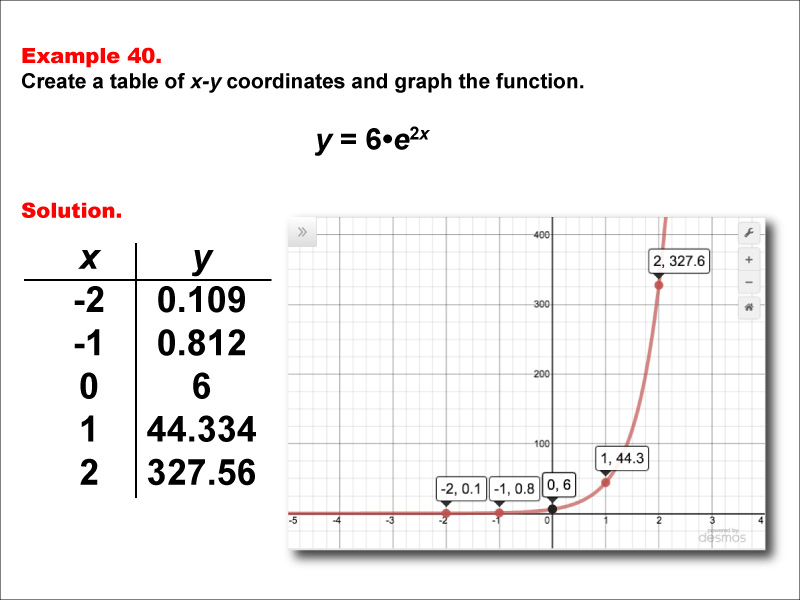
This math example illustrates the exponential function y = 6 * e2x through both a table of values and a graph. The table presents x-y coordinates for x values from -2 to 2, demonstrating the rapid growth of this function as x increases. The accompanying graph provides a visual representation of this exponential growth function, highlighting its steep curve and behavior.
Exponential functions with coefficients and exponents are fundamental in mathematics and have numerous real-world applications, such as modeling compound interest, population growth, and certain physical phenomena. This collection of examples aids in teaching the topic by offering students diverse representations of exponential functions, enabling them to observe how different parameters influence the function's behavior, particularly when dealing with coefficients greater than 1 and exponents containing variables.
The importance of multiple worked-out examples in understanding complex exponential functions cannot be overstated. Each example in this set showcases a unique form of the exponential function, helping students identify patterns and comprehend how alterations in the coefficient and exponent affect the function's graph and values. This variety of examples strengthens the concept and assists students in developing a more intuitive grasp of exponential behavior, especially in cases where the growth rate is significantly accelerated.
Teacher Script: "Let's examine the function y = 6 * e2x. Notice how rapidly the y-values increase as x becomes positive. The coefficient of 6 shifts the y-intercept up to (0, 6), while the factor of 2 in the exponent dramatically increases the growth rate compared to a standard exponential function. Compare this graph to our previous examples with ex. How does the steeper curve affect the function's behavior? Can you think of any real-world scenarios where we might encounter such rapid exponential growth?"
For a complete collection of math examples related to Exponential Functions click on this link: Math Examples: Exponential Functions Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7.E, CCSS.Math.CONTENT.HSF.LE.A.2, CCSS.MATH.CONTENT.HSF.LE.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Graphs of Exponential and Logarithmic Functions |
| Copyright Year | 2015 |
| Keywords | function, graphs of exponential functions, exponential function tables |