Display Title
Math Example--Exponential Concepts--Exponential Functions in Tabular and Graph Form: Example 47
Display Title
Math Example--Exponential Concepts--Exponential Functions in Tabular and Graph Form: Example 47
Topic
Exponential Functions
Description
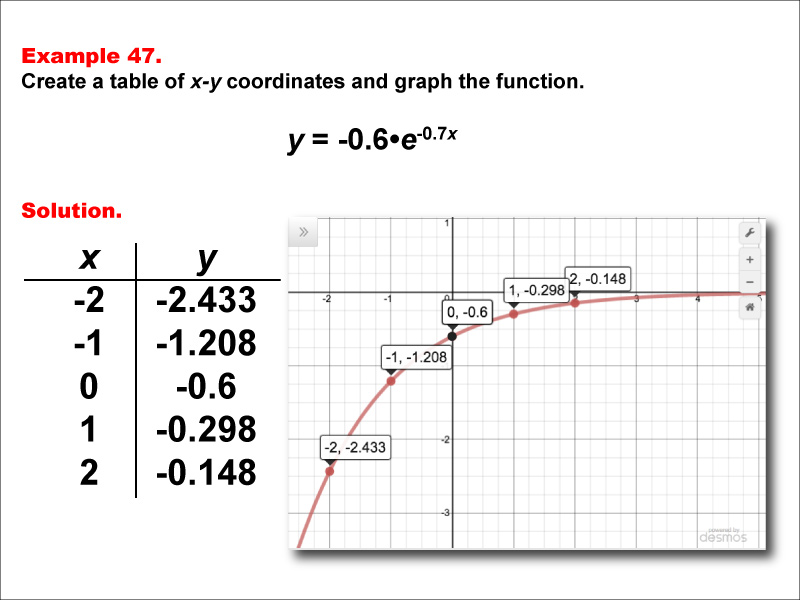
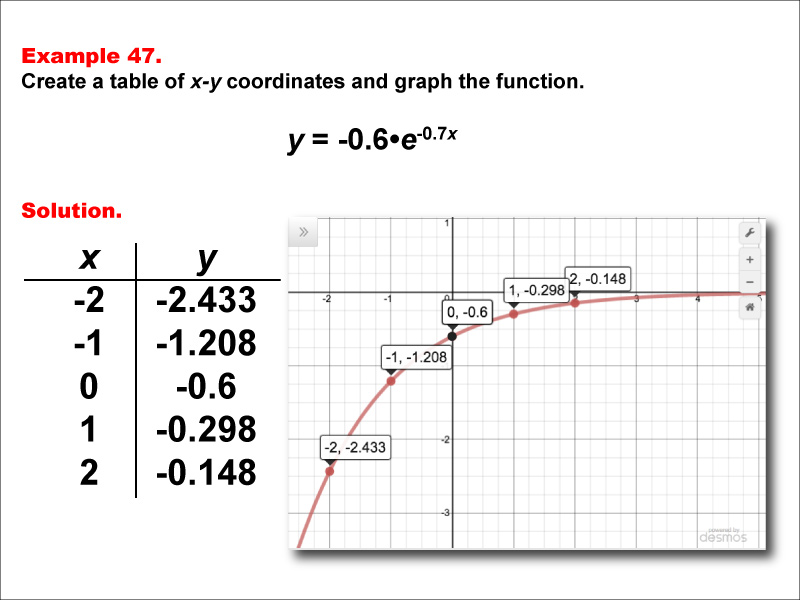
This example illustrates the exponential function y = -0.6 * e-0.7x through a graph and table of x-y coordinates. The table provides points (-2, -2.433), (-1, -1.208), (0, -0.6), (1, -0.298), and (2, -0.148), which are plotted on the accompanying graph. Students are challenged to create this table and graph to visualize the function's behavior.
Exponential functions with negative coefficients and exponents, like this one, demonstrate interesting decay processes with a reflection across the x-axis. This collection of examples showcases various forms of exponential functions, including those with different bases, coefficients, and exponents. By exploring these variations, students can better understand how changes in the equation affect the shape and behavior of the graph, reinforcing their comprehension of exponential functions and their real-world applications.
Providing multiple worked-out examples is essential for students to fully grasp mathematical concepts like exponential functions. It enables them to identify patterns, make predictions, and build confidence in interpreting and creating graphs. Engaging with diverse problems helps develop a comprehensive, enduring understanding of these crucial mathematical ideas, preparing students for more advanced topics and real-world problem-solving scenarios.
Teacher Script: As you analyze this graph and table, consider how the negative coefficient and negative exponent influence the shape and direction of the curve. How does this function compare to other exponential functions we've studied? Discuss with your peers any trends you observe in the y-values as x increases. Before plotting the points, what predictions can you make about the graph's behavior and its long-term trend?
For a complete collection of math examples related to Exponential Functions click on this link: Math Examples: Exponential Functions Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7.E, CCSS.Math.CONTENT.HSF.LE.A.2, CCSS.MATH.CONTENT.HSF.LE.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Graphs of Exponential and Logarithmic Functions |
| Copyright Year | 2015 |
| Keywords | function, graphs of exponential functions, exponential function tables |