Display Title
Math Example--Exponential Concepts--Laws of Exponents: Example 3
Display Title
Math Example--Exponential Concepts--Laws of Exponents: Example 3
Topic
Exponents
Description
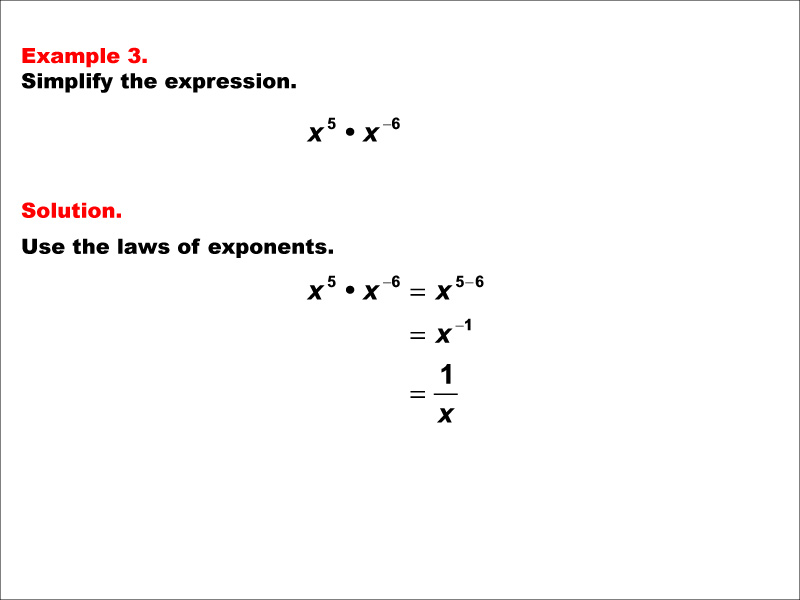
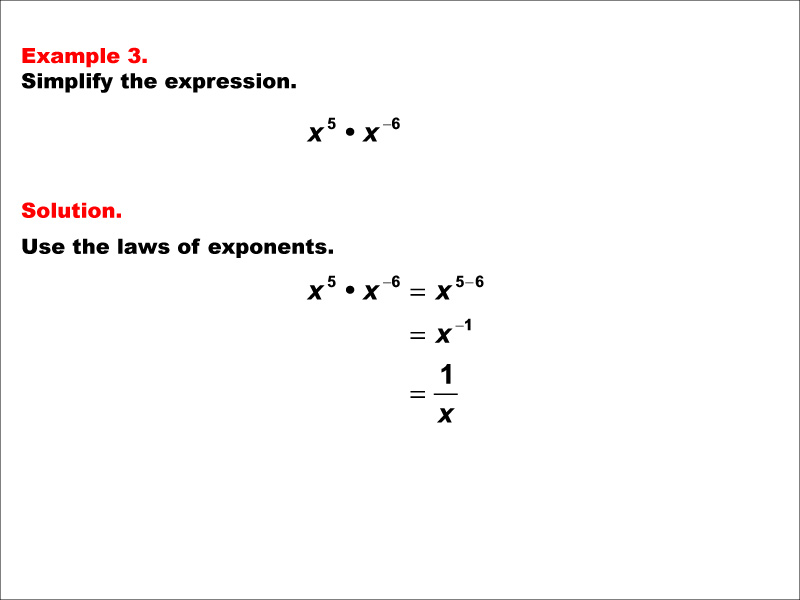
This example demonstrates the simplification of an expression resulting in a negative exponent. The problem involves simplifying x5 * x(-6), which results in x(-1) or 1/x. This showcases how positive and negative exponents interact and how the result can be expressed as a fraction.
Understanding how to handle expressions that result in negative exponents is crucial in algebra and higher mathematics. These examples help students grasp the concept that a negative exponent indicates the reciprocal of a number raised to the positive of that exponent. By presenting various scenarios, students learn to apply these rules confidently in different mathematical contexts.
Exposure to multiple worked-out examples is vital for students to fully comprehend the concept of exponents, especially when dealing with negative results. Each example builds upon previous knowledge, reinforcing core principles while introducing new complexities. This approach helps students develop a deeper understanding and the ability to tackle more challenging problems involving exponents.
Teacher's Script: Let's look at an interesting example: x5 * x(-6). Remember, we add the exponents when multiplying terms with the same base. So, what do we get? x(-1), right? But what does x(-1) mean? It's actually equal to 1/x. This is a key concept: a negative exponent means we take the reciprocal of the base raised to the positive of that exponent. So whenever you see a negative exponent, think 'reciprocal'!
For a complete collection of math examples related to Exponents click on this link: Math Examples: Laws of Exponents Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.EE.A.1, CCSS.MATH.CONTENT.HSA.SSE.B.3.C |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Laws of Exponents |
| Copyright Year | 2013 |
| Keywords | exponents, laws of exponents |