Display Title
Math Example--Function Concepts--Building Functions: Example 12
Display Title
Math Example--Function Concepts--Building Functions: Example 12
Topic
Arithmetic
Description
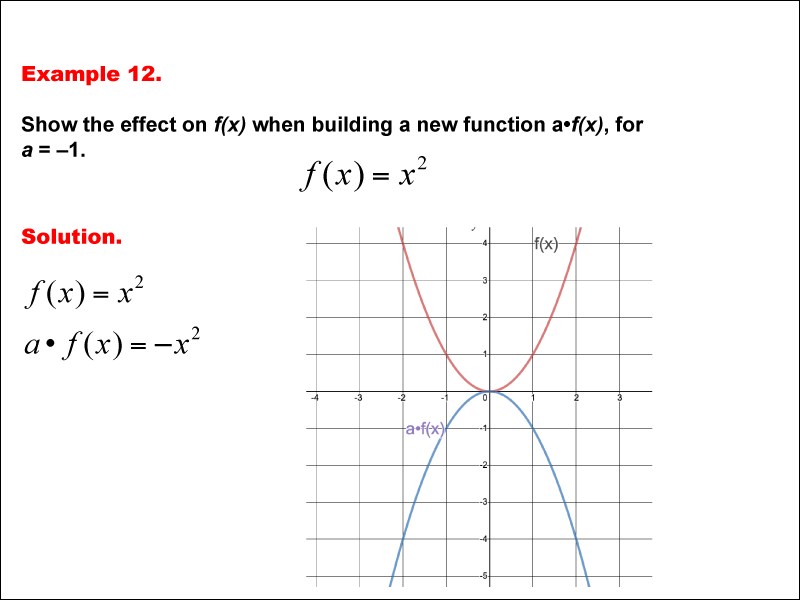
The problem examines the effect on the function f(x) = x2 when it is scaled by a constant a. The specific case here is a = -1. The goal is to describe the transformation caused by the scaling. This example shows the effect on the function when multiplying f(x) by a constant a reflects the graph across the x-axis if a < 0. for a = -1, the function becomes a * f(x) = -x2. this results in a reflection of the original graph (in red) to the inverted graph (in blue).
The topic of functions is fundamental in mathematics. By observing how different transformations—shifts, reflections, and scalings—impact the function, students can better understand the concept of functional relationships. These examples provide a hands-on approach to dissecting how functions behave under various operations, making abstract concepts tangible.
Seeing multiple worked-out examples helps students grasp concepts deeply. It allows them to recognize patterns, test their hypotheses, and correct misconceptions, building a strong foundation in function operations.
Teacher’s Script: Look at the function presented here, where multiplying f(x) by a constant a reflects the graph across the x-axis if a < 0. for a = -1, the function becomes a * f(x) = -x2. this results in a reflection of the original graph (in red) to the inverted graph (in blue). Can you observe how the transformation changes the graph? Notice how this operation directly affects the function's behavior. Try applying a similar transformation to a different function to see if the pattern holds.
For a complete collection of math examples related to Functions click on this link: Math Examples: Building Functions Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.B.3 |
|---|---|
| Grade Range | 8 - 10 |
| Curriculum Nodes |
Algebra • Functions and Relations • Relations and Functions |
| Copyright Year | 2020 |
| Keywords | building functions |