Display Title
Math Example--Function Concepts--Functions and Their Inverses: Example 7
Display Title
Math Example--Function Concepts--Functions and Their Inverses: Example 7
Topic
Functions
Description
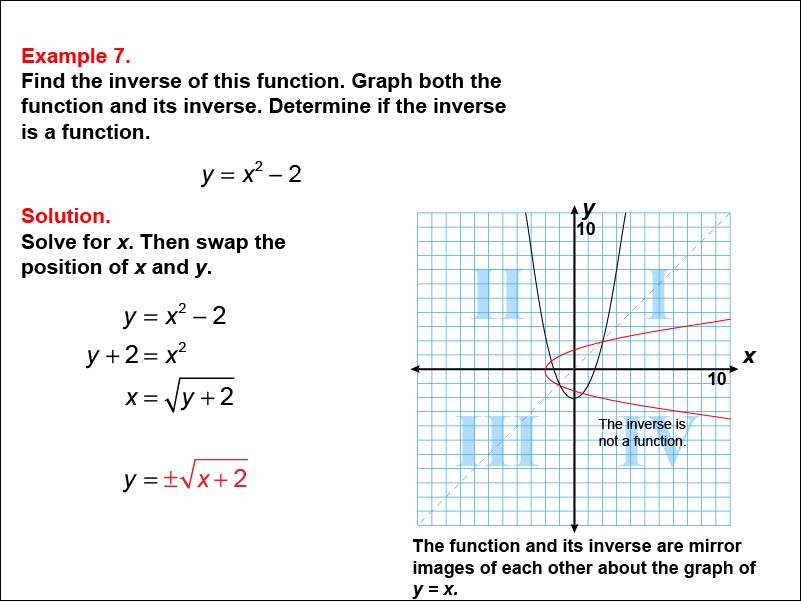
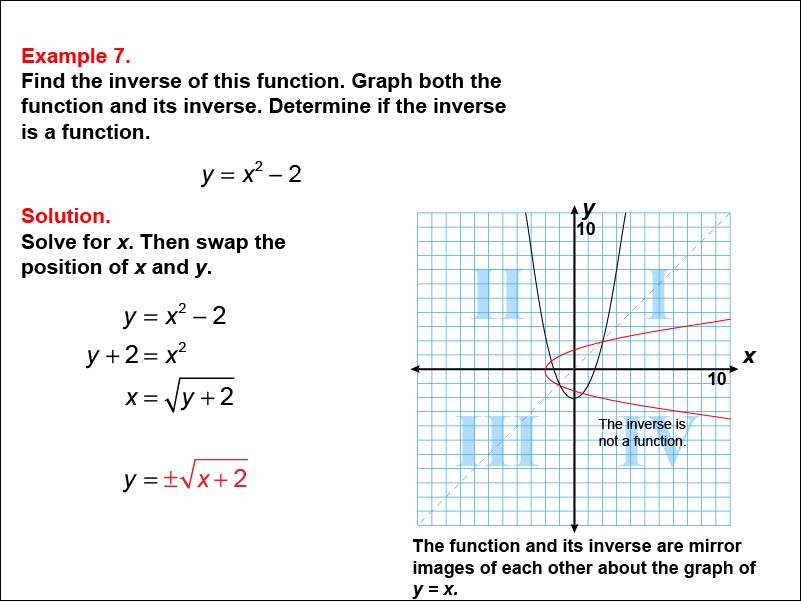
This example explores finding the inverse of the function y = x2 - 2. The graph displays an upward-opening parabola and its inverse, mirrored over the line y = x. Students are challenged to determine the inverse function by solving for x and then swapping x and y.
The study of inverse functions is a fundamental concept in mathematics, allowing students to explore the relationships between functions and their inverses. This collection of examples provides a comprehensive look at various types of functions and their inverses, helping students understand the concept from different perspectives.
Exposure to multiple worked-out examples is crucial for students to fully grasp the concept of inverse functions. Each example presents a unique scenario or function type, enabling students to recognize patterns and develop problem-solving strategies applicable to a wide range of mathematical situations.
For this example, focus on how we find the inverse of y = x2 - 2. The result is y = ±√(x + 2). Note that the inverse is not a function, as it fails the vertical line test. Understanding this process will help you solve similar problems and recognize when an inverse is or is not a function.
For a complete collection of math examples related to Inverse Functions click on this link: Math Examples: Inverse Functions Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.B.4, CCSS.MATH.CONTENT.HSF.BF.B.4.A, CCSS.MATH.CONTENT.HSF.BF.B.4.B, CCSS.MATH.CONTENT.HSF.BF.B.4.C, CCSS.MATH.CONTENT.HSF.BF.B.4.D |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Functions and Relations • Functions and Their Inverses |
| Copyright Year | 2013 |
| Keywords | functions, inverses |