
Display Title
Math Example: Graphs of Logarithmic Functions: Example 21
Display Title
Math Example: Graphs of Logarithmic Functions: Example 21
Topic
Logarithmic Functions
Description
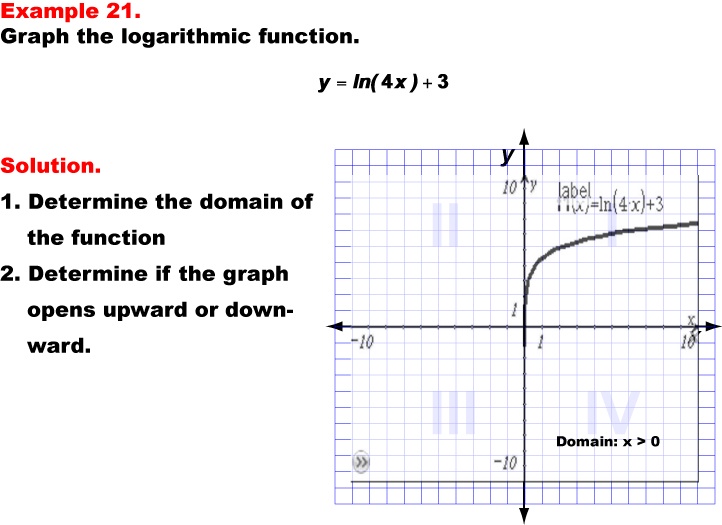
This example illustrates the graph of the natural logarithmic function y = ln(4x) + 3. The graph opens upward, curving from left to right, demonstrating how the combination of multiplication inside the logarithm and addition outside affects the function's behavior. The domain of this function is x > 0, as the expression inside the natural logarithm must be positive.
Natural logarithmic functions with various transformations are crucial in many scientific and mathematical applications, particularly in calculus and differential equations. They are often used to model complex growth and decay processes in fields such as biology, physics, and economics. This collection of examples helps teach logarithmic functions by presenting a range of equations with different parameters, allowing students to visualize and understand how these changes impact the graph's shape, position, and range.
Exploring multiple worked-out examples is vital for students to develop a comprehensive understanding of logarithmic functions and their transformations. Each example builds upon previous knowledge, introducing new concepts and variations. This approach helps students recognize patterns, make connections between different forms of logarithmic equations, and develop problem-solving skills that can be applied to more complex scenarios involving exponential and logarithmic relationships.
Teacher's Script: Let's examine our twenty-first example, y = ln(4x) + 3. How does this graph compare to the basic natural logarithm function we saw earlier? Notice the similar shape, but can you spot any differences in its position? The domain remains x > 0, but why? Consider how the multiplication by 4 inside the logarithm affects the graph's horizontal position. Then, think about how the addition of 3 outside the logarithm impacts the vertical position. Can you predict where this graph will intersect the y-axis? How does this transformation impact the overall behavior of the function?
For a complete collection of math examples related to Logarithmic Functions click on this link: Math Examples: Graphs of Logarithmic Functions Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.IF.C.8.B, CCSS.MATH.CONTENT.HSF.BF.B.5, CCSS.MATH.CONTENT.HSF.IF.C.7.E, CCSS.Math.CONTENT.HSF.LE.A.2, CCSS.MATH.CONTENT.HSF.LE.A.3, CCSS.MATH.CONTENT.HSF.LE.A.4, CCSS.MATH.CONTENT.HSF.LE.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Graphs of Exponential and Logarithmic Functions |
| Copyright Year | 2013 |
| Keywords | logarithmic functions, graphs |