Display Title
Math Example: Laws of Logarithms: Example 02
Display Title
Math Example: Laws of Logarithms: Example 02
Topic
Logarithms
Description
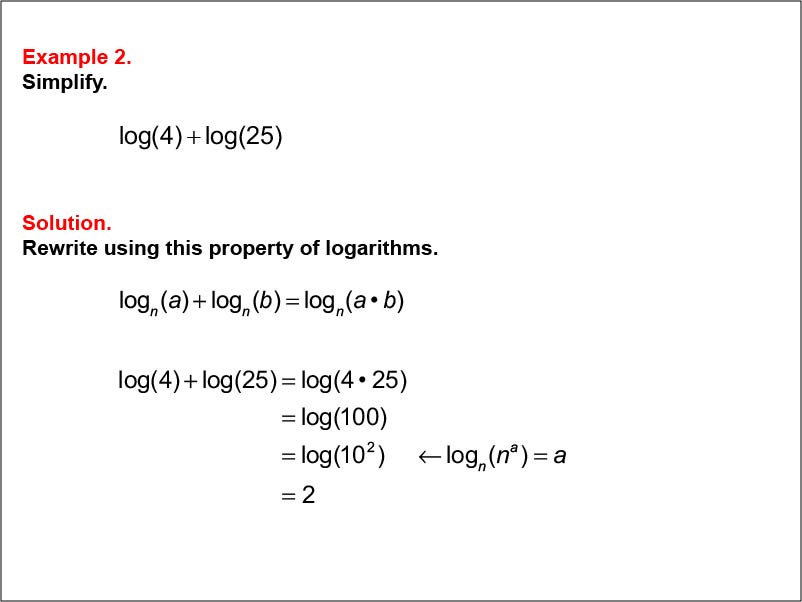
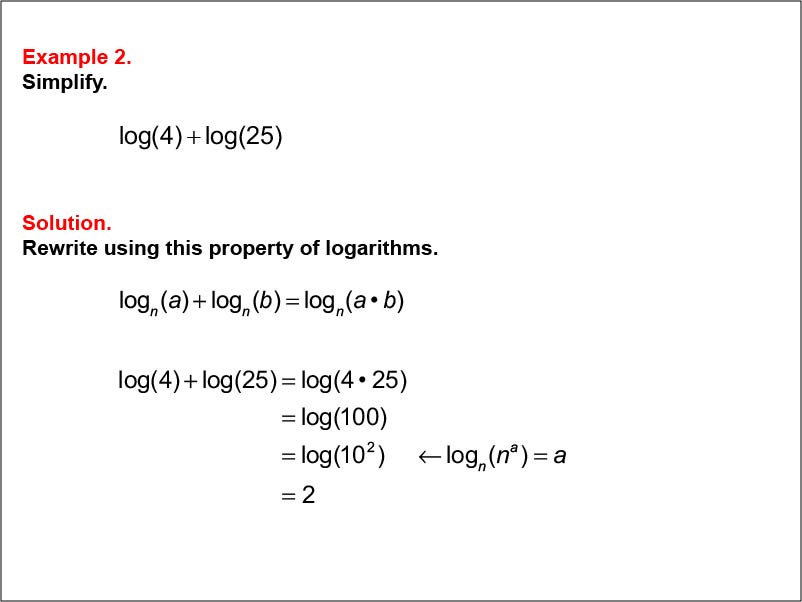
This example illustrates the simplification of log of 4 plus log of 25 using logarithm properties. The solution applies the property that the sum of logarithms equals the logarithm of the product of their arguments. Here, log(4) + log(25) simplifies to log(100), which further simplifies to log(102), resulting in 2.
Understanding the Laws of Logarithms is essential for manipulating and simplifying logarithmic expressions. These examples provide students with practical applications of these laws, helping them develop problem-solving skills and mathematical intuition. By working through various scenarios, students learn to recognize patterns and apply appropriate strategies.
Exposure to multiple worked-out examples is crucial for students to fully comprehend logarithmic concepts. Each example presents a unique situation, allowing students to see how the laws of logarithms can be applied in different contexts. This repetition reinforces understanding and builds confidence in tackling complex problems.
Teacher's Script: As we work through this example, pay attention to how we combine logarithms with the same base. This property is fundamental in simplifying logarithmic expressions. Can you think of a real-world scenario where this type of calculation might be useful?
For a complete collection of math examples related to Logarithms click on this link: Math Examples: Laws of Logarithms Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Laws of Logarithms |
| Copyright Year | 2013 |
| Keywords | logarithms, laws of logarithms |