Display Title
Math Example: Laws of Logarithms: Example 08
Display Title
Math Example: Laws of Logarithms: Example 08
Topic
Logarithms
Description
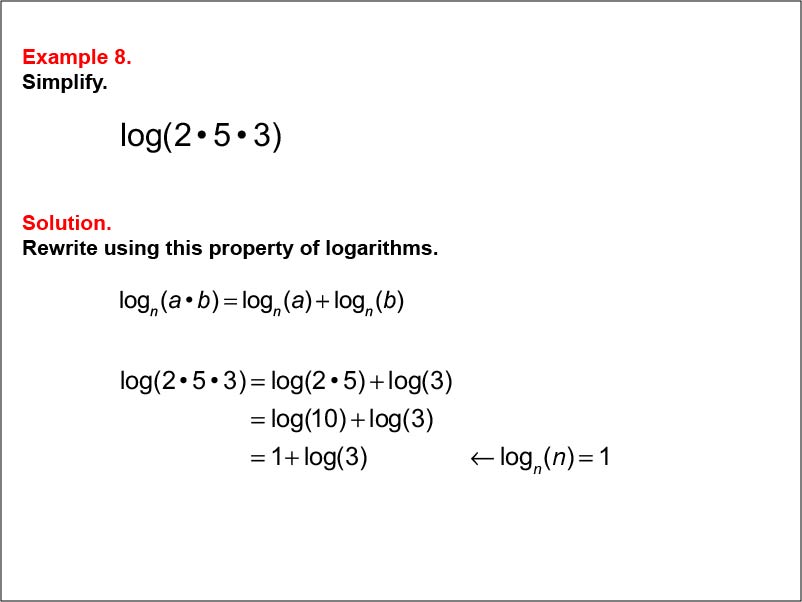
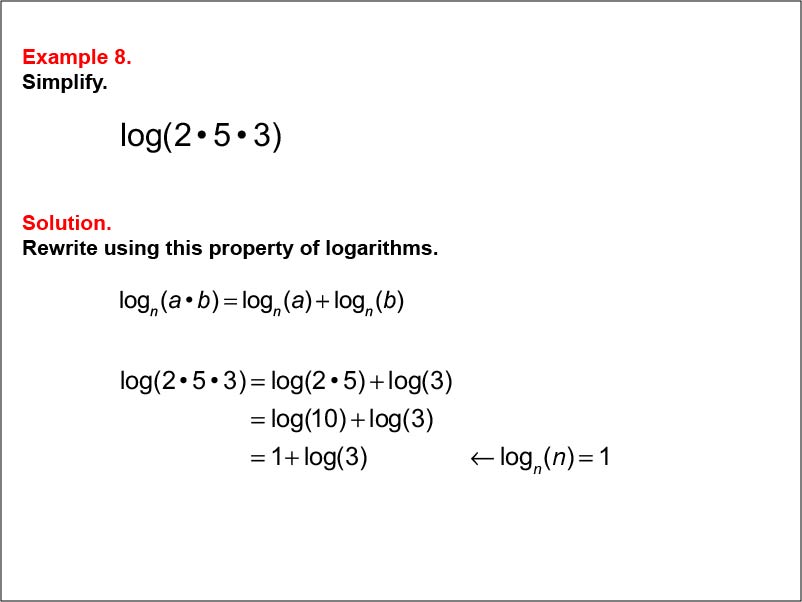
This example demonstrates the simplification of a logarithmic expression using the product property of logarithms. The problem involves simplifying log(2 * 5 * 3) by applying the rule logn(a * b) = logn(a) + logn(b). The solution shows that this expression can be rewritten as log(10) + log(3), which further simplifies to 1 + log(3).
The topic of logarithms is fundamental in advanced mathematics, particularly in algebra and calculus. These examples help teach logarithmic properties by providing step-by-step demonstrations of how to manipulate and simplify logarithmic expressions. By working through various scenarios, students can gain a deeper understanding of the rules governing logarithms and how to apply them in different contexts.
It's crucial for students to see multiple worked-out examples to fully grasp logarithmic concepts. Each example reinforces the principles while introducing slight variations, helping students recognize patterns and develop problem-solving strategies. This approach builds confidence and proficiency in handling logarithmic expressions.
Teacher's Script: Alright, class, let's look at this example of simplifying a logarithmic expression. We're dealing with log(2 * 5 * 3). Remember, when we have a product inside a logarithm, we can split it into the sum of individual logarithms. So, we'll rewrite this as log(2) + log(5) + log(3). Now, what's log(2 * 5)? That's log(10), which equals 1. So our final answer is 1 + log(3). See how we used the product property to break down a complex expression into something simpler?
For a complete collection of math examples related to Logarithms click on this link: Math Examples: Laws of Logarithms Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Laws of Logarithms |
| Copyright Year | 2013 |
| Keywords | logarithms, laws of logarithms |