Display Title
Math Example: Laws of Logarithms: Example 21
Display Title
Math Example: Laws of Logarithms: Example 21

Topic
Logarithms
Description
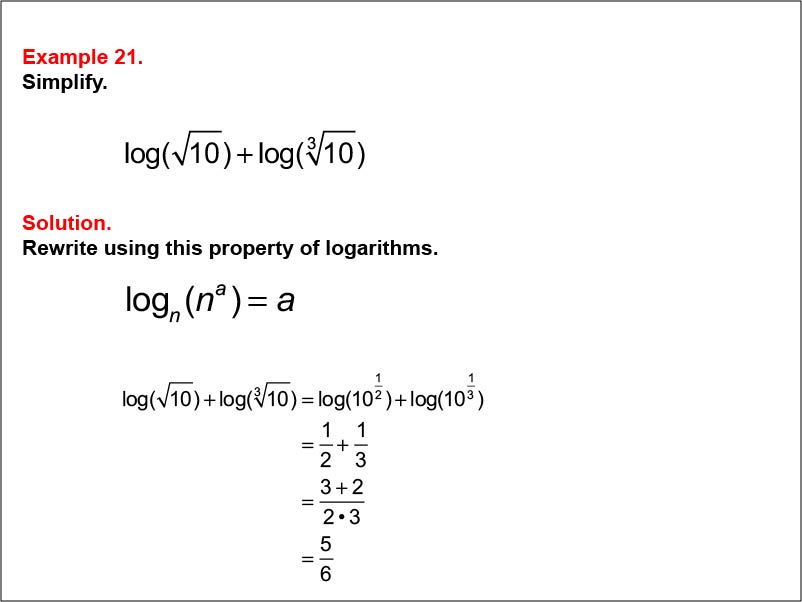
This example demonstrates the simplification of logarithmic expressions involving √(10) and cube root of 10. The solution applies the property log(na) = a * log(n) to rewrite log(√(10)) as (1/2)log(10) and log(3√10) as (1/3)log(10). These are then combined using the addition property of logarithms, resulting in log(10(1/2 + 1/3)) = log(10(5/6)).
Logarithms involving roots are an important topic in advanced algebra and precalculus. These examples help students understand how to manipulate logarithmic expressions with fractional exponents. By working through such scenarios, students learn to apply logarithmic rules effectively and gain confidence in solving more intricate problems involving logarithms and radical expressions.
Providing multiple worked-out examples is crucial for students to fully grasp logarithmic concepts, especially when dealing with roots and fractional exponents. Each example reinforces the basic principles while introducing more complex variations, helping students recognize patterns and develop problem-solving strategies for a wide range of logarithmic expressions.
Teacher's Script: Let's look at this example involving logarithms of square and cube roots. We need to simplify log(√(10)) + log(3√10). Remember, we can rewrite roots as fractional exponents: √(10) is 10(1/2) and 3√10 is 10(1/3). Now we can use the property log(na) = a * log(n). So, our expression becomes (1/2)log(10) + (1/3)log(10). We can factor out log(10): (1/2 + 1/3)log(10) = (5/6)log(10). This is equivalent to log(10(5/6)). The final result is 5/6. This example shows how we can simplify complex logarithmic expressions involving roots by using fractional exponents and logarithm properties.
For a complete collection of math examples related to Logarithms click on this link: Math Examples: Laws of Logarithms Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Laws of Logarithms |
| Copyright Year | 2013 |
| Keywords | logarithms, laws of logarithms |