Display Title
Math Example: Laws of Logarithms: Example 25
Display Title
Math Example: Laws of Logarithms: Example 25
Topic
Logarithms
Description
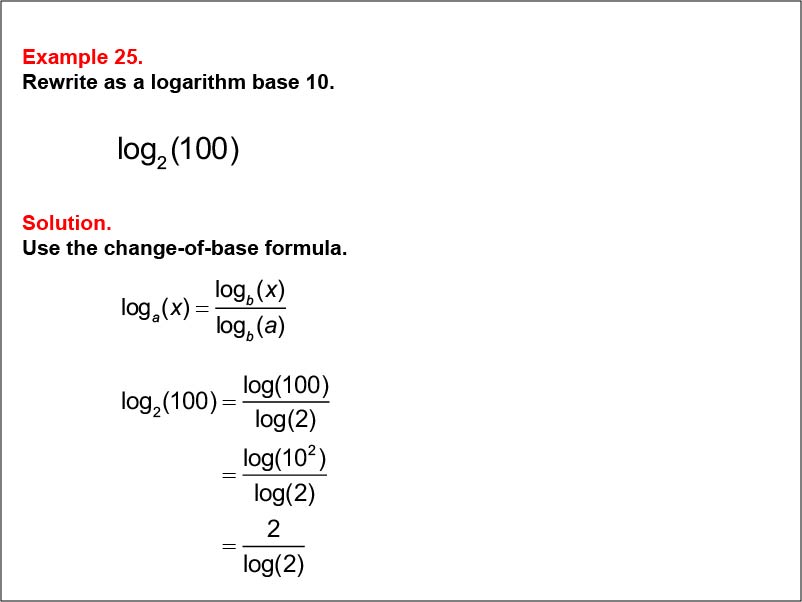
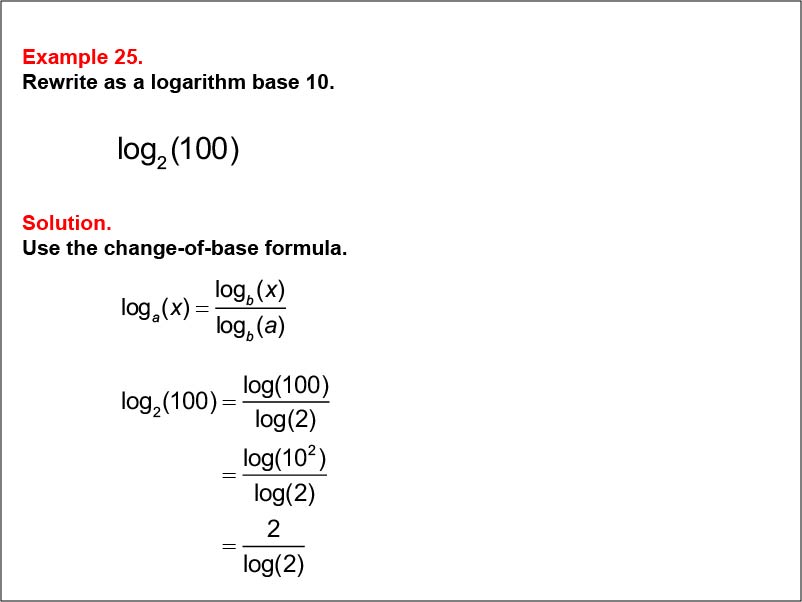
This example demonstrates the application of the change-of-base formula to convert a logarithm with base 2 to a logarithm with base 10. The problem involves rewriting log2(100) using the common logarithm (base 10). The solution applies the formula loga(x) = logb(x) / logb(a) to transform log2(100) into log(100) / log(2), which simplifies to 2 / log(2).
The change-of-base formula is a crucial concept in advanced algebra and precalculus. It allows us to express logarithms of any base in terms of logarithms of another base, typically base 10 or base e (natural logarithm). This is particularly useful when working with calculators or computers that may only have functions for common and natural logarithms.
Understanding and applying the change-of-base formula helps students develop a deeper comprehension of logarithmic properties and enhances their problem-solving skills in more complex logarithmic expressions. It bridges the gap between theoretical knowledge of logarithms and practical applications in scientific and engineering calculations.
Teacher's Script: Let's look at this example where we need to rewrite a logarithm with base 2 as a logarithm with base 10. We start with log2(100). To use the change-of-base formula, we write log2(100) = log(100) / log(2). Now, log(100) is the same as log(102), which equals 2. So our expression becomes 2 / log(2). This is as far as we can simplify without a calculator. Remember, this formula allows us to express any logarithm in terms of common logarithms, which is very useful when using calculators or solving more complex problems.
For a complete collection of math examples related to Logarithms click on this link: Math Examples: Laws of Logarithms Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Laws of Logarithms |
| Copyright Year | 2013 |
| Keywords | logarithms, laws of logarithms |