Display Title
Math Example: Laws of Logarithms: Example 27
Display Title
Math Example: Laws of Logarithms: Example 27
Topic
Logarithms
Description
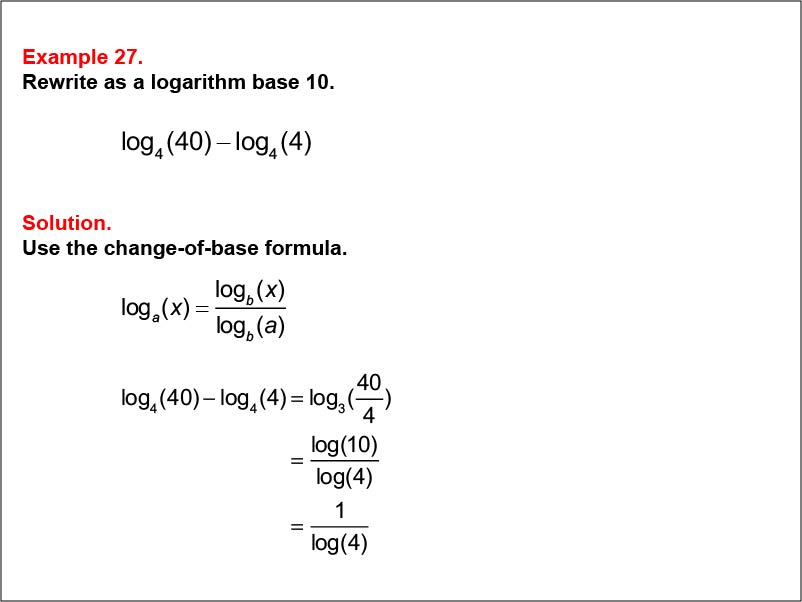
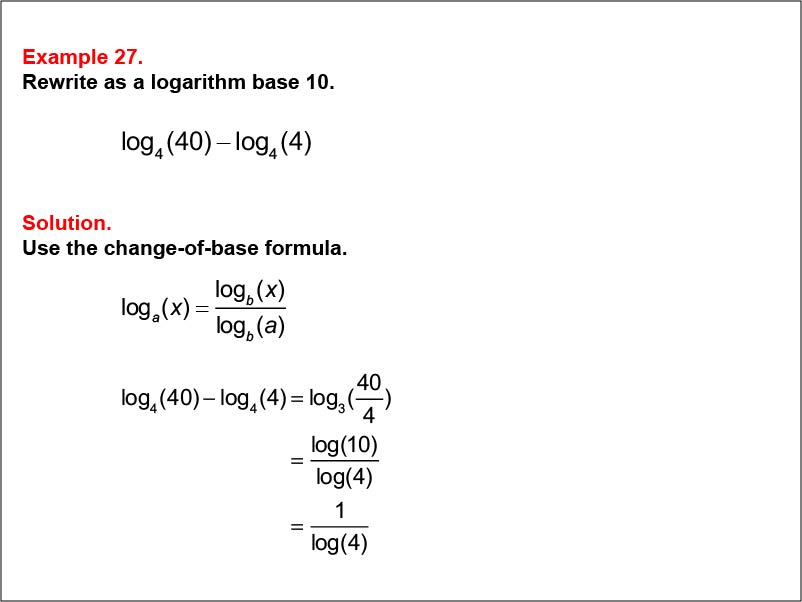
This example demonstrates the application of the change-of-base formula to convert the difference of two logarithms with base 4 to a single logarithm with base 10. The problem involves rewriting log4(40) - log4(4) using the common logarithm (base 10). The solution first applies the quotient rule of logarithms to combine the terms into log4(40/4), then uses the change-of-base formula loga(x) = logb(x) / logb(a) to transform it into log(10) / log(4), which simplifies to 1 / log(4).
This example highlights the importance of understanding multiple logarithmic properties and how they can be applied in sequence. It combines the quotient rule of logarithms with the change-of-base formula, showcasing how these properties work together to simplify complex expressions. Such problems help students develop a more integrated understanding of logarithmic manipulation and enhance their problem-solving skills.
By working through examples like this, students learn to approach logarithmic problems systematically, applying rules in a logical sequence to achieve the desired form. This skill is crucial for success in advanced mathematics and many scientific and engineering applications.
Teacher's Script: Let's tackle this example where we have the difference of two logarithms with base 4, and we need to express it as a single logarithm with base 10. We start with log4(40) - log4(4). First, we can use the quotient rule of logarithms to combine these into a single logarithm: log4(40/4) = log4(10). Now, to change the base to 10, we apply the change-of-base formula: log4(10) = log(10) / log(4). We know that log(10) = 1, so our final expression is 1 / log(4). This example demonstrates how combining the quotient rule with the change-of-base formula can simplify complex logarithmic expressions efficiently.
For a complete collection of math examples related to Logarithms click on this link: Math Examples: Laws of Logarithms Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Laws of Logarithms |
| Copyright Year | 2013 |
| Keywords | logarithms, laws of logarithms |