Display Title
Math Example: Laws of Logarithms: Example 29
Display Title
Math Example: Laws of Logarithms: Example 29
Topic
Logarithms
Description
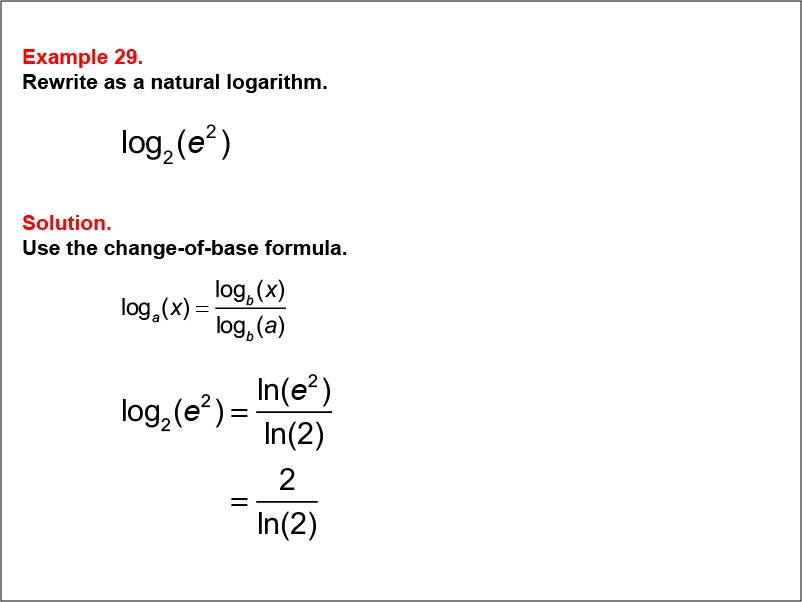
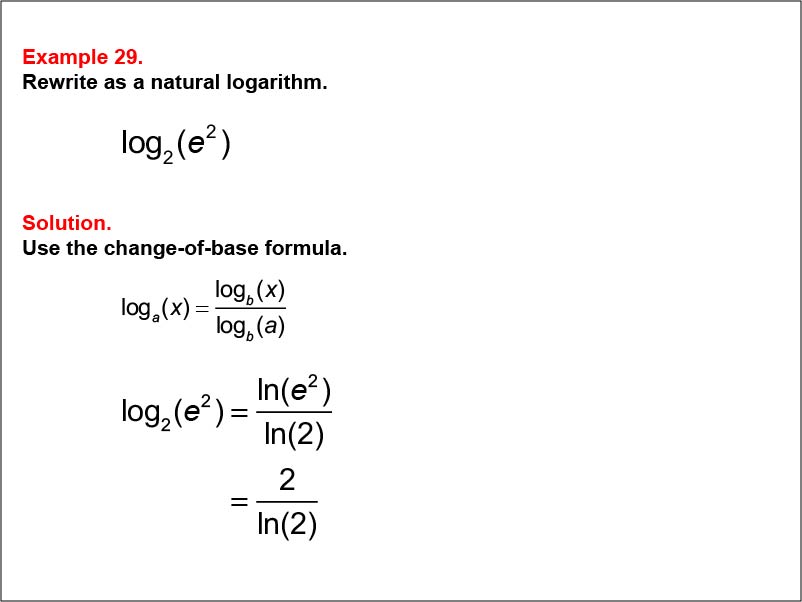
This example demonstrates the application of the change-of-base formula to convert a logarithm with base 2 to a natural logarithm (base e). The problem involves rewriting log2(e2) using the natural logarithm. The solution applies the formula loga(x) = logb(x) / logbb(a) to transform log2(e2) into ln(e2) / ln(2), which simplifies to 2 / ln(2).
This example highlights the relationship between logarithms of different bases, particularly the connection between base 2 logarithms and natural logarithms. It showcases how the change-of-base formula can be used to convert between these different types of logarithms. Understanding these relationships is crucial for students as they progress to more advanced mathematical concepts, especially in calculus and differential equations.
By working through examples like this, students develop a deeper appreciation for the versatility of logarithmic properties and their applications in various mathematical and scientific contexts. This knowledge is particularly valuable in fields such as computer science, where base 2 logarithms are common, and in natural sciences, where natural logarithms are frequently used.
Teacher's Script: Let's look at this interesting example where we need to rewrite a logarithm with base 2 as a natural logarithm. We start with log2(e2). To use the change-of-base formula, we write log2(e2) = ln(e2) / ln(2). Now, ln(e2) simplifies to just 2, because the natural log and e cancel each other out. So our expression becomes 2 / ln(2). This is as far as we can simplify without a calculator. This example shows how we can convert between different types of logarithms, which is a valuable skill in many areas of mathematics and science.
For a complete collection of math examples related to Logarithms click on this link: Math Examples: Laws of Logarithms Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Laws of Logarithms |
| Copyright Year | 2013 |
| Keywords | logarithms, laws of logarithms |