Display Title
Math Example: Laws of Logarithms: Example 30
Display Title
Math Example: Laws of Logarithms: Example 30
Topic
Logarithms
Description
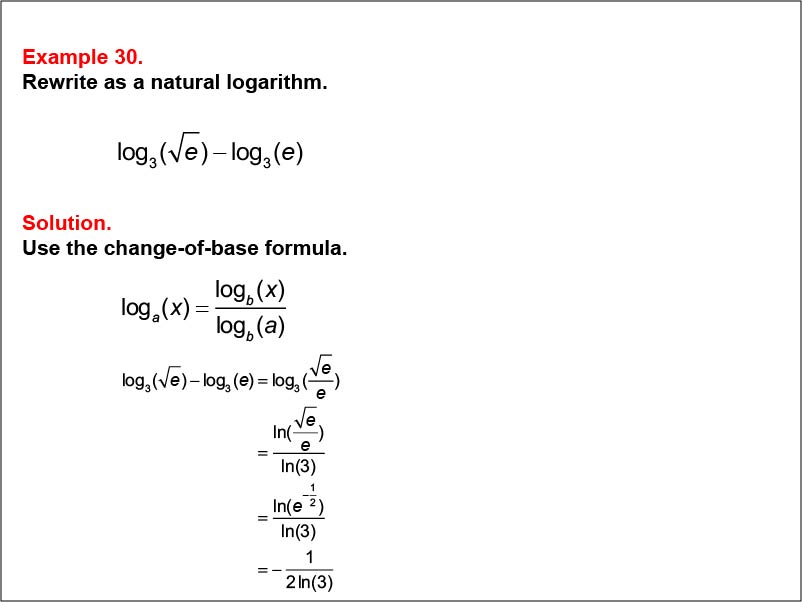
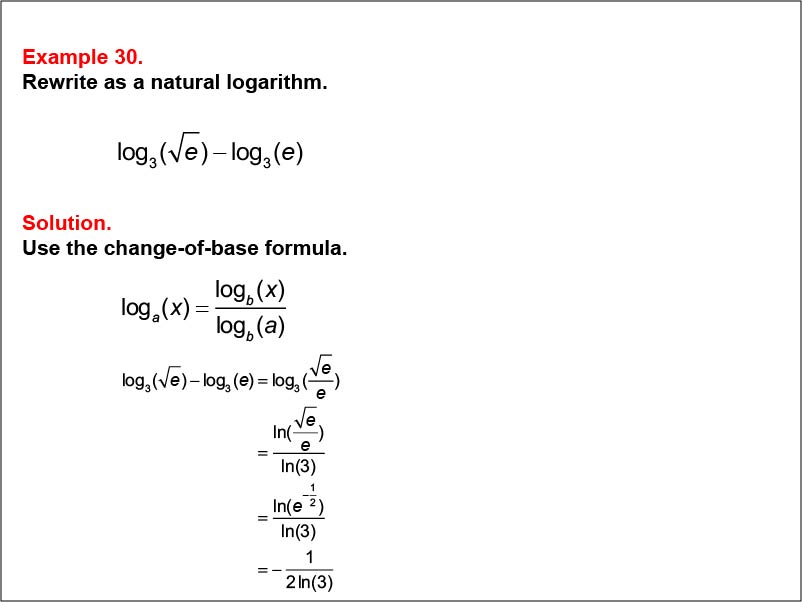
This example demonstrates the application of the change-of-base formula to convert the difference of two logarithms with base 3 to a natural logarithm expression. The problem involves rewriting log3(√(e)) - log3(e) using natural logarithms. The solution first applies the quotient rule of logarithms to combine the terms into log_3(√(e)/e), then uses the change-of-base formula loga(x) = ln(x) / ln(a) to transform it into ln(√(e)/e) / ln(3), which simplifies to -1 / (2 ln(3)).
This example showcases the interplay between various logarithmic properties, including the quotient rule, properties of square roots, and the change-of-base formula with natural logarithms. It demonstrates how these properties can be combined to simplify and transform complex logarithmic expressions involving the mathematical constant e. Such problems help students develop a more comprehensive understanding of logarithmic manipulation and enhance their problem-solving skills with advanced mathematical concepts.
By working through examples like this, students learn to approach logarithmic problems strategically, choosing the most appropriate properties and techniques for each situation, even when dealing with expressions involving irrational numbers and mathematical constants. This flexibility in applying logarithmic rules is essential for success in advanced mathematics, particularly in calculus and differential equations.
Teacher's Script: Let's tackle this example involving logarithms with base 3 and the constant e. We start with log3(√(e)) - log3(e). First, we can use the quotient rule of logarithms to combine these into a single logarithm: log3(√(e)/e). Now, √(e)/e = e(-1/2). To change the base to e (natural log), we apply the change-of-base formula: log_3(e(-1/2)) = ln(e(-1/2)) / ln(3) = -1/2 / ln(3) = -1 / (2 ln(3)). This example shows how we can use multiple logarithm properties along with the change-of-base formula to simplify complex expressions involving e and different logarithm bases.
For a complete collection of math examples related to Logarithms click on this link: Math Examples: Laws of Logarithms Collection.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.BF.B.5 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Laws of Logarithms |
| Copyright Year | 2013 |
| Keywords | logarithms, laws of logarithms |