
Display Title
Math Example--Linear Function Concepts--The Point-Slope Formula: Example 1
Display Title
Math Example--Linear Function Concepts--The Point-Slope Formula: Example 1
Topic
The Point-Slope Form
Description
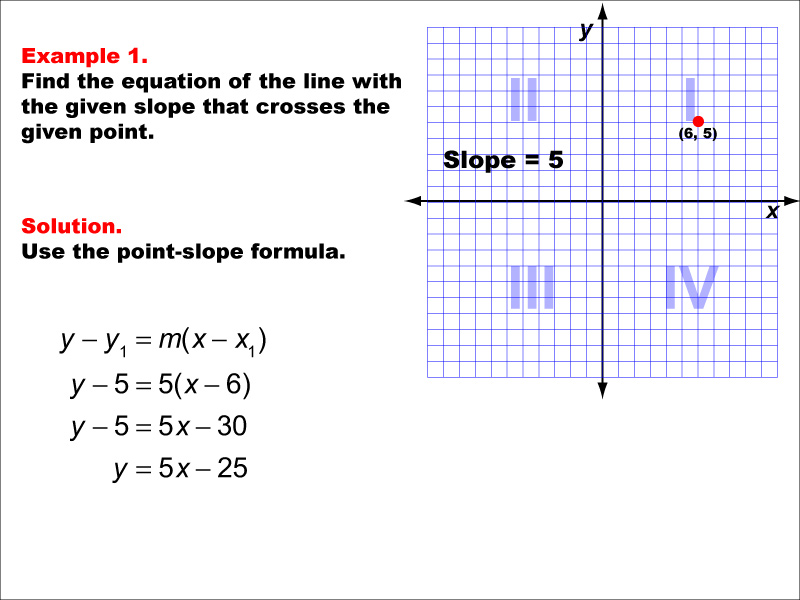
This example demonstrates how to find the equation of a line using the point-slope form. The given information includes a slope of 5 and a point (6, 5) through which the line passes. Using the point-slope formula y - y1 = m(x - x1), we can substitute the known values to derive the equation y - 5 = 5(x - 6). Simplifying this equation leads to the final result: y = 5x - 25.
The point-slope form is a powerful tool in linear algebra, allowing us to quickly determine the equation of a line when given a point on the line and its slope. This collection of examples helps teach this topic by providing various scenarios with different slopes and points, demonstrating the versatility of the formula.
Presenting multiple worked-out examples is crucial for students to fully grasp the concept. Each example reinforces the application of the point-slope formula while showcasing different numerical values and graph positions. This repetition helps students recognize patterns and build confidence in their problem-solving skills.
Teacher's Script: Now, let's look at our first example. We're given a line with a slope of 5 that passes through the point (6, 5). Remember, the point-slope form is y - y1 = m(x - x1), where m is the slope, and (x1, y1) is a point on the line. Can you help me plug in the values we know? Great! Now, let's simplify the equation step by step to get our final answer.
For a complete collection of math examples related to the Point-Slope Form click on this link: Math Examples: Point-Slope Form Collection.
| Common Core Standards | CCSS.MATH.CONTENT.8.EE.B.6, CCSS.MATH.CONTENT.8.EE.B.5 |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Linear Functions and Equations • Point-Slope Form |
| Copyright Year | 2013 |
| Keywords | point-slope form, slope-intercept form, linear equations |