Display Title
Math Example--Math of Money--Compound Interest: Example 2
Display Title
Math Example--Math of Money--Compound Interest: Example 2
Topic
Math of Money
Description
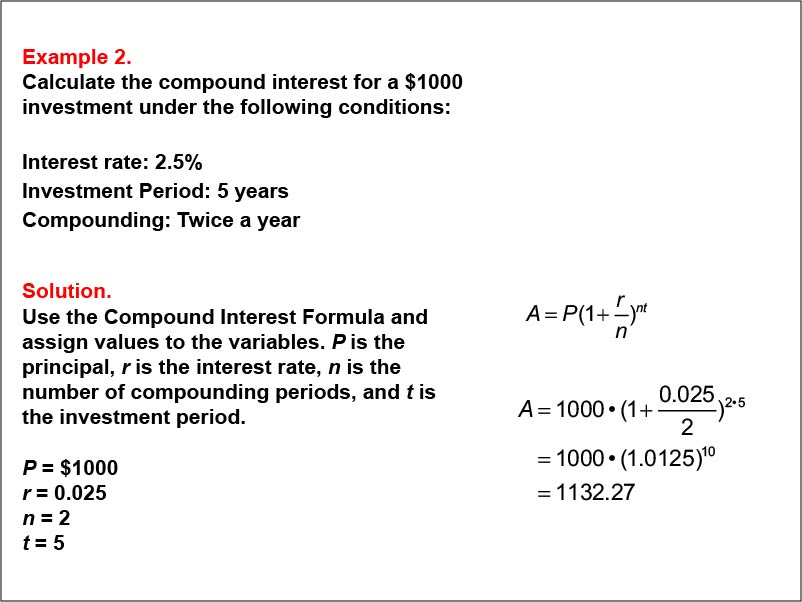
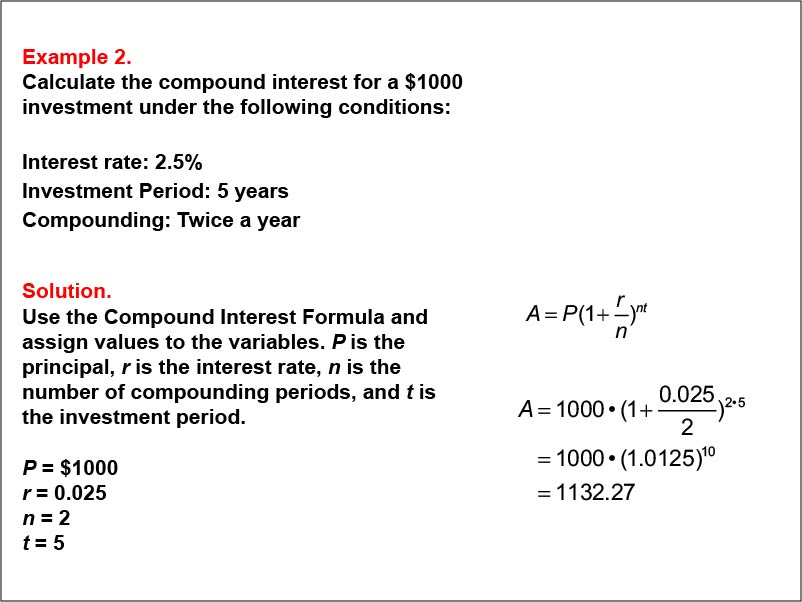
This example illustrates the calculation of compound interest for a $1000 investment at a 2.5% interest rate over 5 years, compounded semi-annually. The formula A = P(1 + r/n)nt is applied with P = 1000, r = 0.025, n = 2, and t = 5. The final amount after 5 years is $1132.27.
Compound interest is a powerful concept in finance that shows how investments can grow exponentially over time. By presenting examples with different compounding frequencies, students can observe how more frequent compounding leads to slightly higher returns. This collection of examples helps to solidify understanding of the compound interest formula and its applications in various financial scenarios.
Examining multiple worked-out examples is essential for students to fully comprehend compound interest. It allows them to compare results, identify patterns, and understand the impact of changing variables such as compounding frequency. This approach enhances their analytical skills and prepares them for more complex financial calculations in real-world situations.
Teacher Script: "Now, let's see what happens when we compound the interest twice a year instead of annually. We'll use the same $1000 investment at 2.5% for 5 years, but now we'll compound every 6 months. Watch how this small change affects the final amount."
For a complete collection of math examples related to Compound Interest click on this link: Math Examples: Compound Interest Collection.
| Common Core Standards | CCSS.MATH.CONTENT.7.RP.A.3, CCSS.MATH.CONTENT.HSF.LE.A.1, CCSS.MATH.CONTENT.HSF.IF.C.8.B |
|---|---|
| Grade Range | 8 - 12 |
| Curriculum Nodes |
Algebra • Exponential and Logarithmic Functions • Compound Interest |
| Copyright Year | 2013 |
| Keywords | interest, compound interest, math of money |