Display Title
Math Example--Measures of Central Tendency--Mean: Example 18
Display Title
Math Example--Measures of Central Tendency--Mean: Example 18
Topic
Measures of Central Tendency
Description
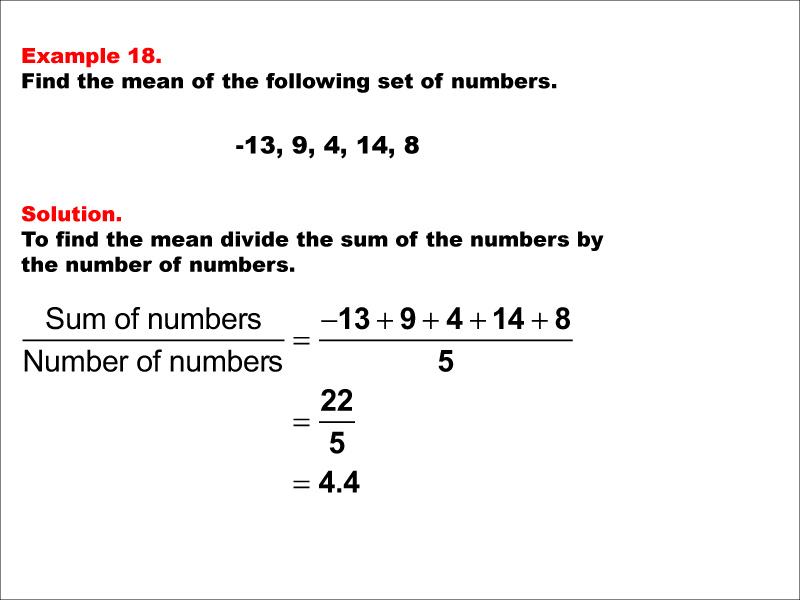
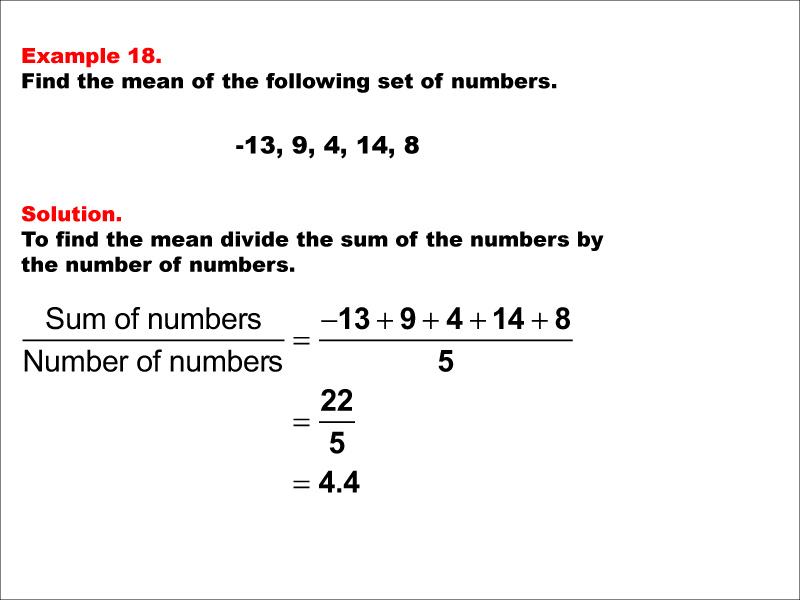
This example demonstrates the calculation of the mean for the numbers -13, 9, 4, 14, and 8. The process involves summing all values (22) and dividing by the count of numbers (5), resulting in a mean of 4.4. This example is particularly instructive as it shows how a single negative number can significantly impact the mean, even when most of the numbers are positive.
Understanding measures of central tendency, particularly the mean, is crucial in statistics and data analysis. These measures provide a way to summarize large amounts of data into a single, representative value, allowing for quick comparisons and insights. Mastering these concepts, especially when dealing with datasets that include outliers or extreme values, enables students to interpret data effectively and make informed decisions in various fields of study.
The presentation of multiple examples, especially those with a mix of positive and negative numbers, is key to solidifying students' grasp of the mean concept. Each new example, while following the same basic process, presents a unique set of numbers with different characteristics. This repetition with variation helps students recognize the underlying principles and develop the flexibility to apply the concept across diverse situations, including those where the mean might be influenced by extreme values.
Teacher Script: "Let's examine our eighteenth example of calculating the mean. Notice how our dataset includes mostly positive numbers, but with one significant negative value. As we work through this, consider how this single negative number affects our final result. The mean of 4.4 is positive, but it's lower than most of the individual positive values in our set. This example illustrates an important point: even a single extreme value can have a noticeable impact on the mean. This underscores the importance of looking at the entire dataset, not just the mean, when analyzing data."
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Mean Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.6.SP.A.3, CCSS.MATH.CONTENT.HSS.ID.A.2, CCSS.MATH.CONTENT.HSS.ID.A.3 |
|---|---|
| Grade Range | 6 - 12 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2014 |
| Keywords | data analysis, tutorials, measures of central tendency, mean, average |