Display Title
Math Example--Measures of Central Tendency--Mean: Example 34
Display Title
Math Example--Measures of Central Tendency--Mean: Example 34

Topic
Measures of Central Tendency
Description
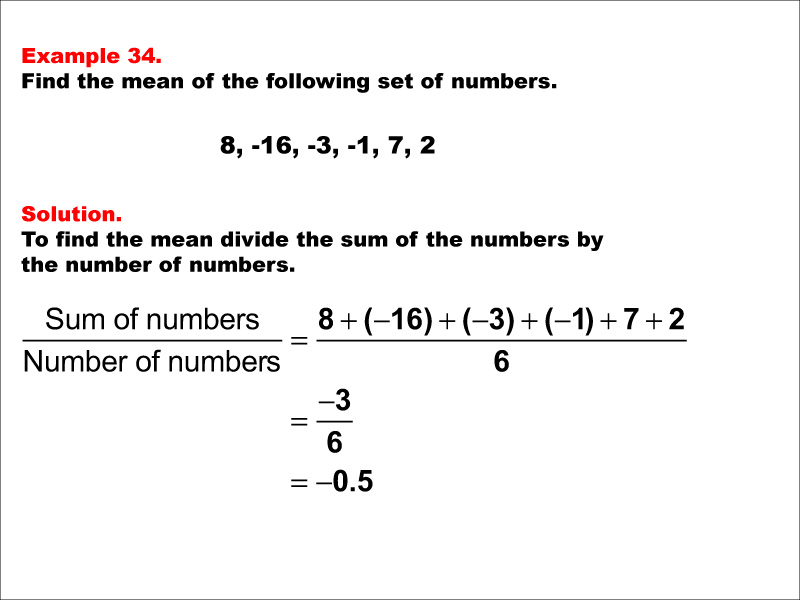
This example demonstrates the calculation of the mean for yet another set of numbers. The process involves summing all values and dividing by the count of numbers. This example is particularly instructive as it continues to reinforce the concept of finding a central value that represents the entire dataset, potentially dealing with a different range or distribution of numbers than previous examples.
Understanding measures of central tendency, particularly the mean, is crucial in statistics and data analysis. These measures provide a way to summarize large amounts of data into a single, representative value, allowing for quick comparisons and insights. Mastering these concepts enables students to interpret data effectively and make informed decisions in various fields of study.
The presentation of multiple examples is key to solidifying students' grasp of the mean concept. Each new example, while following the same basic process, presents a unique set of numbers with different characteristics. This repetition with variation helps students recognize the underlying principles and develop the flexibility to apply the concept across diverse situations, including those where the mean might be influenced by extreme values or unusual distributions.
Teacher Script: "Let's examine our thirty-fourth example of calculating the mean. As we work through this, consider how this dataset might be similar to or different from the ones we've seen before. How does the mean we calculate relate to the individual values in our set? Remember, the mean provides a balance between all values in a dataset. This example illustrates an important point: the mean takes into account all values in a dataset, balancing out extremes. In real-world scenarios, this property of the mean can be both a strength and a weakness, depending on the context. It's crucial to always consider the entire dataset alongside the mean when interpreting data."
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Mean Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.6.SP.A.3, CCSS.MATH.CONTENT.HSS.ID.A.2, CCSS.MATH.CONTENT.HSS.ID.A.3 |
|---|---|
| Grade Range | 6 - 12 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2014 |
| Keywords | data analysis, tutorials, measures of central tendency, mean, average |