Display Title
Math Example--Measures of Central Tendency--Mean: Example 6
Display Title
Math Example--Measures of Central Tendency--Mean: Example 6

Topic
Measures of Central Tendency
Description
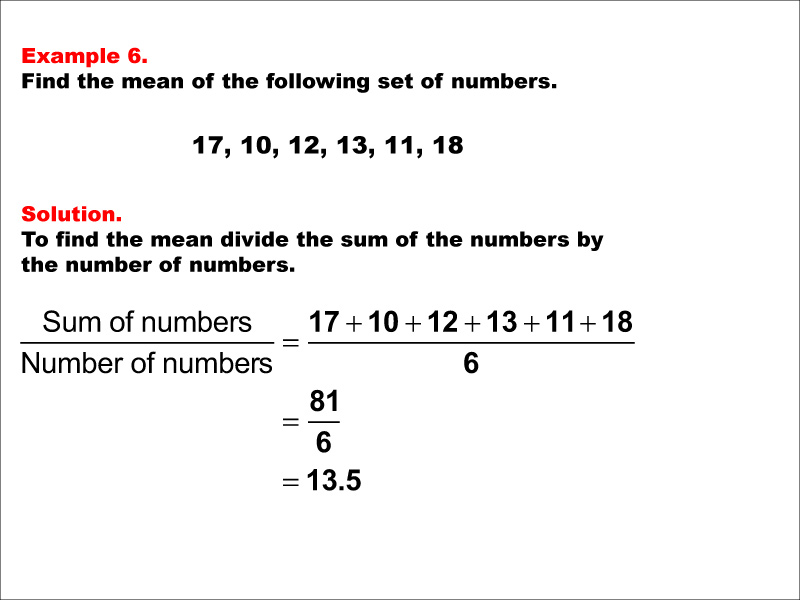
This sixth example in the series on calculating the mean introduces a new set of numbers, further solidifying the concept and its application. It reiterates the consistent process of finding the mean: summing all values and dividing by the count of numbers, regardless of the specific values in the dataset. The visual representation continues to support understanding of each step in the calculation.
Measures of central tendency, including the mean, are fundamental tools in statistics that allow us to summarize and interpret large sets of data. By reducing a dataset to a single, representative value, these measures enable comparisons between different datasets and provide insights into overall trends. Mastering these concepts is essential for students as they progress to more advanced statistical analyses and data interpretation tasks in various academic and professional fields.
Providing multiple examples is crucial for deepening students' understanding of the mean. Each new example reinforces the calculation process while introducing slight variations in the data, helping students recognize that the concept applies universally, regardless of the specific numbers involved. This approach builds confidence and prepares students to apply the concept flexibly in various real-world contexts, from analyzing scientific data to interpreting economic trends.
Teacher Script: "We're now on our sixth example of calculating the mean. As we work through this new set of numbers, I want you to think about how confident you're becoming with this process. Can you predict whether the mean will be higher or lower than in our previous example before we calculate it? Think about how the mean might be used in real-world situations. For instance, how might a company use the mean salary of its employees to make decisions? Or how might a farmer use the mean rainfall over several years to plan crop rotations? Remember, understanding the mean isn't just about numbers - it's about gaining insights that can inform important decisions."
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Mean Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.6.SP.A.3, CCSS.MATH.CONTENT.HSS.ID.A.2, CCSS.MATH.CONTENT.HSS.ID.A.3 |
|---|---|
| Grade Range | 6 - 12 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2014 |
| Keywords | data analysis, tutorials, measures of central tendency, mean, average |