Display Title
Math Example--Measures of Central Tendency--Mean: Example 7
Display Title
Math Example--Measures of Central Tendency--Mean: Example 7

Topic
Measures of Central Tendency
Description
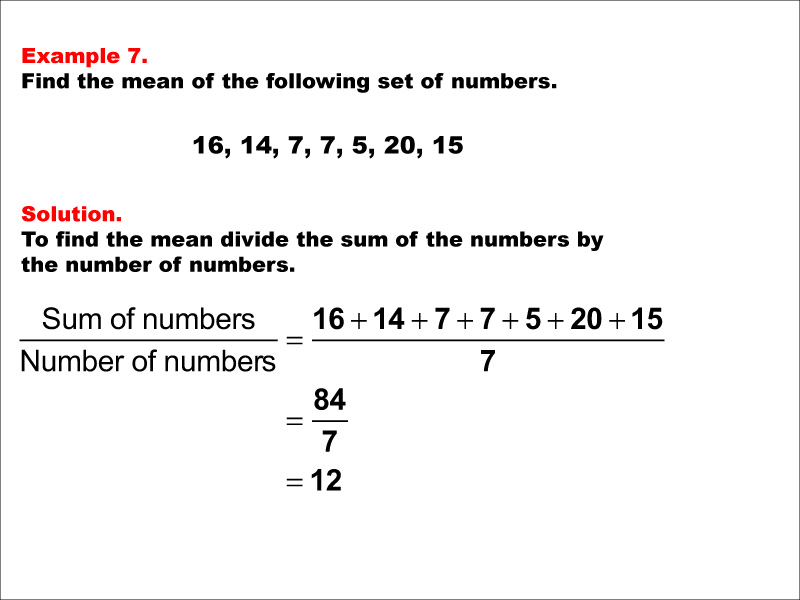
This seventh example in the series on calculating the mean presents yet another set of numbers, further reinforcing the concept and its application in various scenarios. It continues to demonstrate the consistent process of finding the mean: summing all values and dividing by the count of numbers, regardless of the specific values or the size of the dataset. The visual representation aids in understanding each step of the calculation process.
Understanding measures of central tendency, particularly the mean, is crucial in statistics and data analysis. These measures provide a way to summarize large amounts of data into a single, representative value, allowing for quick comparisons and insights. Mastering these concepts enables students to interpret data effectively, make informed decisions, and prepare for more advanced statistical analyses in various fields of study, from social sciences to natural sciences and beyond.
The presentation of multiple examples is key to solidifying students' grasp of the mean concept. Each new example, while following the same basic process, presents a unique set of numbers. This repetition with variation helps students recognize the underlying principles and develop the flexibility to apply the concept across diverse situations. It also builds confidence in their ability to handle different types of datasets they might encounter in academic research or real-world problem-solving scenarios.
Teacher Script: "We've reached our seventh example of calculating the mean. As we work through this new set of numbers, I want you to reflect on how your understanding of the concept has grown. Can you explain why we might choose to use the mean instead of other measures of central tendency in certain situations? Think about how you might use the mean in your own life. For instance, how could knowing the mean help you budget your allowance or plan for a group project? Remember, the power of the mean lies not just in the calculation, but in how we can use it to make informed decisions and understand the world around us."
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Mean Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.6.SP.A.3, CCSS.MATH.CONTENT.HSS.ID.A.2, CCSS.MATH.CONTENT.HSS.ID.A.3 |
|---|---|
| Grade Range | 6 - 12 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2014 |
| Keywords | data analysis, tutorials, measures of central tendency, mean, average |