Display Title
Math Example--Measures of Central Tendency--Median: Example 1
Display Title
Math Example--Measures of Central Tendency--Median: Example 1
Topic
Measures of Central Tendency
Description
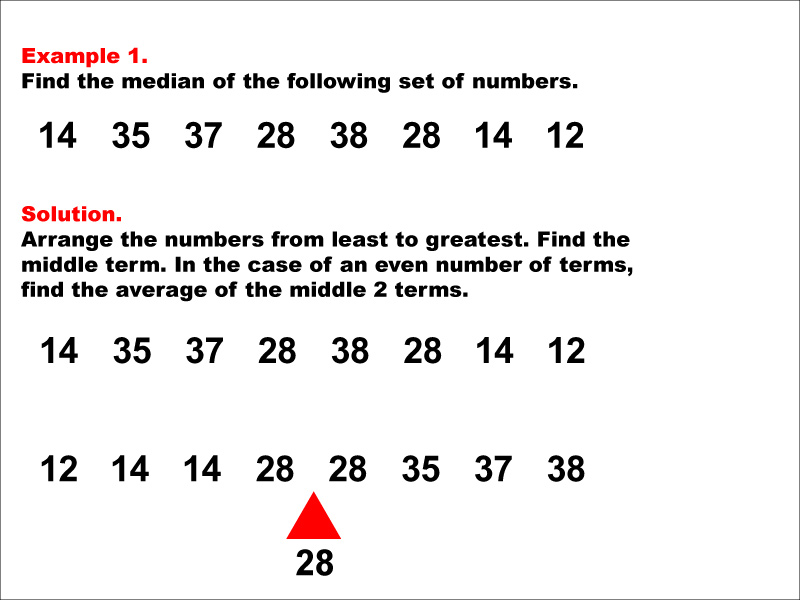
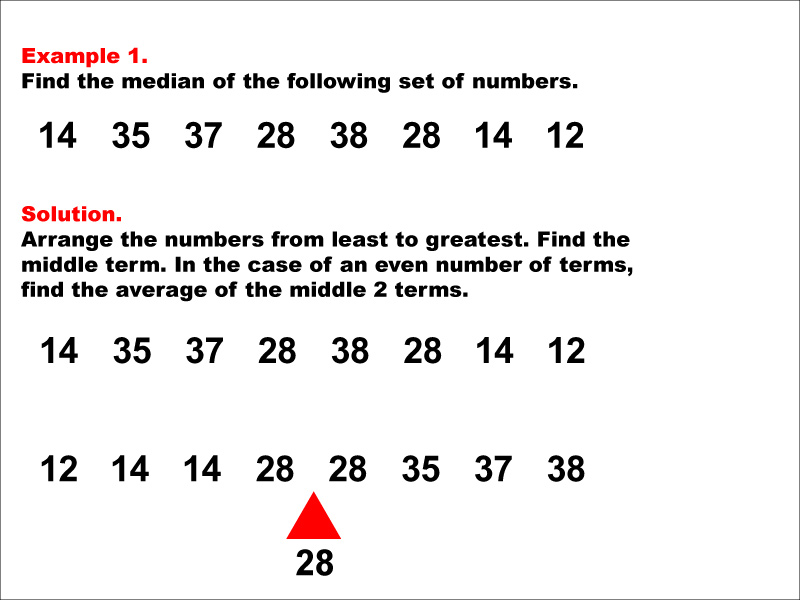
This example demonstrates how to find the median of a set of numbers: 14, 35, 37, 28, 38, 28, 14, 12. The process involves arranging the numbers in ascending order and identifying the middle value. In this case, with an even number of terms, the median is calculated by finding the average of the two middle terms, resulting in a median of 28.
The concept of median is a crucial measure of central tendency in statistics. It represents the middle value in a sorted dataset, providing a robust measure that is less affected by extreme values compared to the mean. These examples help teach this topic by illustrating the step-by-step process of finding the median for various datasets, including those with even and odd numbers of values.
Presenting multiple worked-out examples is essential for students to fully grasp the concept of median. By seeing different scenarios, such as datasets with even or odd numbers of values, positive and negative numbers, or repeated values, students can develop a comprehensive understanding of how to calculate the median in various situations. This approach reinforces the procedure and helps students recognize patterns and nuances in median calculation.
Teacher Script: "Class, let's look at this example of finding the median. Remember, the median is the middle number when our data is in order. First, we'll arrange these numbers from least to greatest. Then, since we have an even number of terms, we'll find the average of the two middle numbers. This process helps us find the typical or central value in our dataset, which is useful for understanding the overall distribution of our data."
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Median Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.6.SP.A.3, CCSS.MATH.CONTENT.HSS.ID.A.2, CCSS.MATH.CONTENT.HSS.ID.A.3 |
|---|---|
| Grade Range | 6 - 12 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2014 |
| Keywords | data analysis, tutorials, measures of central tendency, median, average |