Display Title
Math Example--Measures of Central Tendency--Mode: Example 35
Display Title
Math Example--Measures of Central Tendency--Mode: Example 35
Topic
Measures of Central Tendency
Description
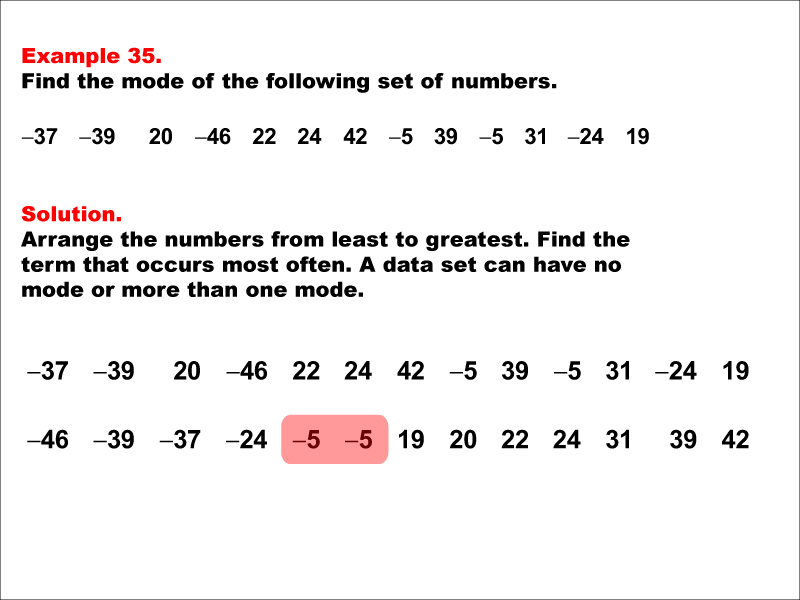
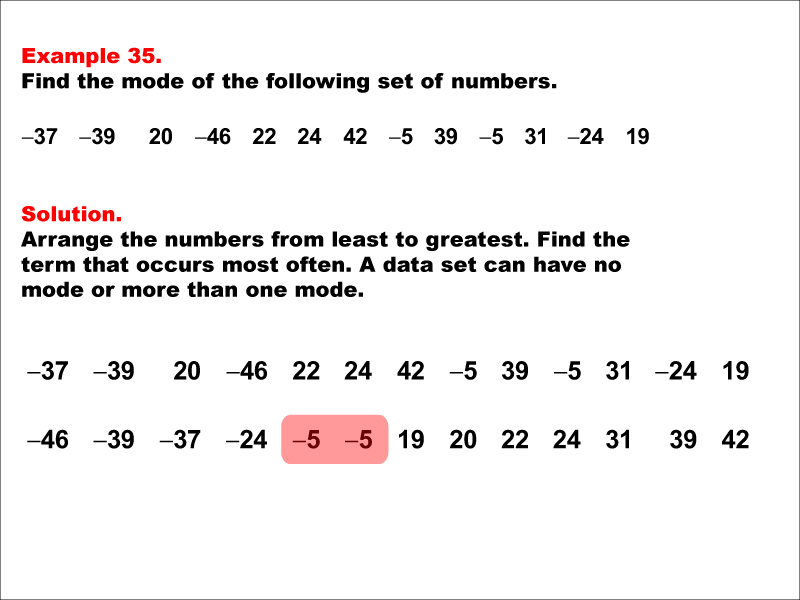
This example showcases a situation of measures of central tendency, where the goal is to identify a key summary measure in a set of data. The image displays a math example where a set of numbers is provided to find the mode. The numbers are sorted and the mode is highlighted in red as it appears multiple times in the list. This example demonstrates how to identify the mode when a number appears more frequently than others in a data set that includes both positive and negative numbers.
Measures of Central Tendency lessons are instrumental in providing students with a better understanding of how to interpret data through these examples. Each example highlights distinct scenarios which reinforce the concept of determining frequency of occurrences within given sets, enhancing students' analytical skills.
Seeing multiple worked-out examples is crucial in solidifying a student's grasp on a concept. Each example contributes unique perspectives and challenges that can arise when thinking about data sets. This varied approach not only caters to diverse learning styles but also ensures that all students can see the relevance of these concepts in their learning journey.
Teacher's Script
Now, let's look at this interesting example. We have a mix of positive and negative numbers: -37, -39, 20, -46, 22, 24, 42, -5, 39, -5, 31, -24, and 19. Our job is to find the mode. Remember, the mode is the value that appears most frequently in our data set. As we arrange these numbers from least to greatest, pay attention to how many times each number appears. Do you notice any number showing up more than once? That's right, the number -5 appears twice, while all other numbers appear only once. This makes -5 our mode. This example teaches us an important lesson: the mode can be found in data sets with both positive and negative numbers. In real-world scenarios, this could represent situations where we're dealing with both gains and losses, like in financial data or temperature changes.
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Mode Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.6.SP.A.3, CCSS.MATH.CONTENT.HSS.ID.A.2, CCSS.MATH.CONTENT.HSS.ID.A.3 |
|---|---|
| Grade Range | 6 - 12 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2014 |
| Keywords | data analysis, tutorials, measures of central tendency, mode, average |