
Display Title
Math Example--Measures of Central Tendency--Mode: Example 70
Display Title
Math Example--Measures of Central Tendency--Mode: Example 70
Topic
Measures of Central Tendency
Description
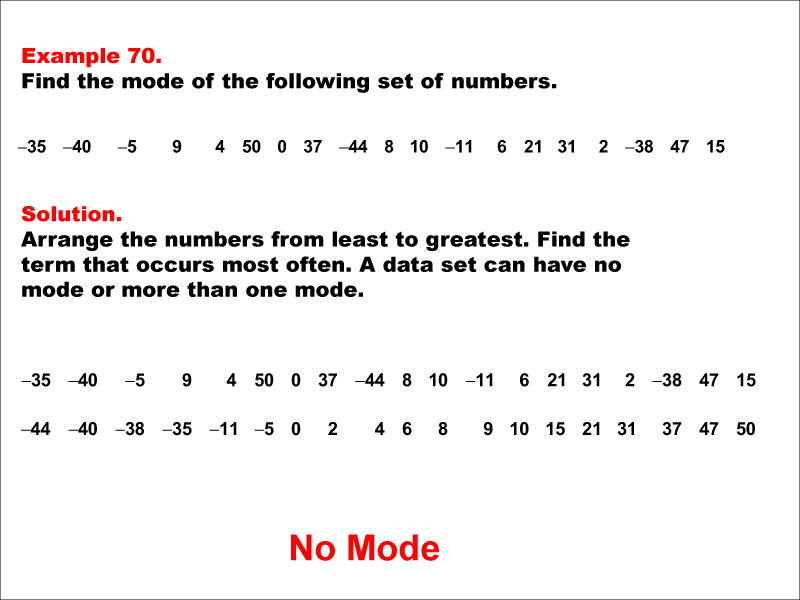
This example illustrates how no modes exist within certain datasets due to lack of repetition among individual entries, resulting in a uniform distribution across the entire range of values present. This makes it impossible to determine a single most frequent occurrence, thereby highlighting the importance of considering alternative statistical methods such as mean and median instead, in order to gain deeper insights into underlying trends and patterns observable therein. Ultimately, this aids decision-making processes in an informed manner given available information and contextually relevant circumstances encountered along the journey of discovery, knowledge acquisition, exploration, understanding, comprehension, and application thereof in practical settings and everyday life situations encountered daily in work and personal lives alike!
Teacher's Script
This example shows us that not every dataset will have a mode. In real-world data, this could indicate a wide variety of values with no clear 'most common' value. For instance, if these numbers represented different measurements or observations, having no mode would suggest a diverse set with no particular value being more common than others.
For a complete collection of math examples related to Measures of Central Tendency click on this link: Math Examples: Measures of Central Tendency: Mode Collection.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.6.SP.A.3, CCSS.MATH.CONTENT.HSS.ID.A.2, CCSS.MATH.CONTENT.HSS.ID.A.3 |
|---|---|
| Grade Range | 6 - 12 |
| Curriculum Nodes |
Algebra • Probability and Data Analysis • Data Analysis |
| Copyright Year | 2014 |
| Keywords | data analysis, tutorials, measures of central tendency, mode, average |